The story of a mercilessly thrown sphere...
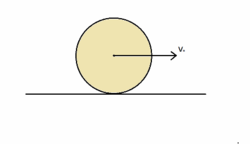 A spherical ball of mass
M
and radius
R
is thrown along a rough horizontal surface so that
initially
, it slides with a linear speed
υ
0
,but
does not rotate
.
As it slides, it begins to spin and eventually rolls without slipping. The time taken to start rolling can be expressed as
b
a
×
μ
k
g
υ
0
Where
μ
k
is the coefficient of kinetic friction between the surface and the ball .What is
a
+
b
?
A spherical ball of mass
M
and radius
R
is thrown along a rough horizontal surface so that
initially
, it slides with a linear speed
υ
0
,but
does not rotate
.
As it slides, it begins to spin and eventually rolls without slipping. The time taken to start rolling can be expressed as
b
a
×
μ
k
g
υ
0
Where
μ
k
is the coefficient of kinetic friction between the surface and the ball .What is
a
+
b
?
Liked it? try some more
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
one can also used angular momentum conservation about the bottom most point . To get final velocity , m v o R = m v ′ R + 5 2 m R 2 ( R v ′ ) v = 7 5 v o .
Log in to reply
I never thought of that..! Brilliant..!
This is exactly what I did!!!
Exactly what I did....
ONE SHOULD MENTION THAT THE BALL IS SOLID NOT A SHELL AS I DID THE SAME METHOD BUT I TOOK MOMENT OF INERTIA OF SHELL
Shouldn’t the torque be negative?
Log in to reply
We can take it to be positive without changing the math since w_0 is zero.
I think we should multiply by R on the RHS in the final equality.
Conserving angular momentum about any point in the horizontal plane, we get
m v 0 R = m v R + 5 2 m R 2 ( R v ) 2 ⇒ v = 7 5 v 0
and
7 5 v 0 = v 0 − μ g t ⇒ t = 9 μ g 2 v 0
OR
we can solve for t from the following equations
μ m g = m a
μ m g R = 5 2 m R 2 α
v 0 − a t = α
This gives the same answer for t
Please be so kind to mention the next time whether the ball is hollow or filled it was certainly a bit confusing
you should give in question that the ball is solid sphere not hollow
FIRST BY USING CONSERVATION OF ANGULAR MOMENTUM ABUT POINT OF CONTACT FIND THE VELOCITY OF CENTER OF SPHERE time is 2/7 times yhe given exp
Taking the center of the sphere at the origin ,equation of motion of the sphere are: ∑ F x = M a x = − f = μ k M g … … … … ( 1 ) ∑ F y = N − M g = 0 … … … … ( 2 ) From the equations (1) & (2) we get a = − μ k g … … … . . ( 3 ) The velocity of the centre of mass at time t is υ = υ 0 + a x t . or υ = υ 0 − μ k g t . . . . . . . . . . . . . . . . ( 4 ) Equation of motion for rotation is ∑ τ = μ k M g R = ( 5 2 M R 2 ) α . . . . . . . . . . . . . . . . . ( 5 ) or α = 2 5 × R μ k g . . . . . . . . . . . . . . ( 6 ) Then the angular velocity at time t is ω = ω 0 + α t = 2 5 × R μ k g t . . . . . . . . . . . . . . . . . . . ( 7 ) condition for pure rolling is υ = ω R Now by using (4) & (7) we get υ 0 − μ k g t = 2 5 × R μ k g t or t = 7 2 × μ k g υ 0