The Ten Points

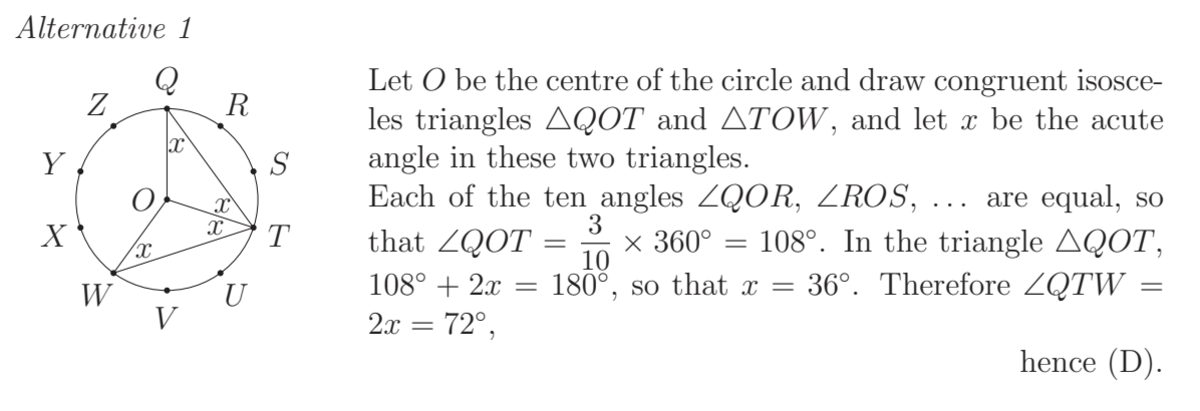
If the circle has ten points, that means that each angle will be 36 degrees because:

$\large{\theta=}$ $\large{\dfrac{360 ^\circ}{\text{\# interior angles}} = \dfrac{360^\circ}{10} = 36 ^\circ}$

The following formula is true for inscribed angles of this type:

$\boxed{\text{m} \angle QTW = \dfrac{1}{2} \text{ m} \angle QCW}$

We don't know $\angle QTW$ , but we do know that each angle slice within the circle is $36 ^\circ$ . Since there are 4 of these slices within $\angle QCW$ , we can see that:

$\angle QCW = 36 ^\circ (4) = 144 ^\circ$

Now we can plug $144 ^\circ$ into the formula above to solve for $\text{m} \angle QTW$ :

$\large{\text{m} \angle QTW = \dfrac{1}{2} \cdot 144 ^\circ = 72 ^\circ}$

So, the solution is $\boxed{\large{72^\circ}}$