The two trains...... at what speed ?

Algebra Level 2

Imagine two trains naming Water and Fire..

Water starts from station A to station B at 45 mph and fire starts from station B to station A at x mph at same time.

After meeting at a place between stations A and B Water reaches to station B after 4 hr 48 min. and fire reaches to station A after 3 hr 20 min. after meeting.

then what is the speed of Fire ?

60 54 36 45

2 solutions

Rohan Kumar
Mar 8, 2014

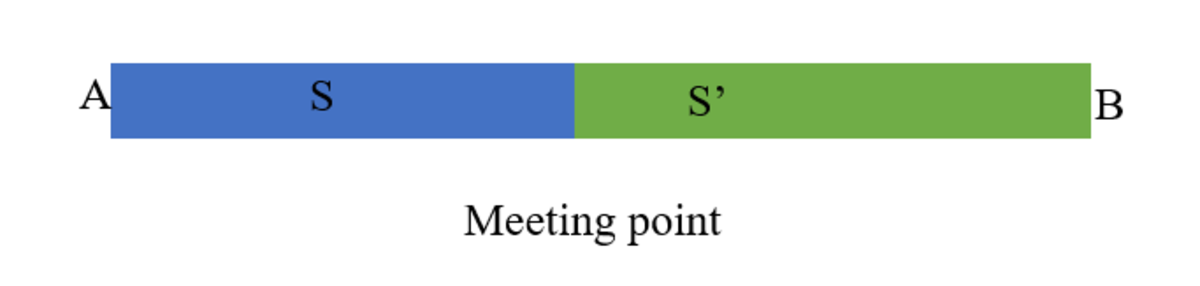
let distance be D and time when they meet be T and speed of fire be x mph

therefore equation will be :-

1) (45 + x) T = D

2) (T + 10/3)x = D

3) (T + 24/5)45 = D

by solving these equations we will get x=54

i dont uderstand the equations plzzz explain

Adnan Malik - 7 years, 3 months ago

x will be the speed of fire and T is the time when they meet and they are going in opposite direction therefore total speed will be (45 + x) and time is T therefore distance = speed *time , by this we got first equation

and second one and third one are also on the basis of speed time and distance formula

I think after doing this you will get your answers ...........

Rohan Kumar - 7 years, 3 months ago

Tuấn Huy Nguyễn
Mar 16, 2020

The meeting point divides the road AB into 2 parts. The lengths of these parts are proportional to the velocity of each car, it means that the ratio between the lengths is the same as the ratio between velocities. After that, the time is disproportional to the velocity. Hence, the time required is disproportional to the SQUARE of the two velocities. The ratio between times is 36/25 which implies that the ratio between velocities is 6/5. Hence the velocity of Fire is 6/5*45=54

The two trains...... at what speed ?

2 solutions

0 pending reports