The Weight Obsessed Islanders

With perfect information, ie whether or not the islander with a different weight is heavier or lighter than the rest, we cannot do it in two uses of the seesaw (try it yourself) so the answer is greater than two.

Here are the 12 islanders, who will each be assigned a letter at some point throughout the procedure.

$A,B,C,D,E,F,G,H,I,J,K,L$

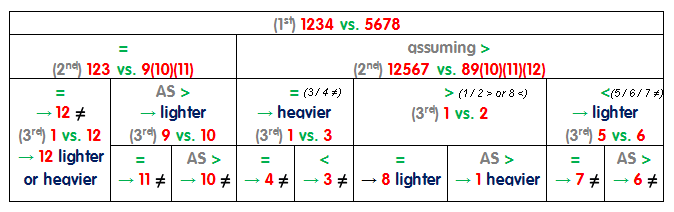
First Weighing

$A,B,C,D$ vs. $E,F,G,H$

Take two groups of four islanders and compare them. One of two scenarios will happen; the two groups will weigh the same, one group will weigh more.

Scenario One $\rightarrow$ S1

If each side weighs the same, you know that all $8$ of the islanders on the seesaw weigh the same, and so they can be used as a known space for future comparisons to determine whether or not an imbalance is caused by someone being lighter or heavier

Second Weighing (S1)

$A,B$ vs. $I,J$

Since we have a known space, one of three scenarios will happen; The weight of $A,B$ will be $>,<$ or $=$ to the weight of $I,J$ .

Third Weighing (S1)

• If $A,B > I,J$ , we know that the one of $I,J$ weighs less. For the third weighing, simply put $I$ vs. $J$ and whichever is lighter has the different weight.

• If $A,B < I,J$ , we know that one of $I,J$ weighs more. For the third weighing, simply put $I$ vs. $J$ and whichever is heavier has the different weight.

• If $A,B = I,J$ , we know that one of $K,L$ is the odd one out. For the third weighing, simply put $A$ vs. $K$ . If they balance, $L$ has the different weight and if they don't, $K$ has the different weight.

Scenario Two $\rightarrow$ S2

If there is an imbalance, then we know that the odd one out is one of the eight islanders on the seesaw.

WLOG assume that person $D$ lies on the heavy side of the seesaw (we can assign letters at any point throughout the process so long as we keep track of who is who)

Second Weighing (S2)

$A,B,C,E$ vs. $D,J,K,L$

Islanders $J,K,L$ are all known space, they are of normal weight. The results of this weighing decide the third weighing:

Third Weighing S2

• If $D,J,K,L > A,B,C,E$ , then person $D$ must be heavy, or $E$ must be light. For the third weighing, simply put $I$ vs. $D$ . If they balance, $E$ has the different weight. If not, $D$ has the different weight.

• If $D,J,K,L < A,B,C,E$ , then person $E$ must have a normal weight since he would have tipped the scale towards his side in the first weighing if he was the heavier one. This means one of $A,B,C$ is heavy. For the third weighing, simply put $A$ vs. $B$ . If they balance $C$ has the different weight and if they don't balance, the heavier one has the different weight.

• If $D,J,K,L = A,B,C,E$ , then we know one of $F,G,H$ is light since last time they were used they were on the lighter side and all other islanders have been established as fixed space. For the third weighing, simply put $F$ vs $G$ . If they balance, then $H$ has the different weight and if they don't balance then the lighter one has the different weight.

This accounts for all of the islanders, so $\boxed{3}$ is the minimum number of uses of the seesaw to identify which has a different weight.