Thermo Inferno Challenge
A gas possessing isentropic expansion factor is made to undergo a thermodynamic cycle consisting of isobaric and isothermal processes each.If during the cycle,the ratio of maximum to minimum volume and ratio of maximum to minimum temperature .Then express the efficiency of the thermodynamic cycle in terms of and .Also assume to get a positive efficiency.
If find .
Details and Assumptions :
1) The isentropic expansion factor, also known as the adiabatic index of a gas is the ratio of its molar specific heat capacities at constant pressure and constant volume ; ; where a constant.
2) The gas is assumed to be ideal.The ideal gas law is always applicable.
3) refers to the natural log of ; .
4) are positive integers in their simplest form.They may or may not be distinct.
5) Just as a sidenote,you may want to investigate the cases when and approach or .
This problem is Original and made by me.Hope you liked it ! :D
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
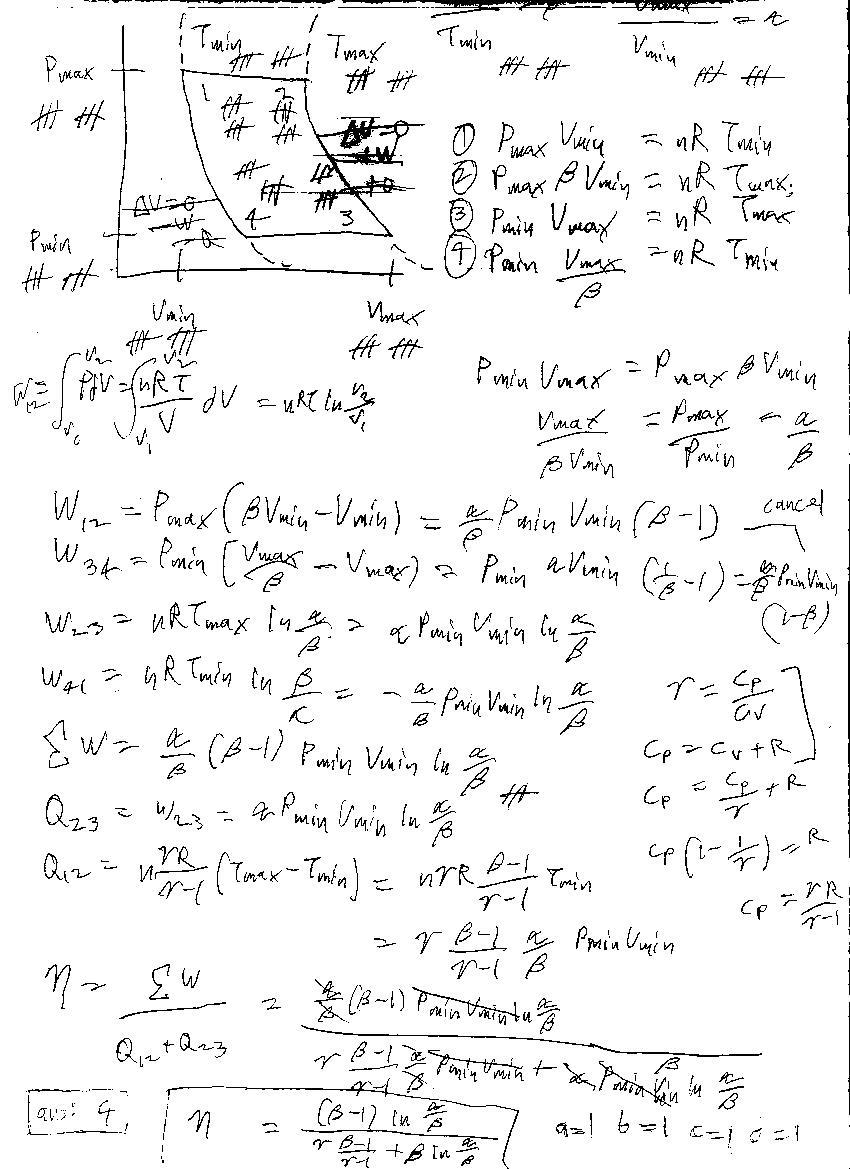
Actually one of my friends on AoPS had given this problem to me possessing the special case α = 4 ; β = 2 ; γ = 5 / 3 .Monoatomic gas.I solved it and decided it to generalise for α , β , γ .These are my results.
As of now I dont have time to write out the entire solution ( Cuz of exams and INJSO ) ; So I will just give the expression for the efficiency.Once INJSO ends I will Latex whole solution.
η = γ − 1 ( β − 1 ) γ + β l n ( β α ) ( β − 1 ) l n ( β α )
Thus a = b = c = d = 1
Hint : Use a bit of deduction ; Where will the system possess max volume.Where will it possess min volume ? Same for temperature.
I would strongly suggest drawing a PV plot and make correct deductions.