Thevenin/Norton Resistance

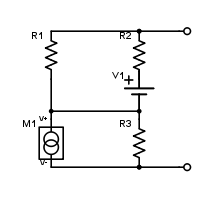
The elements of the linear circuit shown above have the following values:

• Resistors: $R_1=1.2 \text{ k}\Omega$ , $R_2=4.7 \text{ k}\Omega$ and $R_3=3.3 \text{ k} \Omega$
• Independent voltage sources: $V_1=12 \text{ V}$
• Independent current sources: $M_1=3 \text{ mA}$

Calculate the Thevenin equivalent resistance (in $\text{k} \Omega$ ) between the circular nodes. Round off your answer to three decimal places.