This is a Disphenoid!
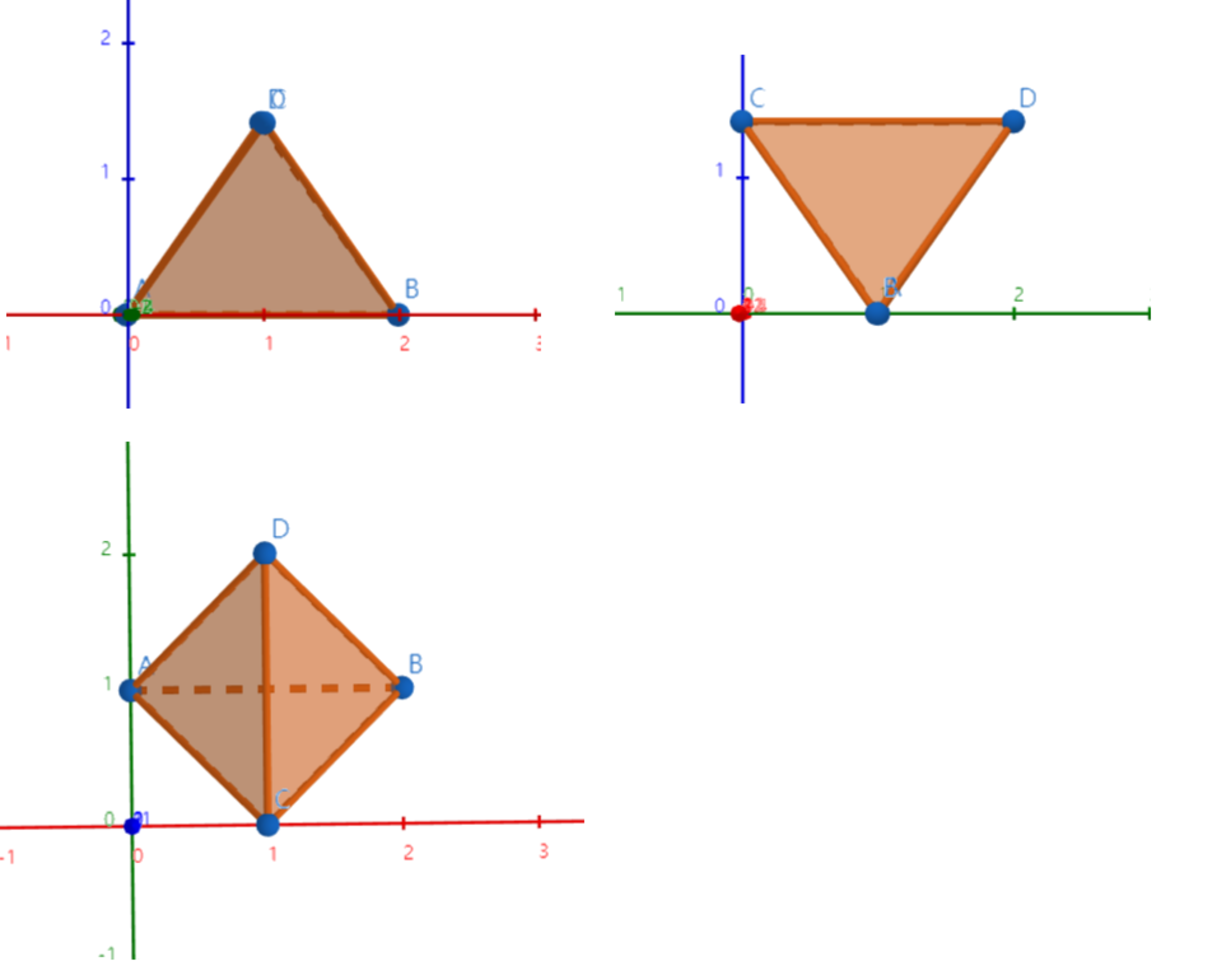 Here is the three-way-view of the solid, where the height of the triangle in the front-view and left-view is
, and the other lengths are defined above.
Here is the three-way-view of the solid, where the height of the triangle in the front-view and left-view is
, and the other lengths are defined above.
Find the volume of the solid.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
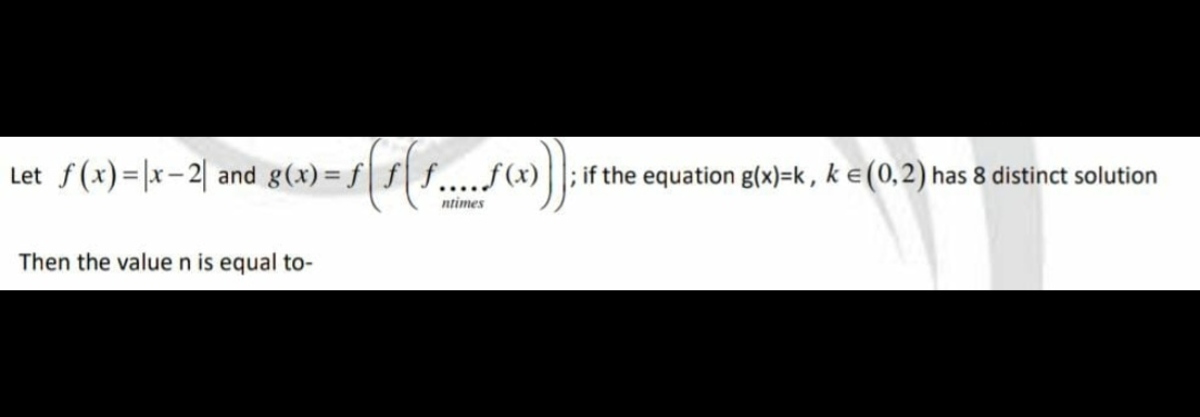
The vertices of this tetrahedron are A ( 0 , 1 , 0 ) , B ( 2 , 1 , 0 ) , C ( 1 , 0 , 2 ) and D ( 1 , 2 , 2 ) . Thus the volume of the tetrahedron is the modulus of 6 1 A D ⋅ ( A B × A C ) = 6 1 ⎝ ⎛ 1 1 2 ⎠ ⎞ ⋅ ⎝ ⎛ ⎝ ⎛ 2 0 0 ⎠ ⎞ × ⎝ ⎛ 1 − 1 2 ⎠ ⎞ ⎠ ⎞ = 6 1 ⎝ ⎛ 1 1 2 ⎠ ⎞ ⋅ ⎝ ⎛ 0 − 2 2 − 2 ⎠ ⎞ = − 3 2 2 and hence is 3 2 2 Alternatively, this solid is simply a regular tetrahedron of side length 2 , from which the result about the volume is immediate.