This isn't rocket science, it's rocket math!
 If the picture is too small, right click/two-finger click it and open it in a new tab.
If the picture is too small, right click/two-finger click it and open it in a new tab.
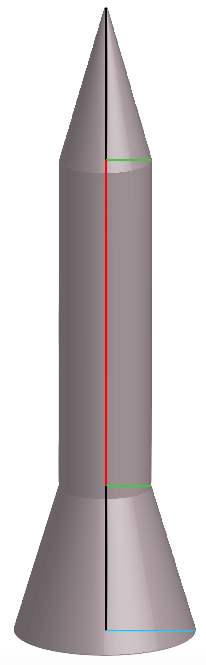
In this above rocket picture,
- The top black line serves as the height of the cone portion of the rocket and the bottom black line serves as the height of the "lamp shade" portion of the rocket. Both black lines have a length of 5 inches.
- The red line serves as the height of the cylindrical portion of the rocket and has a length of 10 inches.
- The green line serves as the radius of the cone portion and cylindrical portion. The green line also serves as the smaller radius of the lampshade portion. The length of the green line is 1 inch.
-
The blue line serves as the larger radius of the lampshade portion, and has a length of 2 inches.
If the surface area of the rocket (in square inches) equals S A , find ⌊ S A ⌋ .
Note: This "rocket" has a sealed base. Please include the base in your answer.
The answer is 139.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
CSA of the cone portion = π r l = π ⋅ 1 ⋅ h 2 + r 2 = π 2 6
CSA of cylinder portion = 2 π r h = 2 π ⋅ 1 ⋅ 1 0 = 2 0 π
CSA of frustum portion = π ( r 1 + r 2 ) h 2 + ( r 1 − r 2 ) 2 + π r 2 2 = 3 π 2 6 + 4 π
The Total SA, = 4 π 2 6 + 2 4 π ∼ 1 3 9 . 4 7 ⇒ ⌊ 1 3 9 . 4 7 ⌋ = 1 3 9
The point of this problem was that you didn't need the frustrum portion. You just needed to combine the top cone with the bottom frustrum to get a bigger cone. The ratios add up.
Log in to reply
Aah !! I can see it now !
We don't even need the radius of the cone thingy on the top, we only need similarities to prove that it is half of the radius of the lampshade thingy at the bottom.
Dont you think that was a good practice for Boards Summatives..!! Ahhh....
But did you include the base? It is a rocket and has an open base.
The two conical bits (frustum at the bottom and cone at the top) have the same height, and the radius of the top cone is half the radius of the bottom of the frustum. Proportions therefore allow us to put these two pieces together to produce a cone of height 2*5 = 10. The slant length, l , of this cone is 1 0 2 + 2 2 , by Pythagoras' Theorem. Since the curved surface area of a cone is π r l , we find that it is 2 π 1 0 2 + 2 2 .
Next, the area of a circle is π r 2 , so the area of the base is 4 π .
Finally the curved surface area of a cylinder is π r 2 h . This is 2 0 π here.
The total is 2 π 1 0 4 + 4 π + 2 0 π .
This value is just short of 1 4 0 , hence the answer of 1 3 9 .
you should ve wrote that you must round it
Relevant wiki: Surface Area - Problem Solving
Let A be the surface area of the top part, B be the surface area of the middle part and C be the surface area of the bottom part. Then,
A = 2 1 c L = 2 1 ( π ) ( 2 ) 5 2 + 1 2 = π 2 6
B = c h = 2 π ( 1 0 ) = 2 0 π
C = 2 1 ( c 1 + c 2 ) L + π r 2 = 2 1 π ( 2 + 4 ) 5 2 + 1 2 + π ( 2 2 ) = 2 1 π ( 6 ) 2 6 + 4 π = 3 π 2 6 + 4 π
Finally,
S A = A + B + C = π 2 6 + 2 0 π + 3 π 2 6 + 4 π ≈ 1 3 9 a n s w e r
Definitions:
c = circumference of the circle
L = slant height
r = radius of the circle
h = height
c 1 = upper circumference
c 2 = lower circumference