Three check chess: Forced Victory?
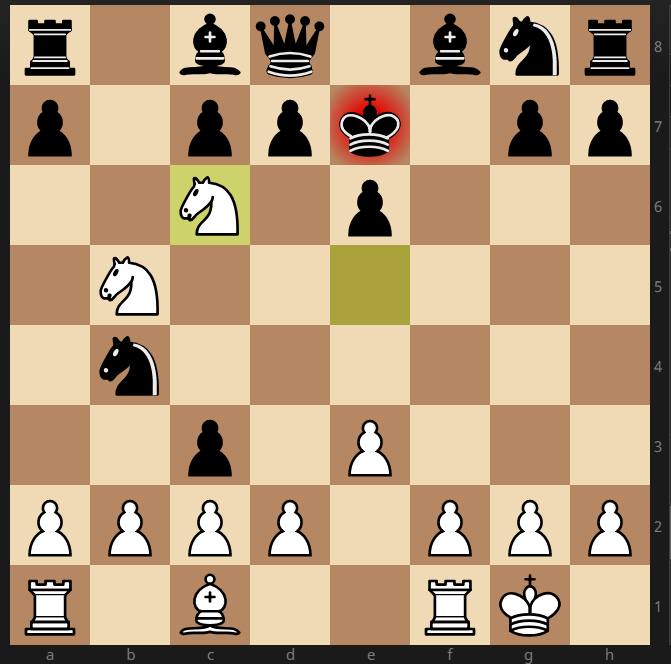
This is a variation of chess called three-check chess where you can win either by checking your opponent three times or just a standard mate. In this game, black has made a slight inaccuracy and played pawn to b5, so now it is white's turn to play.
Assuming that from this point onward, both sides play optimally: is there a chance that white can win this game by either mate or a forced 3 check sequence, and if so in how many moves?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
The correct answer is 8 (a forced three check sequence). Here is the sequence: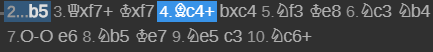
ending in inevitable three check.
