Three half circles and one mini circle
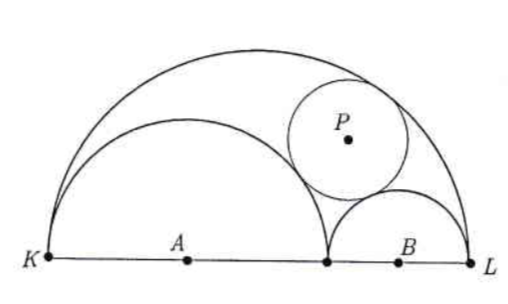
Given K A = 3 and L B = 2 . If the radius of circle with center P can be written as b a , where a and b are positive coprime integers, what is a + b ?
Source: Syrian math olympiad-regional 2019
The answer is 49.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
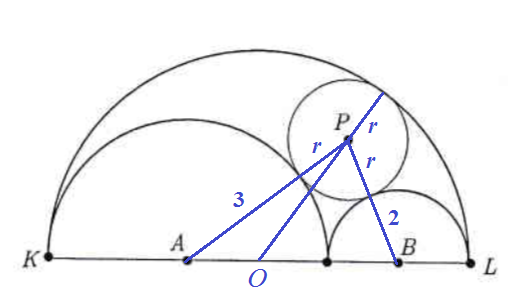
Let the radius of circle with center P be r and the center of the biggest semicircle be O ( 0 , 0 ) . Then A ( − 2 , 0 ) and B ( 3 , 0 ) and point P satisfies the following three equations of circle:
⎩ ⎪ ⎨ ⎪ ⎧ ( x + 2 ) 2 + y 2 = ( 3 + r ) 2 ( x − 3 ) 2 + y 2 = ( 2 + r ) 2 x 2 + y 2 = ( 5 − r ) 2 . . . ( 1 ) . . . ( 2 ) . . . ( 3 )
⎩ ⎪ ⎨ ⎪ ⎧ x 2 + 4 x + 4 + y 2 = 9 + 6 r + r 2 x 2 − 6 x + 9 + y 2 = 4 + 4 r + r 2 x 2 + y 2 = 2 5 − 1 0 r + r 2 . . . ( 1 ) . . . ( 2 ) . . . ( 3 )
{ ( 1 ) − ( 3 ) : ( 3 ) − ( 2 ) : 4 x + 4 = − 1 6 + 1 6 r 6 x − 9 = 2 1 − 1 4 r ⟹ x = 4 r − 5 ⟹ 3 x = 1 5 − 7 r . . . ( 4 ) . . . ( 5 )
3 × ( 4 ) − ( 5 ) : 1 9 r − 3 0 ⟹ r = 0 = 1 9 3 0
Therefore, a + b = 1 9 + 3 0 = 4 9 .
I think you meant (3)-(2) instead of (3)-(1) :)
Solution by: Nibedan Norman Mukherjee