Three segments don't simply become medians of a triangle!
a , b and c are the medians of a triangle with non-zero area.
Which numbers cannot be length of a , b and c , respectively?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
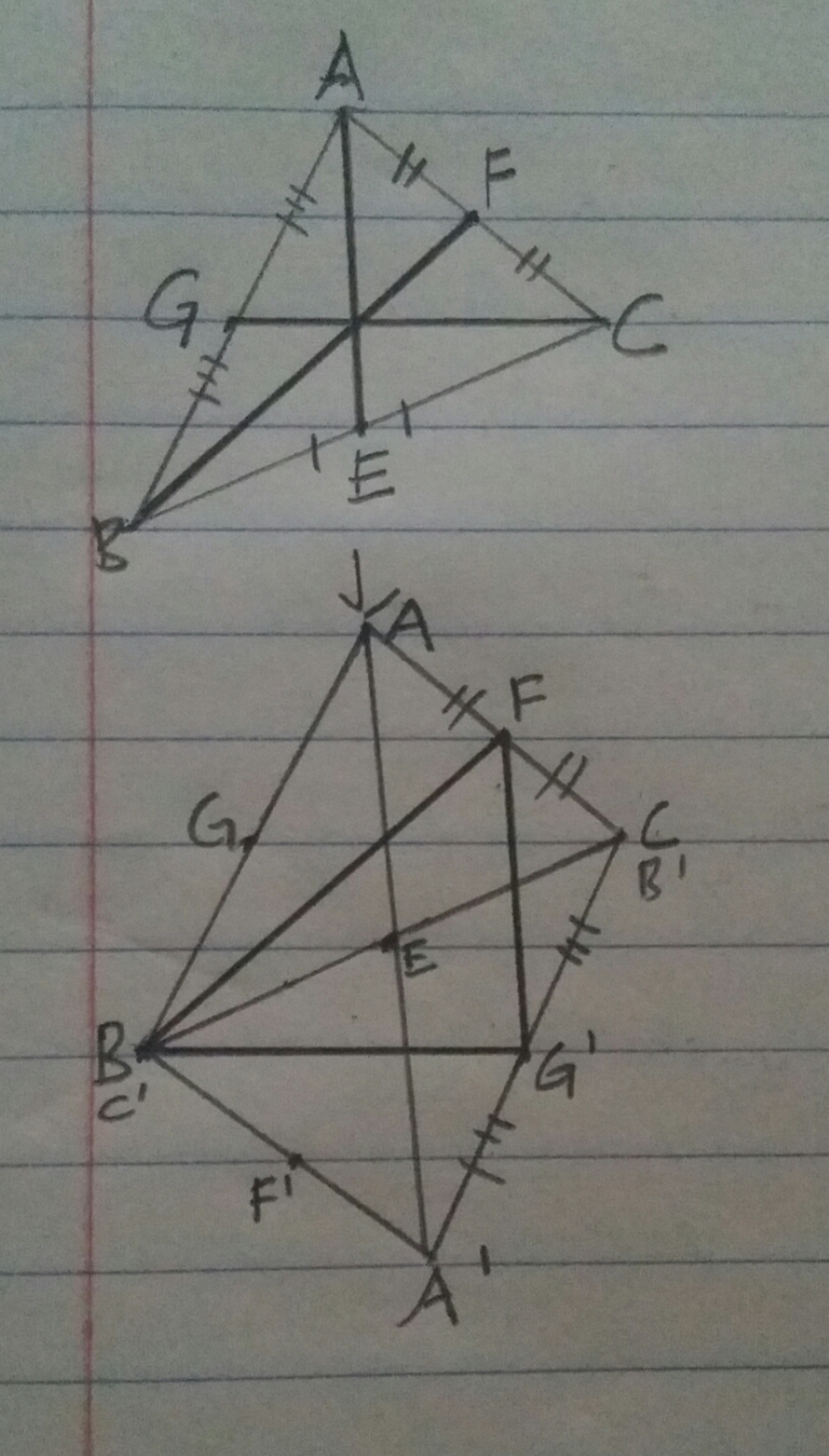 Let the triangle as
△
A
B
C
, and
E
,
F
,
G
as the medium of
B
C
,
A
C
,
A
B
respectively. Hence, the median are
A
E
,
B
F
,
C
G
.
Now, rotate the triangle 180° with center point
E
. From the new diagram, we can get a new triangle
△
B
F
G
′
.Besides that,
C
F
=
C
A
,
C
G
′
=
G
′
A
′
, so
F
G
′
=
2
1
A
A
′
=
A
E
. Note that
B
G
′
=
C
′
G
′
=
C
G
. Hence, the sides of this new triangle are the original median respectively.
Let the triangle as
△
A
B
C
, and
E
,
F
,
G
as the medium of
B
C
,
A
C
,
A
B
respectively. Hence, the median are
A
E
,
B
F
,
C
G
.
Now, rotate the triangle 180° with center point
E
. From the new diagram, we can get a new triangle
△
B
F
G
′
.Besides that,
C
F
=
C
A
,
C
G
′
=
G
′
A
′
, so
F
G
′
=
2
1
A
A
′
=
A
E
. Note that
B
G
′
=
C
′
G
′
=
C
G
. Hence, the sides of this new triangle are the original median respectively.
Hence, we can apply the theorem 'sum of two sides of triangle is larger than other side'. By try and error, we can get that 1 0 + 1 2 = 2 2 < 2 3 , so 1 0 , 1 2 , 1 3 c a n n o t be the length of a , b , c .
Proof of necessity is build upon the very inspiration of the problem, which I found on the brilliant wiki Area of a Triangle .
Now,
A = 3 4 σ ( σ − u ) ( σ − v ) ( σ − w ) ⟹ A = 3 1 ( u + v + w ) ( v + v − w ) ( u + w − v ) ( v + w − u )
Now the claim of necessity is obvious; as sum of any two of u , v and w must be greater than the third one; otherwise the square root will be undefined.
First, read this page about Constructing a Triangle with Three Medians Given . Of course, this construction is based on the classic proof of internal division ratio 2 : 1 of the medians at their point of concurrency.
Just notice, as long as the sum of 3 2 portions of any two medians are greater than the 3 2 portion of the third one, or equivalently, the sum of any two medians is greater than the third one, this construction is possible . That proves the claim of sufficiency.
Only the medians candidate set ( a , b , c ) = ( 1 0 , 1 2 , 2 3 ) , among the 4 alternatives, breaks the condition.