Thrown for a loop

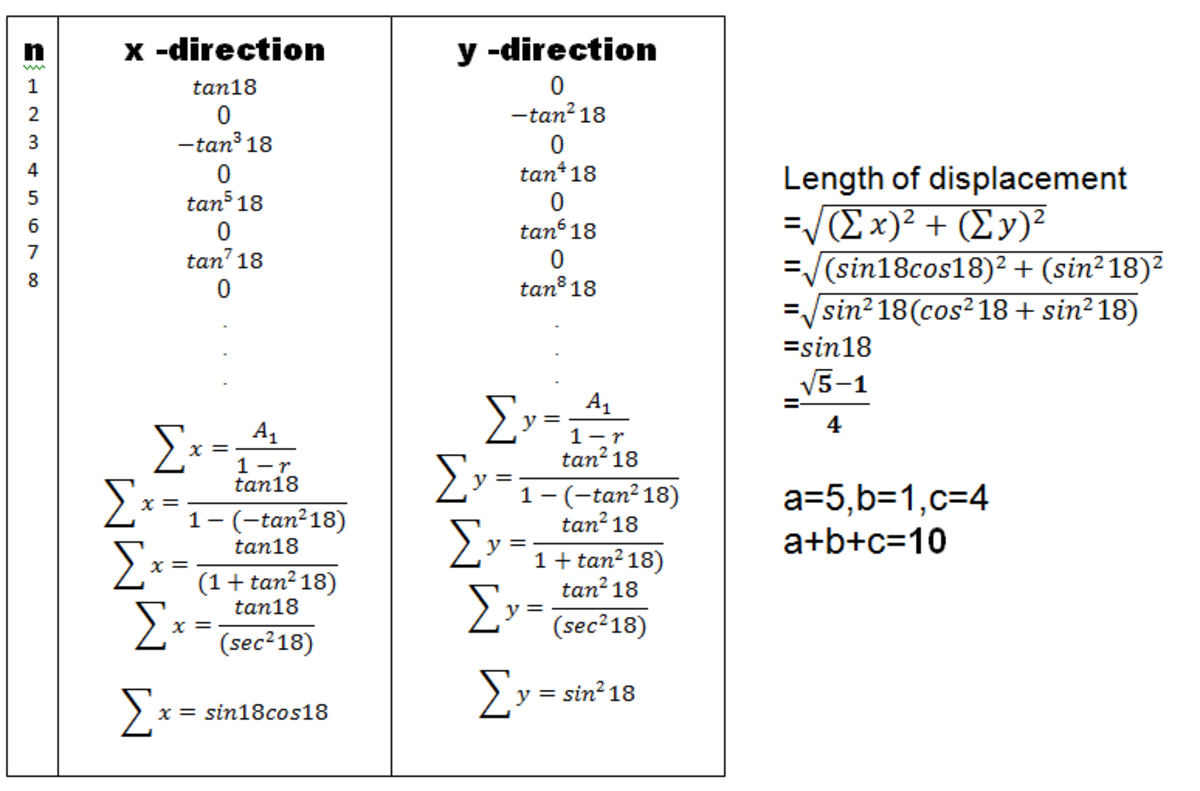
Letting $\theta = \frac{\pi}{10},$ the distance the particle travels in the positive $x$ direction is

$\displaystyle\sum_{k=1}^{\infty} (-1)^{k+1}\tan^{2k-1}(\theta) = \dfrac{\tan(\theta)}{1 + \tan^{2}(\theta)} = \tan(\theta)\cos^{2}(\theta) = \sin(\theta)\cos(\theta).$

The distance the particle travels in the positive $y$ direction is

$\displaystyle\sum_{k=1}^{\infty} (-1)^{k+1} \tan^{2k}(\theta) = \dfrac{\tan^{2}(\theta)}{1 + \tan^{2}(\theta)} = \sin^{2}(\theta).$

The distance between the starting and finishing points is then

$\sqrt{\sin^{2}(\theta)\cos^{2}(\theta) + \sin^{4}(\theta)} = \sqrt{\sin^{2}(\theta)(\cos^{2}(\theta) + \sin^{2}(\theta))} = |\sin(\theta)|.$

With $\theta = \frac{\pi}{10} = 18^{\circ},$ the desired distance is

$\sin(18^{\circ}) = \dfrac{\sqrt{5} - 1}{4},$ and so $a + b + c = 5 + 1 + 4 = \boxed{10}.$