Tiny Tidy Corner
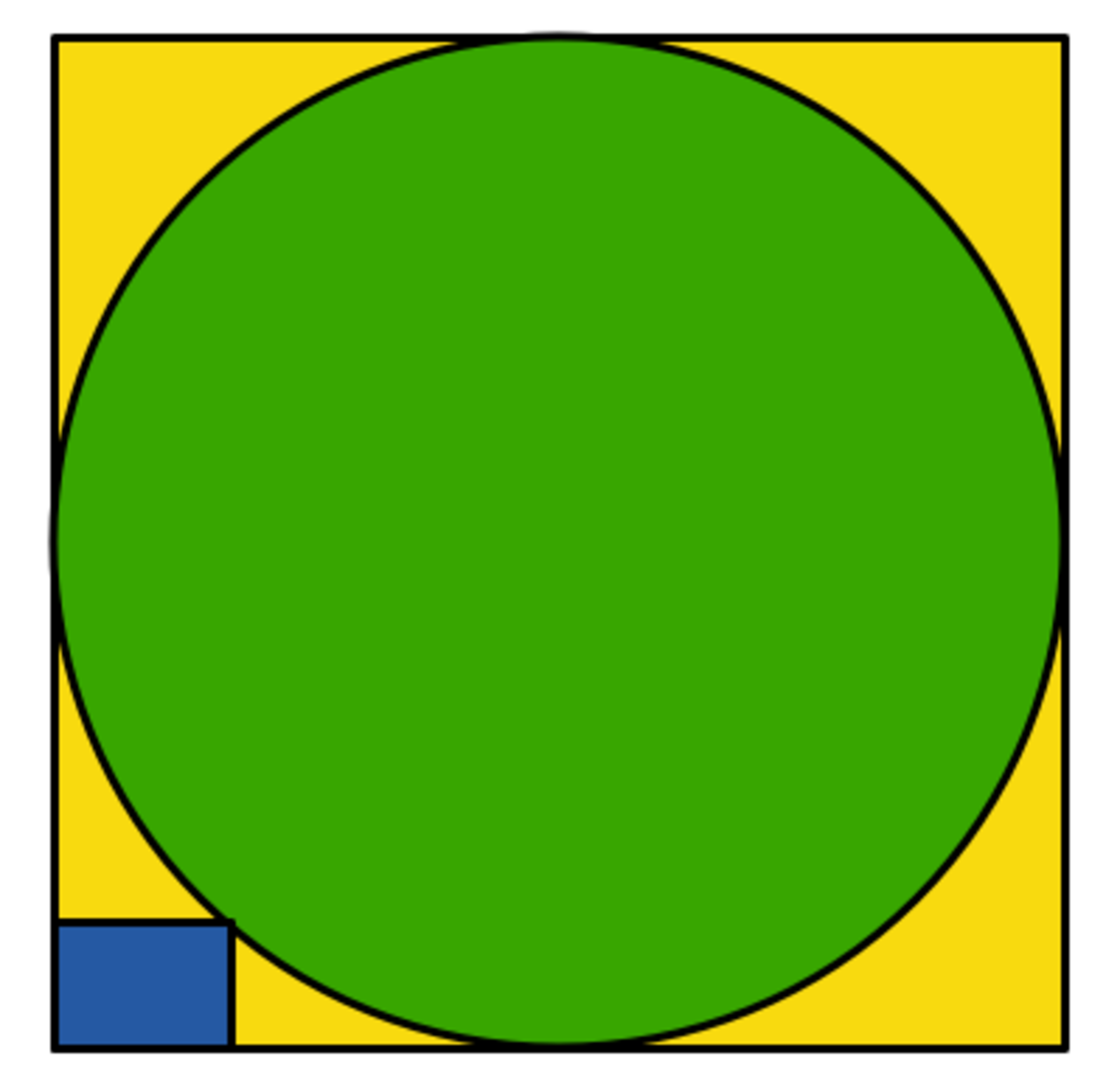
In the yellow square, we inscribe the green circle. The blue rectangle is flush against the corner of the square, and touches the circumference of the green circle.
If the blue rectangle has dimensions 2 × 9 , what is the radius of the green circle?
The answer is 17.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Without loss of generality let the vertical dimension of the blue square be 2 and the horizontal dimension 9 .
Let P be the upper right corner of the blue square, O the center of the circle and Q the point of intersection of the extension of the upper side of the blue square and the vertical diameter of the circle.
Then Δ O P Q is a right triangle with hypotenuse ∣ O P ∣ = r , the radius of the circle, and side lengths ∣ O Q ∣ = r − 2 and ∣ P Q ∣ = r − 9 . By Pythagoras, we then have that
r 2 = ( r − 2 ) 2 + ( r − 9 ) 2 ⟹ r 2 = r 2 − 4 r + 4 + r 2 − 1 8 r + 8 1 ⟹
r 2 − 2 2 r + 8 5 = 0 ⟹ ( r − 5 ) ( r − 1 7 ) = 0 .
As r must exceed either dimension of the blue square, we conclude that r = 1 7 .
 Consider the diagram on my left. By Pythagorean theorem, we have
Consider the diagram on my left. By Pythagorean theorem, we have
R 2 = ( R − 9 ) 2 + ( R − 2 ) 2
R 2 = R 2 − 1 8 R + 8 1 + R 2 − 4 R + 4
R 2 − 2 2 R + 8 5 = 0
By factoring, we have,
( R − 1 7 ) ( R − 5 ) = 0
R = 1 7 or R = 5
We use R = 1 7 because R = 5 is less than 9 .
