A Tricky Semicircle

Use $\text{SOH}$ $\implies$ $sine=\dfrac{opposite~side}{hypotenuse}$

$sin~60=\dfrac{r}{2}$

$r=2~sin~60$

The diameter therefore is

$d=2r=2(2~sin~60) = 3.464$

By pythagorean theorem, $AE=\sqrt{4^2-2^2}=\sqrt{16-4}=\sqrt{12}$

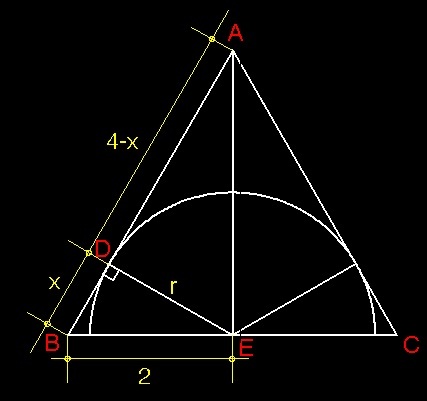
Apply Pythagorean Theorem at right $\triangle BDE$ $\implies$ $r^2=2^2-x^2=4-x^2$

Apply Pythagorean Theorem at right $\triangle EDA$ $\implies$ $r^2=(\sqrt{12})^2-(4-x)^2=-4+8x-x^2$

$r^2$ is equal to $r^2$ $\implies$ $4-x^2=-4+8x-x^2$ $\implies$ $1=x$

Solving for $r$ , we get $\implies$ $r^2=4-1^2=4-1=3$ $\implies$ $r=\sqrt{3}$ .

Hence, $d=2r=2\sqrt{3}\approx3.464$

Let r be radius of circle and h the height of triangle. The vertical line would cut the triangle in two equal half triangles with sides 4,2 and h. The area of half triangle with base 2 is 2h/2=h and with base 4 and height r is 4r/2=2r. Therefore, 2r=h. Using Pythagoras theorem, h^2+2^2=4^2, implies h=2√3.Hence, 2r=h=2√3.