Total Area with stacking Squares
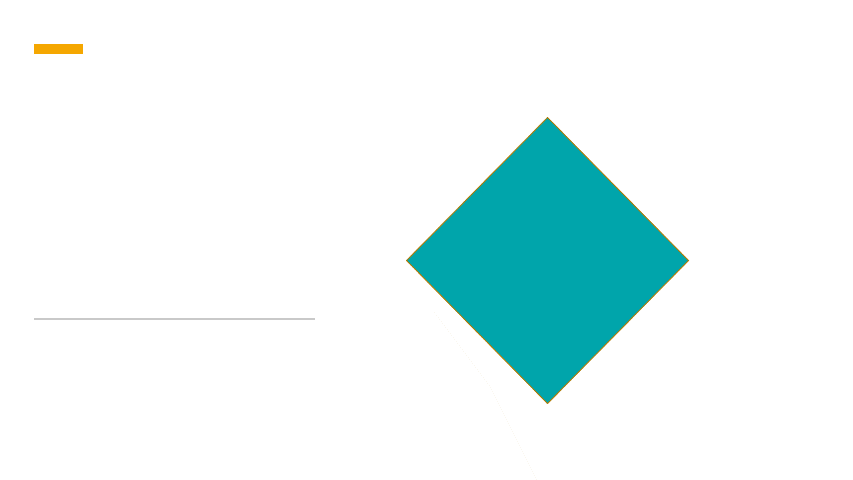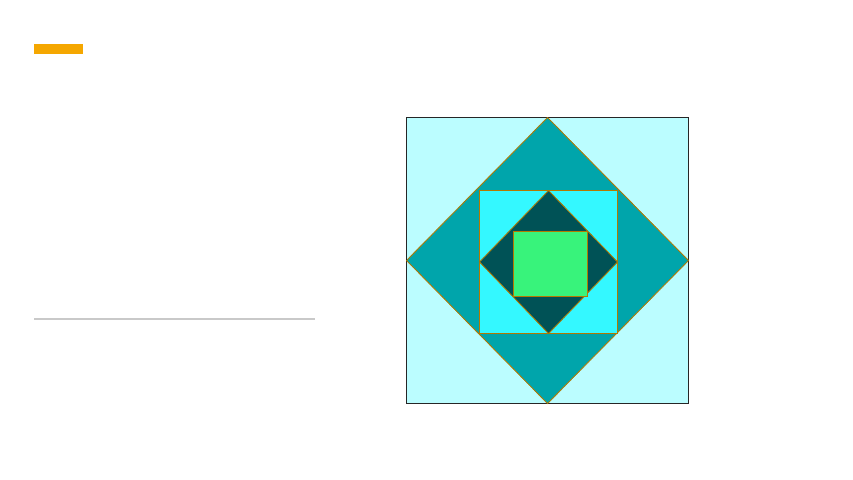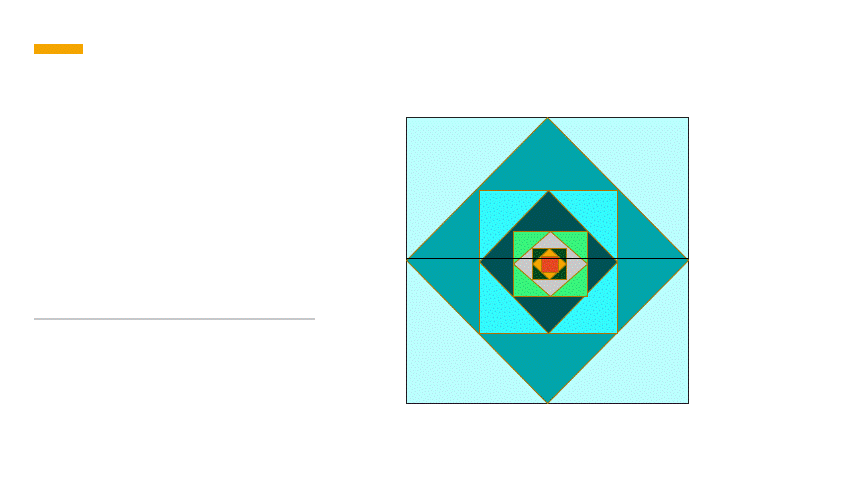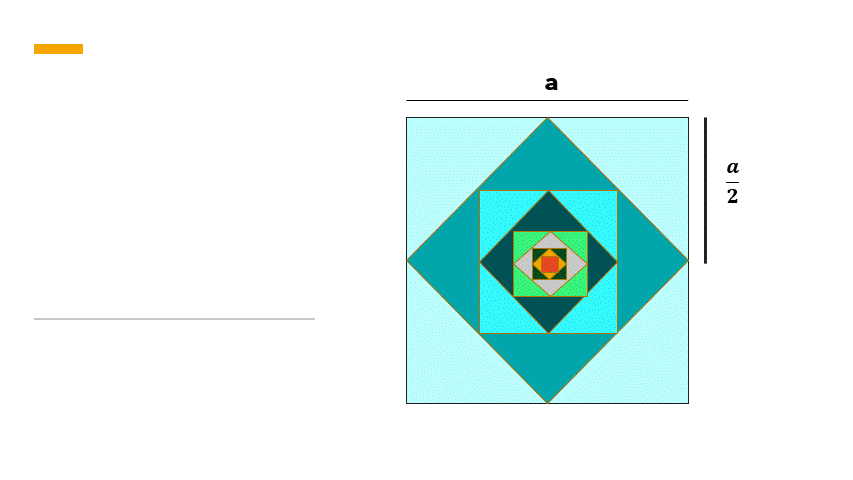 Let
A
1
be the area of the squares with sides parallel to the
x
- and
y
-axes and
A
2
be the area of the squares with slanted sides.
Let
A
1
be the area of the squares with sides parallel to the
x
- and
y
-axes and
A
2
be the area of the squares with slanted sides.
What is A 2 A 1 ?
The answer is 2.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Thank you.
A square with sides parallel to the x − and y − axes can be broken up into 8 congruent triangles so that 4 of those triangles make up a square with slanted sides.

Therefore, the ratio of the area of one square in A 1 to the area of its connected square in A 2 is 4 8 = 2 .
Since each square in A 1 has one connected square in A 2 , this ratio is maintained throughout the whole diagram, so A 2 A 1 is also 2 .
Thank you.
If the value of a is say 2 , then the length of the inner square ( A 2 ) will be 2
\[\begin{cases} A_{1} = (2)^2 = 4 \\ A_{2} = (\sqrt{2})^2 = 2 \end{cases}
\implies \dfrac{A_{1}}{A_{2}} = \dfrac{4}{2} = \boxed{2}\]
Thank you.
In the given animation, each square with side parallel to the x − and y − axes , i.e., each square in the sum A 1 has a corresponding square of half the area in the squares in the sum A 1 .
⟹ A 1 = 2 × A 2
⟹ A 2 A 1 = A 2 2 × A 2
⟹ A 2 A 1 = 2