Trace the Dot!
Here are 2 vectors
Let vector
Let
be the length of the dot
’s trace, which can be expressed as
, which are all square-free.
Then, give your answer as .
The answer is 294.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
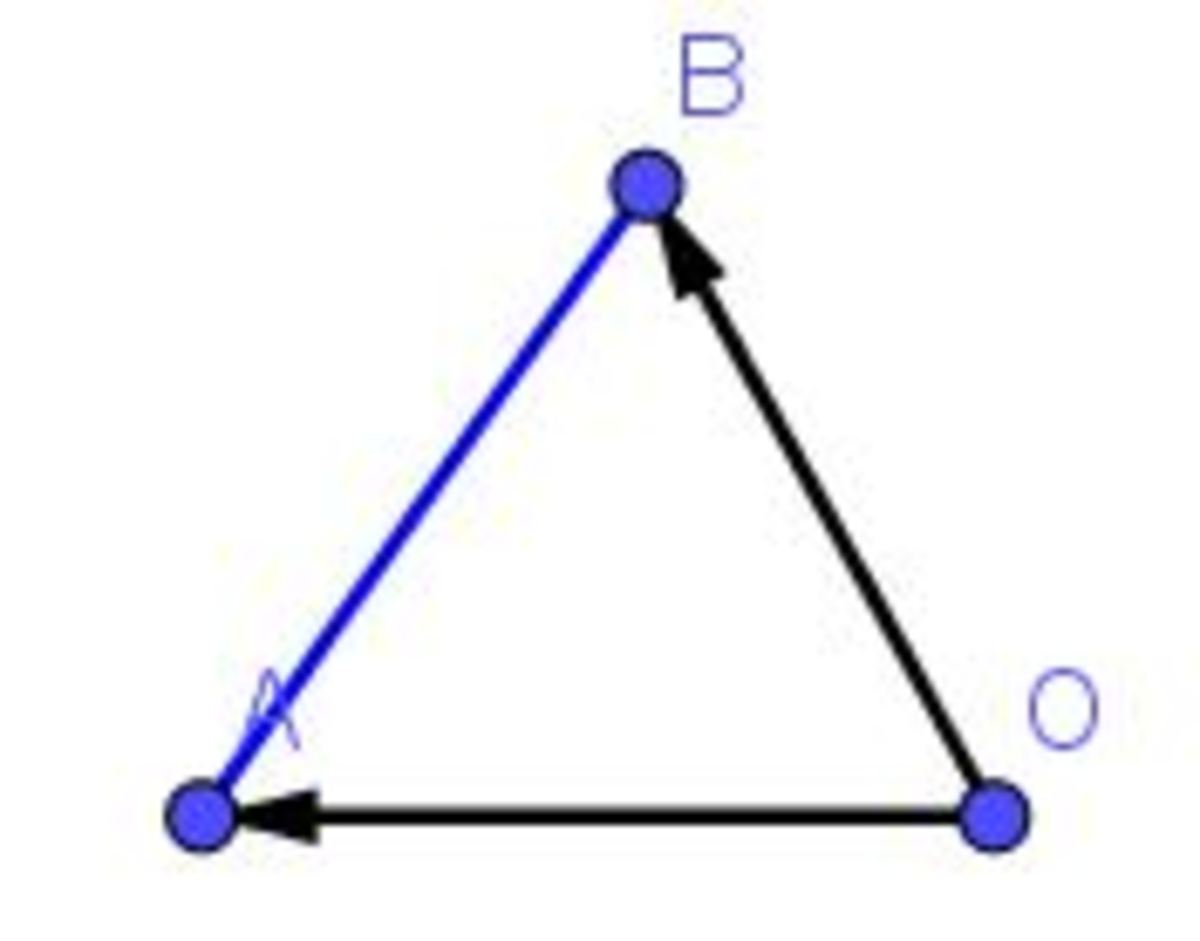
First, cut the range of t to better understand the vector O P .
i ) − 2 ≤ t < 0 O P = ( 1 − t ) O A + ( 2 + t ) O B , O B = 3 − O A When t = − 2 , O P = 3 O A and when t = 0 , O P = O A + 2 O B Thus, when − 2 ≤ t < 0 , trace of the dot P is like the blue line below: i
i
)
0
≤
t
<
1
O
P
=
(
1
−
t
)
O
A
+
(
2
−
t
)
O
B
,
O
B
=
1
+
O
A
When
t
=
1
,
O
P
=
O
B
Thus, when
0
≤
t
<
1
, trace of the dot
P
is like the blue line below:
i
i
)
0
≤
t
<
1
O
P
=
(
1
−
t
)
O
A
+
(
2
−
t
)
O
B
,
O
B
=
1
+
O
A
When
t
=
1
,
O
P
=
O
B
Thus, when
0
≤
t
<
1
, trace of the dot
P
is like the blue line below:
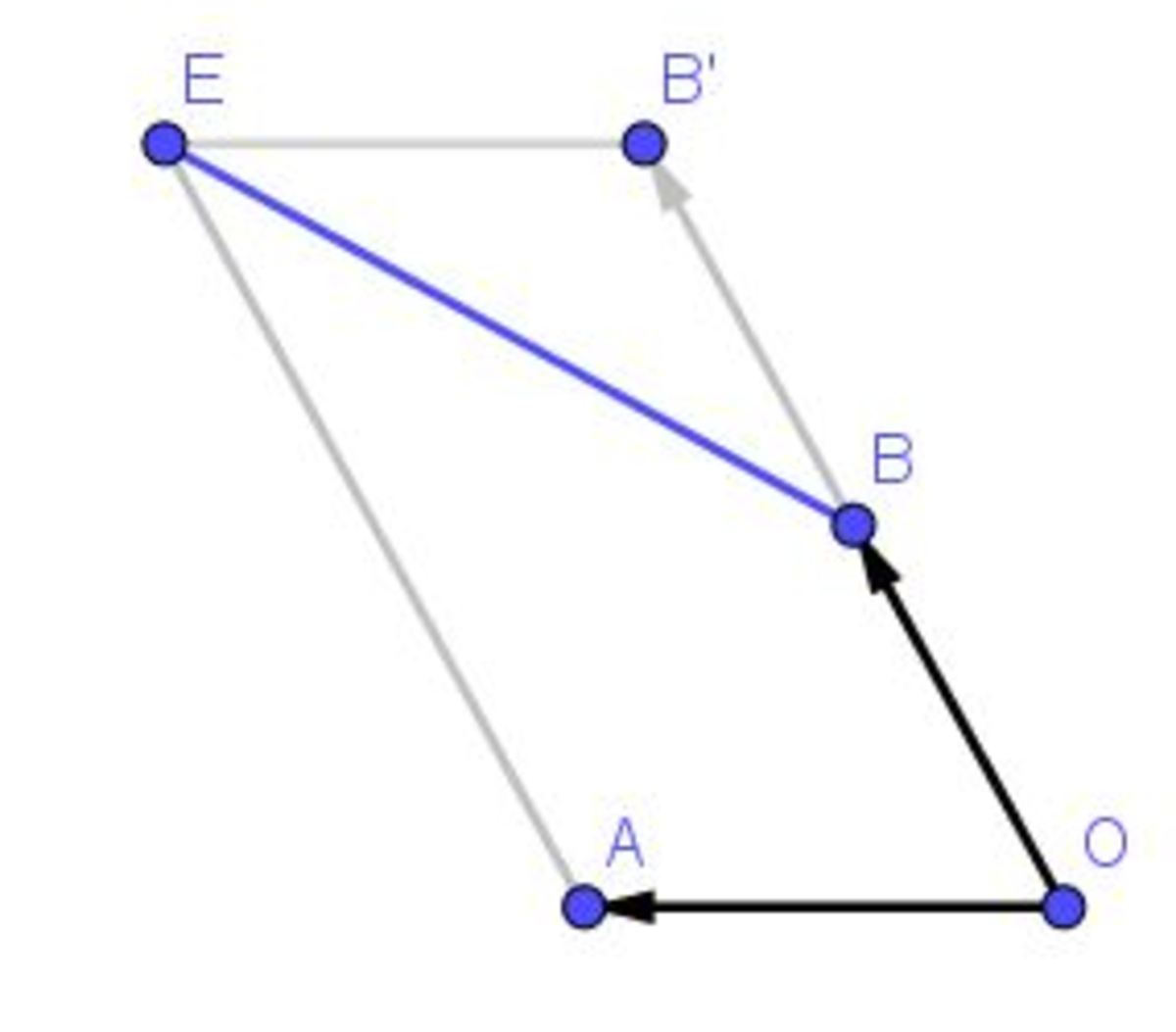 i
i
i
)
1
≤
t
<
2
O
P
=
(
t
−
1
)
O
A
+
(
2
−
t
)
O
B
,
O
B
=
1
−
O
A
When
t
=
2
,
O
P
=
O
A
Thus, when
1
≤
t
<
1
, trace of the dot
P
is like the blue line below:
i
i
i
)
1
≤
t
<
2
O
P
=
(
t
−
1
)
O
A
+
(
2
−
t
)
O
B
,
O
B
=
1
−
O
A
When
t
=
2
,
O
P
=
O
A
Thus, when
1
≤
t
<
1
, trace of the dot
P
is like the blue line below:
 Therefore, the whole trail of dot
P
is like the blue line below:
Therefore, the whole trail of dot
P
is like the blue line below:
 A
′
(
−
1
2
,
0
,
0
)
,
B
′
(
3
,
2
,
2
)
,
E
(
2
,
4
,
4
)
A
′
(
−
1
2
,
0
,
0
)
,
B
′
(
3
,
2
,
2
)
,
E
(
2
,
4
,
4
)
Finally, find the length of L . A ′ E 2 = 2 2 8 = a , E B 2 = 9 = b 2 , B A 2 = 5 7 = c a + b 2 + c = 2 9 4 .