Track & Trace
 The ends of a stiff bar
of length 4 slide freely inside a parabolic track (specifically, the parabola
). As they do, the midpoint
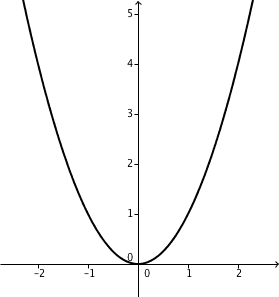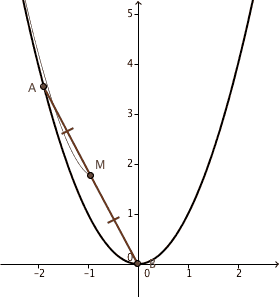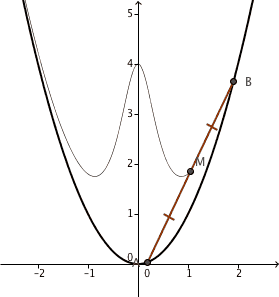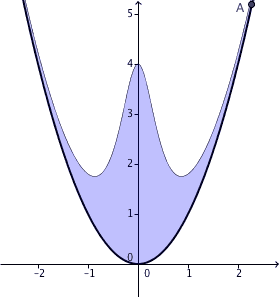
of that bar traces a curve. Find the area of the region between the parabola and the curve traced by
.
The ends of a stiff bar
of length 4 slide freely inside a parabolic track (specifically, the parabola
). As they do, the midpoint
of that bar traces a curve. Find the area of the region between the parabola and the curve traced by
.
Assume that the bar slides infinitely in both directions.
The answer is 6.2831853.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Consider the parametric coordinates for the end points of the rod as :
( t 1 , t 1 2 ) and ( t 2 , t 2 2 )
The constraints on these two points is that, the distance between them is always 4 .
Thus,
( t 2 2 − t 1 2 ) 2 + ( t 2 − t 1 ) 2 = 4
( t 1 2 + t 2 2 − 2 t 1 t 2 ) ( 1 + t 1 2 + t 2 2 + 2 t 1 t 2 ) = 1 6 − − − ( 1 )
This will be our constraint. Now, consider the mid-point of the bar, which has the coordinates :
( X , Y ) = ( 2 t 1 + t 2 , 2 t 1 2 + t 2 2 )
We need to find the locus of this point.
X = 2 t 1 + t 2 Y = 2 t 1 2 + t 2 2
⇒ 2 X = t 1 + t 2 − − − ( 2 ) ⇒ 2 Y = t 1 2 + t 2 2 − − − ( 3 )
Playing with the above two equations, we get,
2 X 2 − Y = t 1 t 2 − − − ( 4 )
We can now eliminate t 1 and t 2 from our constraint equation, by substituting from ( 2 ) , ( 3 ) and ( 4 )
Doing so, the constraint equation simplifies to :
( 4 Y − 4 X 2 ) ( 1 + 4 X 2 ) = 1 6
Y = X 2 + 1 + 4 X 2 4
We can now switch back to lower-case letters. (I used uppercase to avoid any confusion)
y = x 2 + 1 + 4 x 2 4
This is the locus of the midpoint of the bar
Now, the area between a function f ( x ) and g ( x ) with f ( x ) > g ( x ) , is given by the integral of the difference of the functions, with limits as the x-coordinates of the intersection points of the two functions.
Hence, the area between the graph of y = x 2 + 1 + 4 x 2 4 and y = x 2 is :
A = ∫ − ∞ ∞ ( x 2 + 1 + 4 x 2 4 ) − ( x 2 ) d x
A = ∫ − ∞ ∞ 1 + 4 x 2 4 d x
A = 2 π ≈ 6 . 2 8