Transforming Triangle 2

From the inspiration problem , we discovered that if $P$ is a point inside an equilateral triangle $\triangle QRS$ , then equilateral triangles can be taken out of $\triangle QRS$ and the remaining triangles can be rearranged to make a triangle with double the sides of $PQ$ , $PR$ , and $PS$ as shown below, which means that $\angle QPR$ , $\angle QPS$ , and $\angle RPS$ are exactly $60°$ more than corresponding angles in the non-equilateral triangle.

In this case, double $PQ$ , $PR$ , and $PS$ are $10$ , $70\sqrt{3}$ , are $20\sqrt{37}$ , and since $10^2 + (70\sqrt{3})^2 = (20\sqrt{37})^2$ , these form a right triangle where the angle between $10$ and $70\sqrt{3}$ is $90°$ . Therefore, the angle between $5$ and $35\sqrt{3}$ near Brilli the Ant is $90° + 60° = 150°$ , and using the law of cosines, the third side (and also the side of the equilateral triangle) solves to $\sqrt{5^2 + (35\sqrt{3})^2 - 2 \cdot 5 \cdot 35\sqrt{3} \cos 150°} = \boxed{65}$ .

Let the side length of the equilateral triangle be $a$ and the coordinates of the top, left and right vertices be $\left(0, \frac {\sqrt 3}2a\right)$ , $\left(-\frac 12a, 0 \right)$ , and $\left(\frac 12a, 0 \right)$ respectively, and the coordinates where Brilli is $(x,y)$ . Then by Pythagorean theorem :

$\begin{cases} (x-0)^2 + \left(y-\frac {\sqrt 3}2a\right)^2 = 5^2 & \implies x^2 + y^2 - \sqrt 3ay + 0.75a^2 = 25 & ...(1) \\ \left(x+\frac 12a\right)^2 + (y-0)^2 = (10\sqrt {37})^2 & \implies x^2 + ax + 0.25a^2 + y^2 = 3700 & ...(2) \\ \left(x-\frac 12a\right)^2 + (y-0)^2 = (35\sqrt 3)^2 & \implies x^2 - ax + 0.25a^2 + y^2 = 3675 & ...(3) \end{cases}$

From $(2)-(3): \implies 2ax = 25 \implies ax = 12.5 \quad ...(4)$

From $(2)+(3): \implies 2x^2 + 0.5a^2 + 2y^2 = 7375 \implies x^2 + y^2 = 3687.5 - 0.25a^2 \quad ...(5)$

From $(2)-(1): \implies ax + \sqrt 3ay - 0.5a^2 = 3675 \implies \sqrt 3a y = 3662.5 + 0.5a^2 \quad ...(6)$

Therefore, we have:

$\begin{aligned} {\color{#3D99F6}(\sqrt 3ax)^2} + \color{#D61F06}(\sqrt 3ay)^2 & = {\color{#3D99F6}(12.5\sqrt 3)^2} + \color{#D61F06} (3662.5 + 0.5a^2)^2 \\ 3a^2\color{#3D99F6}(x^2+y^2) & = 468.75 + 13413906.25 + 3662.5a^2 + 0.25a^4 & \small \color{#3D99F6} (5): \ x^2 + y^2 = 3687.5 - 0.25a^2 \\ 3a^2\color{#3D99F6}(3687.5 - 0.25a^2) & = 13414375 + 3662.5a^2 + 0.25a^4 \end{aligned}$

$\begin{aligned} \implies a^4 - 7400a^2 + 13414375 & = 0 \\ (a^2 - 4225)(a^2-3175) & = 0 \end{aligned}$

$\implies a = \begin{cases} 65 \\ 5\sqrt{127} \end{cases}$

Since $5\sqrt{127}<35\sqrt 3 < 10\sqrt{37}$ , the answer is $a=\boxed{65}$ .

I solved it as an algebra problem in 3 unknowns: the $x$ and $y$ coordinates of Brilli and $s$ , the length of the side of the equilateral triangle with vertices at $\{0,0\}$ , $s\{\frac12,\frac{\sqrt{3}}{2}\}$ and $s\{1,0\}$ . Unfortunately, there are two solutions. On the basis that the problem implicitly specified an integer side length and that Brilli was inside the triangle, I selected the 65 side length as the submitted solution. The two solutions are: $\left\{\left\{s\to 65,x\to \frac{425}{13},y\to \frac{385 \sqrt{3}}{13}\right\},\left\{s\to 5 \sqrt{127},x\to \frac{320}{\sqrt{127}},y\to 350 \sqrt{\frac{3}{127}}\right\}\right\}$ .

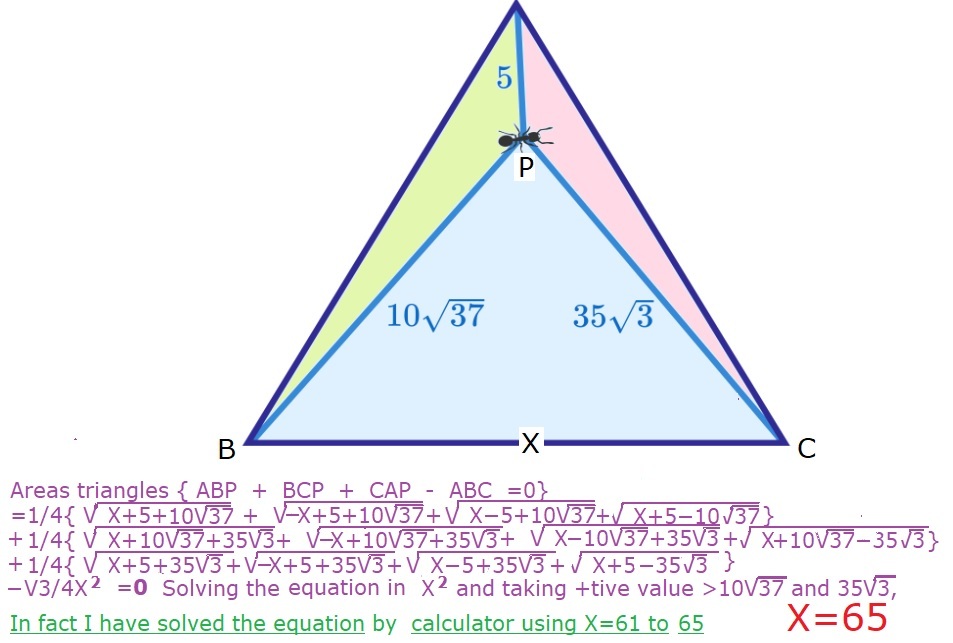
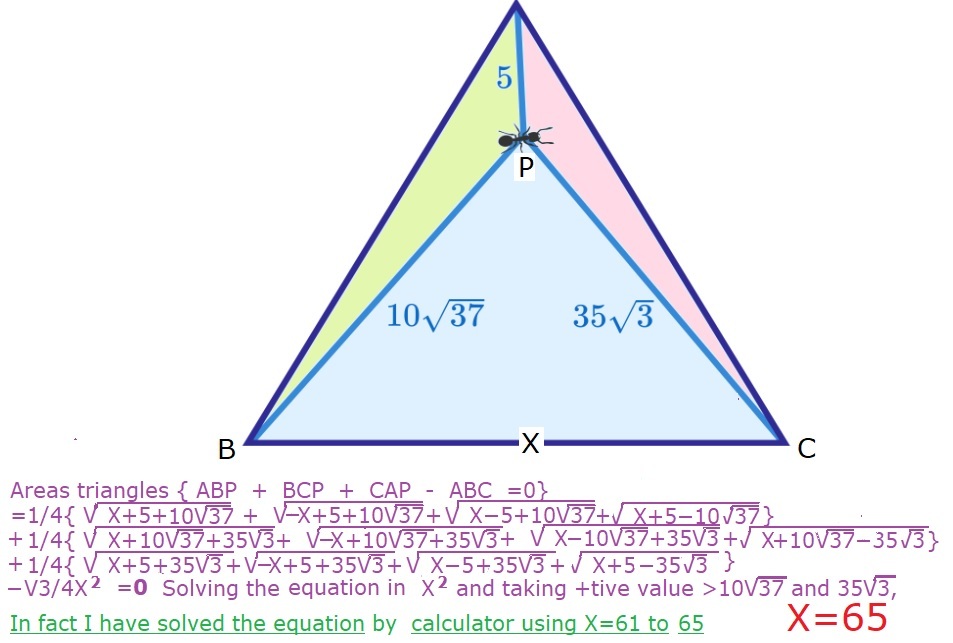
Assume x as the side.
Write Hero's formula for the areas of the three tangles.
Sum of this three formulas = $\dfrac{\sqrt{3}}4*x^2$ .
Solving x=65 is only greater than $10*\sqrt{35},~ and~35*\sqrt3$ .

Let $x$ be the length of one side of the equilateral triangle.

Let $\theta$ be the angle between sides of length $5$ and $35\sqrt{3}$ .

By rule of cosines, we have,

$x^2=5^2+(35\sqrt{3})^2-2\times 35\sqrt{3}\times 5\times \cos{\theta}$

We have that the three given lengths together form the side of a right-angled triangle, Since

$5^2+(35\sqrt{3})^2=(10\sqrt{37})^2$ .

Therefore in the new triangle formed from the Inspiration Problem we have that the angle between sides of length $5$ and $35\sqrt{3}=90^{\circ}$ . This implies (from answer to Inspiration Problem ), that $\theta=150^{\circ}$

Hence the first equation yields $x^2=5^2+(35\sqrt{3})^2-2\times 35\sqrt{3}\times 5\times \cos{150^{\circ}}=25+3675-2\times 35\sqrt{3}\times 5\times(-\cos(30^{\circ}))=25+3675+525=4225$

$\implies x=65$

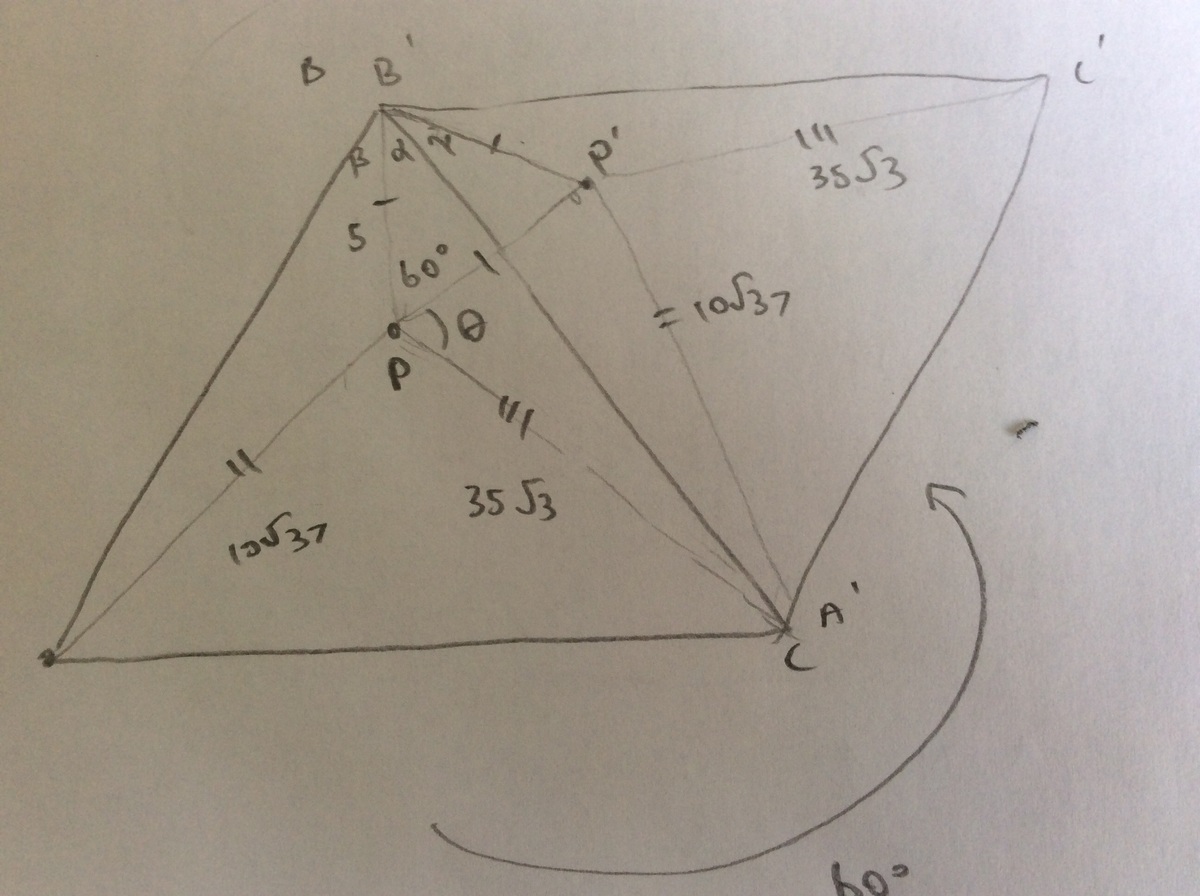
Consider the original triangle ABC, rotate it counter clockwise 60 degrees to form the corresponding points A'B'C' Now γ = β upon rotation, so α + γ = α + β = 60° From which we can deduce PP'B is an equilateral triangle so PP' = BP = 5 Using the Cosine Rule, ∠BPP' turns out to be 90° so ∠BPC = 150° and the length of a side of the equilateral triangle BC = 65 from the Cosine Rule.

This is an inspiration from a Coffin problem

Draw triangle $AFC \cong AEB$ ,

$\angle EAF = \angle EAC + \angle CAF = \angle EAC + \angle BAE =\angle BAC =60^\circ \rightarrow$ Triangle EAF is equilateral triangle. $EF=FA=AE=5$ units.

Noted that $EF^2+EC^2=FC^2$ , by converse of Pythagorean theorem, $\angle FEC = 90^\circ$

Using cosine rules, we have $AC^2 = AE^2+EC^2-2AE\cdot EC \cos(60^\circ+90^\circ)=4225$

hence $AC=65$

Apply Pythagoras theorem: Let a is horizontal distance form the vertical symmetry line, b is vertical distance from apex to Brilli. ((1/2)x + a)^2 + ((1/2)x√3 – b)^2 = (35√3)^2, ...........(1) ((1/2)x - a)^2 + ((1/2)x√3 – b)^2 = (10√37)^2, ...........(2) a^2 + b^2 = 5^2. ...........(3), Substract eqs(1) by eqs(2), we get 2xa = -25, or a = (-25)/(2x) , Add eqs(1) to eqs(2), we get 2x^2 + 50 - 2√3xb = 7375, or b = - (7325-2x^2)/(2√3x)
Substitute a, and b to eqs(3) and simplify, then we get x^4 -7400 x^2+ 13414375 = 0, then x^2= (7400±1050)/2 , or x=65