Trapezium 2

Construction: Drop a perpendicular from $B$ to line $AD$ and let $F$ be the foot of the perpendicular. So, $BF=h$ is the height of the trapezium.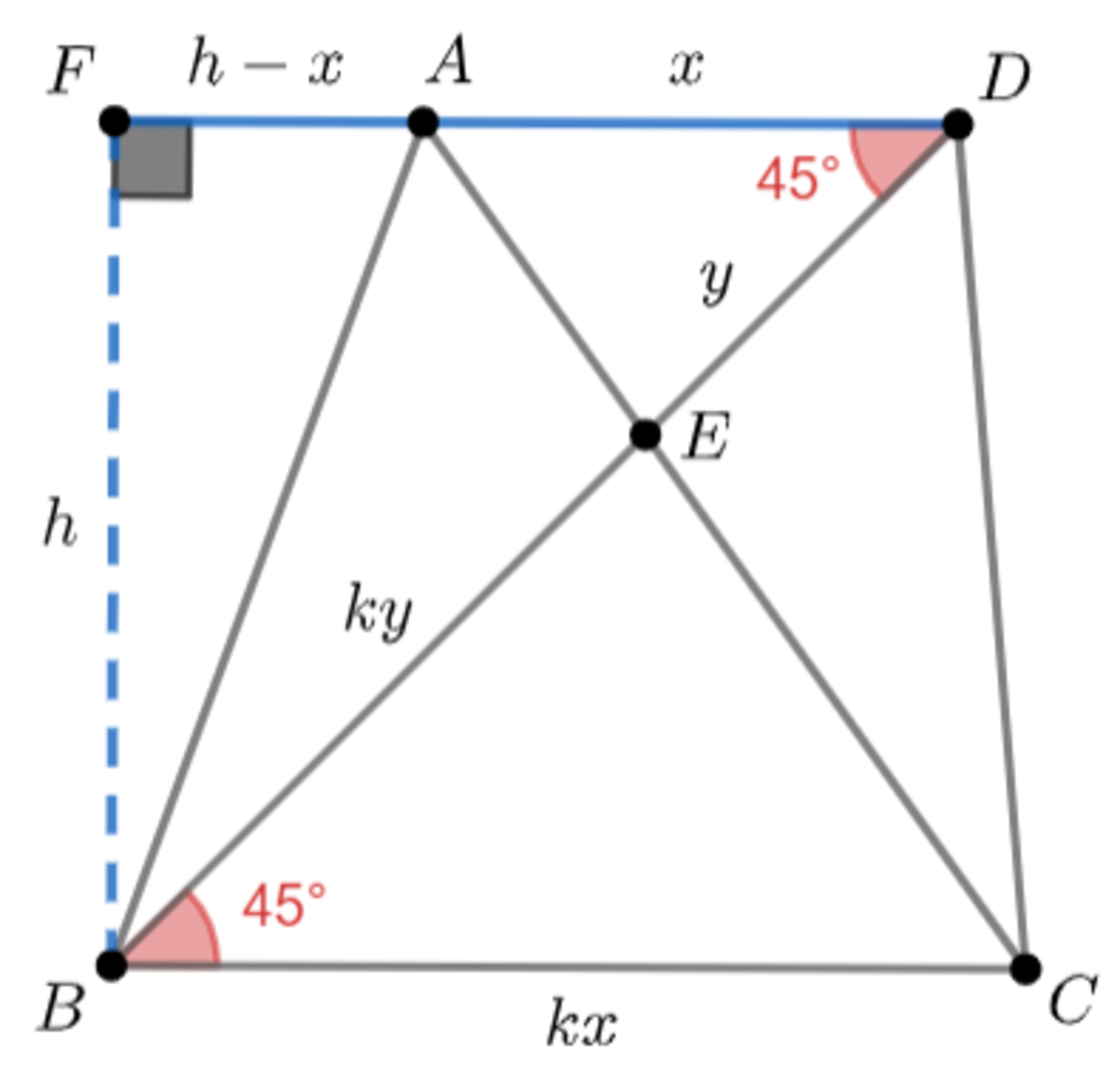 Since $\triangle ADE\sim \triangle CBE$ , we have, $\dfrac{CB}{AD}=\dfrac{BE}{DE}=k$ , for some constant $k$ . Let $AD= x\implies CB=kx\;$ and $\;DE=y\implies BE=ky$ .

We are given that, $\begin{aligned} AD+DB+BC&=35\\ x(k+1)+y(k+1)&=35 &&\;\;\;\;\;\;(1) \\ \\ \frac{1}{2}\cdot (AD+CB)\cdot BF&=75\sqrt{2} \\ x(k+1)\cdot h&=150\sqrt{2} &&\;\;\;\;\;\;(2) \\ \\ AD+BC&>BD \\ x(k+1)&>y(k+1) &&\;\;\;\;\;\; (3) \end{aligned}$ Note that $\triangle BFD$ is an isosceles right-triangle, so $\begin{aligned} DB^2&=BF^2+FD^2 \\ y(k+1)&=\sqrt{h^2+h^2} \\ y(k+1)&=h\sqrt{2} \end{aligned}$ Substituting this in $(1)$ and $(2)$ , defining $z=x(k+1)$ , we have the system of equations, $\begin{cases} z+h\sqrt{2}=35 \\ zh=150 \end{cases}$ Solving the above equations results in a quadratic with solutions $(h,z)=\left(\dfrac{15 \sqrt{2}}{2}, 20\right)\; \text{or} \;(h,z)=\left(10\sqrt{2}, 15\right)$ If $z=x(k+1)=15$ , from equation $(1)$ , $\;y(k+1)=20$ which contradicts equation $(3)$ . Thus, the second solution is invalid.

Therefore, the height of the trapezium is $h=\dfrac{15}{2}\sqrt{2} \implies l+m+n=15+2+2=\boxed{19}$