Triangle and a semicircle

The area of triangle ABC can be obtained from the sides and the semi-perimeter $s$ using Heron's formula:

$A=\sqrt{s(s-12)(s-18)(s-25)}=\frac{5\sqrt{6479}}{4}$

This is equal to the sum of the areas of $\triangle AOB$ and $\triangle BOC$ which are:

$A_{AOB}=\frac{12R}{2}=6R$ ... This comes from the base 12 and the height perpendicular to it,which is the radius $R$ of the half circle.

$A_{BOC}=\frac{18R}{2}=9R$ ... Calculated from the base 18 and the height, which is again the radius $R$ .

Equation $6R+9R=\frac{5\sqrt{6479}}{4}$ has a solution $R=\frac{\sqrt{6479}}{12}$

Area of the half circle is $A_{hc}=\frac{\pi}{2}\times\frac{6479}{12^2}$

So the area inside the triangle but outside the half circle is $A=A_{ABC}-A_{hc}=\frac{5\sqrt{6479}}{4}-\frac{6479\pi}{288}=\frac{360\sqrt{6479}-6479\pi}{288}$

$a+b=360+288=\boxed{648}$

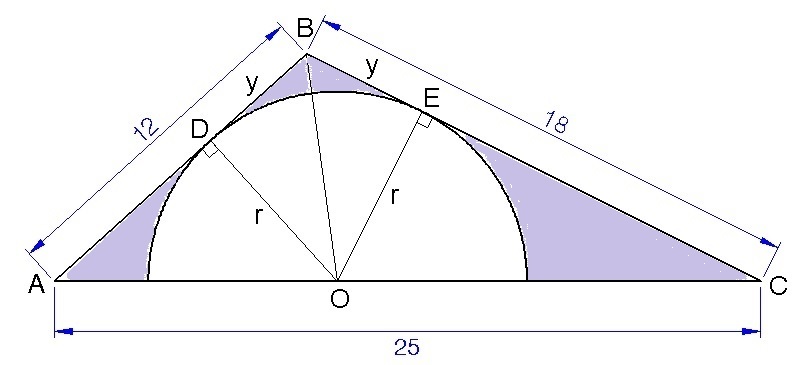
Using @Marvin Kalngan's figure above, where $OD$ and $OE$ are radius $r$ from the centre $O$ to the points of contact of $AB$ and $BC$ respectively. We note that:

$\begin{cases} \sin A = \dfrac r{AO} & \implies AO = \dfrac r{\sin A} \\ \sin C = \dfrac r{CO} & \implies CO = \dfrac r{\sin C} \end{cases}$ .

From cosine rule , we have:

$\begin{aligned} 18^2 & = 12^2+25^2 - 2(12)(25)\cos A \\ \cos A & = \frac {12^2+25^2 - 18^2}{2(12)(25)} = \frac {89}{120} \\ \implies \sin A & = \frac {\sqrt{6479}}{120} \end{aligned}$

From sine rule , we have:

$\begin{aligned} \frac {\sin C}{AB} & = \frac {\sin A}{BC} \\ \implies \sin C & = \frac {AB \cdot \sin A}{BC} = \frac {12}{18}\cdot \frac {\sqrt{6479}}{120} = \frac {\sqrt{6479}}{180} \end{aligned}$

Now we have:

$\begin{aligned} AO + CO = AC & = 25 \\ \implies \frac r{\sin A} + \frac r{\sin C} & = 25 \\ r\left(\frac {120}{\sqrt{6479}} + \frac {180}{\sqrt{6479}} \right) & = 25 \\ \implies r & = \frac {\sqrt{6479}}{12} \end{aligned}$

The area of shaded region is equal to the area of $\triangle ABC$ less that of the semicircle:

$\begin{aligned} A & = \frac 12 AB\cdot AC \cdot \sin A - \frac {\pi r^2}2 \\ & = \frac {12\cdot 25}2 \cdot \frac {\sqrt{6479}}{120} - \frac \pi 2 \cdot \frac {6479}{144} \\ & = \frac {360\sqrt{6479} - 6479 \pi}{288} \end{aligned}$

$\implies a+b = 360+288 = \boxed{648}$ .

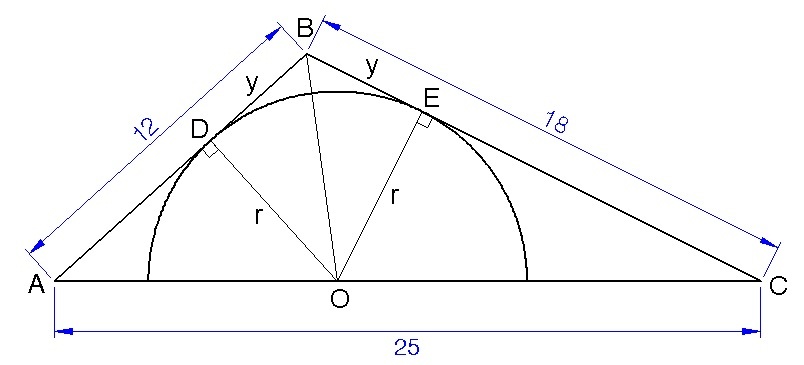Draw $OD$ and $OE$ to the points of contact of tangents $AB$ and $BC$ , respectively. Let $DB=BE=y$ , $r$ = radius of the semicircle, $A$ = area of the region inside the triangle but outside the semicircle, $A_T$ = area of the triangle and $A_C$ = area of the semicircle

In $\triangle ABC$ , $BO$ bisects $\angle B$ so that $\dfrac{AB}{AO}=\dfrac{CB}{CO}$ ,

then,

$\dfrac{12}{AO}=\dfrac{18}{25-AO}$

$12(25-AO)=18(AO)$

$300-12(AO)=18(AO)$

$300=30(AO)$

$10=AO$

It follows that,

$OC=25-AO=25-10=15$

consider $\triangle ADO$ , by Pythagorean Theorem , we have

$10^2=r^2+(12-y)^2$

$-44+24y-y^2=r^2$ $\color{#D61F06}(1)$

consider $\triangle CEO$ , by Pythagorean Theorem , we have

$15^2=r^2+(18-y)^2$

$-99+36y-y^2=r^2$ $\color{#D61F06}(2)$

equate $\color{#D61F06}(1)$ and $\color{#D61F06}(2)$

$-44+24y-y^2=-99+36y-y^2$

$55=12y$

$\dfrac{55}{12}=y$

Substitute the above in $\color{#D61F06}(1)$ or $\color{#D61F06}(2)$

$r^2-(\dfrac{55}{12})^2+24(\dfrac{55}{12})-44$

$r^2=\dfrac{6479}{144}$

$r=\sqrt{\dfrac{6479}{144}}$

solving for the area of the semicircle

$A_c=\dfrac{\pi}{2}r^2=\dfrac{6479\pi}{288}$

solving for the area of the triangle by Heron's Formula

$s=\dfrac{12+18+25}{2}=\dfrac{55}{2}$

$s-12=\dfrac{31}{2}$

$s-18=\dfrac{19}{2}$

$s-25=\dfrac{5}{2}$

Substituting, we obtain

$A_T=\sqrt{(\dfrac{55}{2})(\dfrac{31}{2})(\dfrac{19}{2})(\dfrac{5}{2})}=\sqrt{\dfrac{161975}{16}}$

$A_T=\dfrac{5}{4}\sqrt{6479}$

Finally,

$A=A_T-A_C=\dfrac{5}{4}\sqrt{6479}-\dfrac{6479\pi}{288}=\dfrac{360\sqrt{6479}-6479\pi}{288}$

It follows that,

$a=360$ and $b=288$

so

$a+b=360+288=648$

Sorry. I did not see Mr. Marta Reece's solution. I saw it now. It is almost the same.