Triangle, Circles, Angles

Level 2

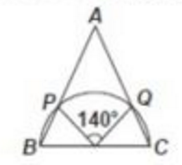
B C BC is the diameter of a semi-circle. The sides of A B AB and A C AC of a triangle A B C ABC meet the semi-circle in P P and Q Q respectively. P Q PQ subtends 14 0 ∘ 140^\circ at the centre of the semi-circle. Then ∠ A \angle A is equal to:

(A) 1 0 ∘ 10^\circ .
(B) 2 0 ∘ 20^\circ .
(C) 3 0 ∘ 30^\circ .
(D) 4 0 ∘ 40^\circ .

20 40 30 10

Amish Garg by Amish Garg , 20, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Sahar Bano
Apr 7, 2020

Let the centre of the semi circle be O

Now, as angle QOC=POB and POB+QOC+POQ=180°

=>QOC=(180°-140°)/2 = 20° In ∆QOC angle OQC = OCQ (Because opposite side OQ and OC are equal)

Also OQC + OCQ + QOC = 180°

=>OQC = (180° - QOC)/2 = 80°

Now as angle OQC + OQA = 180°

=> OQA = 180° - 80° = 100°

Now in quadrilateral OQAP, OQA+QAP+APQ+POQ=360°

=>2(OQA) + 140° + QAP = 360°. (OQA = OPA and POQ=140°)

=>QAP= 360°-200°-140°=20°

As QAP = A

Hence the answer is 20°

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Drex Beckman
Jan 28, 2016

The line BC op course forms a 180 degree angle. 180-140/2=20. By definition, there are two isoceles triangles formed opposite the 140 angle. These have one angle of 20 degrees, so we divide 180-20 by two to get 80. We can find the final angle now: 180-(80+80)=20.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...