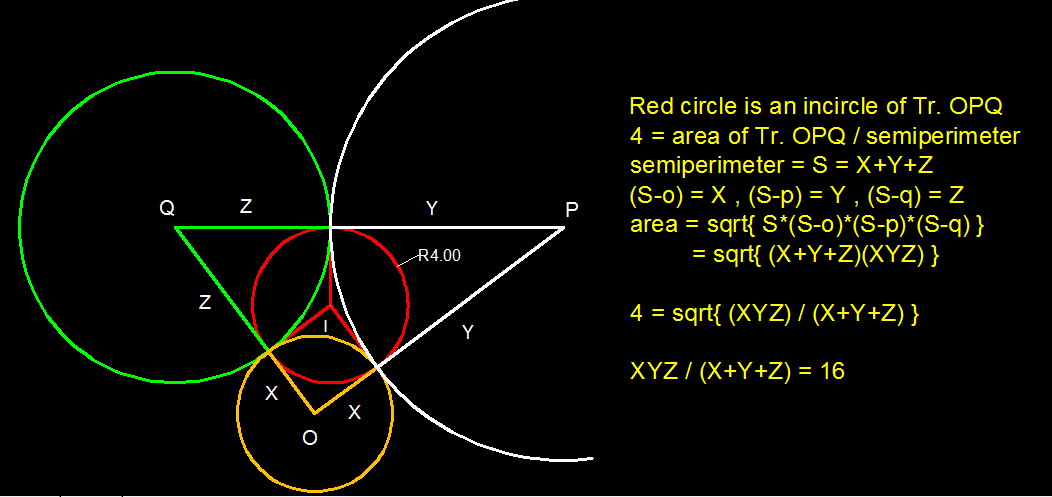
Triangle inside circles

Geometry Level 5

Three circles touch one another externally. The tangents at their points of contact meet at a point whose distance from a point of contact is 4. Find the ratio of the product of the radii to the sum of radii of circles, if your answer is in the form of r R \dfrac{r}{R} , where r r and R R are coprime positive integers, submit your as r + R r+R .


The answer is 17.

Atul Shivam by Atul Shivam , 23, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Ahmad Saad
Jan 24, 2016

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Deepak Kumar
Jan 20, 2016

For quick solving,the three circles may be taken as equal circles i.e of equal radii forming equilateral triangle by joining the centers.The inradius of the triangle will be 4.From here it is easy to proceed even without use of pen and paper.

2 Helpful 0 Interesting 0 Brilliant 0 Confused

but it may not be always true

Atul Shivam - 5 years, 4 months ago

Log in to reply

It's always true, else you need to accept multiple answers to the same question.

Saya Suka - 1 month ago

Then try to solve for circles being of different radii.In the end you will get a relation in terms of the radii . That relation is symmetric in terms and that's why considering special case of equal radii works here.

Deepak Kumar - 5 years, 4 months ago

tera bap ka lora 7-2 hein

Super Ampharos - 2 years, 1 month ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...