Triangle Triangle Triangle
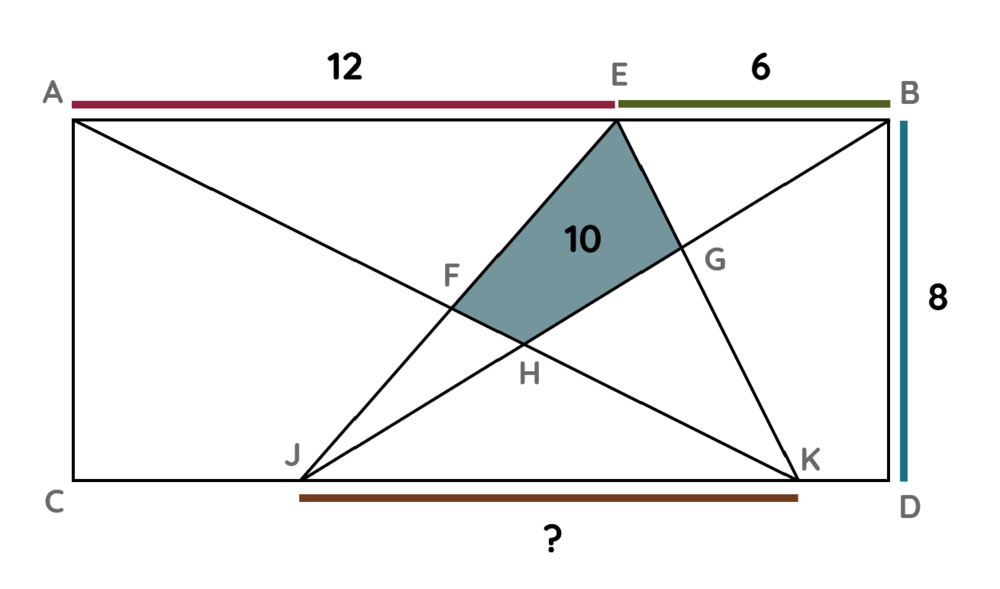
Rectangle A B D C has width 1 8 and height 8 . A point E is chosen such that ∣ A E ∣ = 1 2 and ∣ E B ∣ = 6 . Points J and K are chosen such that the area of quadrilateral E F H G is 1 0 . Find the length of line J K .
The answer is 6.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
We note that the area of quadrilateral E F G H is given by:
[ E F G H ] = [ J E K ] − [ J F K ] − [ J G K ] + [ J H K ]
Let ∣ J K ∣ = x . Then [ J E K ] = 2 1 ⋅ x ⋅ 8 = 4 x . For [ J F K ] , let the altitude of △ J E K be h . Since △ J F K and △ A F E are similar, then 8 − x h = 1 2 x ⟹ h = x + 1 2 8 x and [ J F K ] = 2 x h = x + 1 2 4 x 2 . Similarly, [ J G K ] = x + 6 4 x 2 and [ J H K ] = x + 1 8 4 x 2 . Then we have:
4 x − x + 1 2 4 x 2 − x + 6 4 x 2 + x + 1 8 4 x 2 = 1 0
Solving for x , we get ∣ J K ∣ = x = 6 .
Let J K = x . Triangles Δ A H B and Δ J H K are similar, and their heights add up to 8 . Say the perpendicular distance from H to A B is h (the height of Δ A H B ). Since they're similar, the ratio of their heights is the same as the ratio of their bases: h 8 − h = 1 8 x
Rearranging, we find h = x + 1 8 8 × 1 8
so the area of Δ A H B is Δ A H B = 2 1 × 1 8 × h = x + 1 8 4 × 1 8 2
In the say way, we can find Δ A F E = x + 1 2 4 × 1 2 2 and Δ B G E = x + 6 4 × 6 2
We can write the area of E F H G in terms of these triangles: [ E F H G ] = Δ A H B − Δ A F E − Δ B G E
that is, 1 0 = x + 1 8 4 × 1 8 2 − x + 1 2 4 × 1 2 2 − x + 6 4 × 6 2
Cross-multiplying and tidying leads to a cubic in x ; the only root in the interval 0 < x < 1 8 is x = 6 .