Triangle with an Altitude
A B C is a right triangle with ∠ A B C = 9 0 ∘ . Point D is the midpoint of B C , and point E is the foot of the perpendicular from B to A C . A D and B E intersect at F . If A B = 3 6 and ∠ B A D = ∠ B C A , what is the value of F E 2 ?
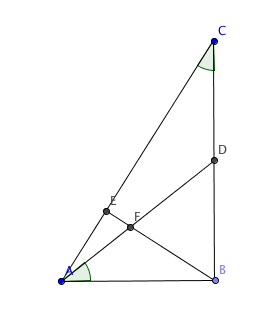
The answer is 54.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
22 solutions
2nd line. Should it be angle BFD or angle FBD?
Log in to reply
Oh, yes, you're correct. Sorry about that. It should actually say ∠ F B D = ∠ B D F = 2 π − θ .
in the third line by pythagorus AD=18√6 but AD should also be equals to 2x (AD=AF+FD=x+x=2x) hence the value should be 36√2. ???????
First of all we have to name the angles.
Angles with x value wil be <ACB < DAB <BFA.
Let y denote 90 - x. So <BAC < ADB <FBD have value y.
Since ADB and ACB have the same angles, we can assume they are similar, then so:
D B A B = B A B C
Since D is the midpoint of BC we get:
D B 3 6 = 3 6 2 D B
So DB = 6 4 8
By Pytagoras we get that:
A B 2 + D B 2 = A D 2
So AD = 1 9 4 4
Searching new similar triangles we see that AFB and BFD are isoseles. We also realize that:
AF = FB = FD.
Since AD = AF + FD, we get:
1 9 4 4 = 2 * AF, and so:
AF = 2 1 9 4 4
So we have the hypotenuse of AEF, and we have to search one the other sides.
Since AEB and ABD are similar we can apply Thales in order to find AE.
6 4 8 1 9 4 4 = A E 3 6
Hence AE = 1 9 4 4 3 6 6 4 8
And finally we use Pytaoras to find EF
E F 2 = A F 2 − A E 2
E F 2 = 4 8 6 − 4 3 2 = 5 4
SOLVED
U have explained it in the simplest way. thanxxxxxxx 4 dat
Let ∠ D A B = θ ⇒ B D = 3 6 tan θ ⇒ B C = 7 2 tan θ .
From the triangle A B C , tan θ = 7 2 tan θ 3 6 ⇒ = 2 1
Plot the coordinates on the cartesian plane with A ( 0 , 0 ) , B ( 3 6 , 0 ) , C ( 3 6 , 7 2 tan θ )
Then equation of straight line A C is y = 2 tan θ x = x 2
And equation of straight line A D is y = tan θ x = x 2 1
m B E = − m A C 1 = − 2 1
Equation of B E is y = − 2 1 ( x − 3 6 )
Comparing straight lines A C and B E , we get E ( 1 2 , 1 2 2 )
Comparing straight lines A C and B E , we get F ( 1 8 , 9 2 )
Hence, E F 2 = ( 1 2 − 1 8 ) 2 + ( 1 2 2 − 9 2 ) 2 = 5 4
please can u tell how did u get coordinates of E and F ??
Log in to reply
To solve for E , find the intersection points of straight line A C and B E . Which is solve y = x 2 , y = − 2 1 ( x − 3 6 ) . Apply the same logic to coordinate F .
It's DAMN COOL!!!!!!
Let
∠
A
C
B
=
∠
B
A
D
=
α
and
B
C
=
h
Of
Δ
ABC,
t
a
n
α
=
B
C
A
B
=
h
3
6
Of
Δ
ABD,
t
a
n
α
=
A
B
D
B
=
3
6
2
h
[
D
is the midpoint of
B
C
]
⇒
h
3
6
=
3
6
2
h
⇒
h
2
=
2
.
3
6
2
⇒
h
=
3
6
2
Of right triangle
A
B
C
, by Pythagorean Theorem,
A
C
=
A
B
2
+
B
C
2
⇒
A
C
=
3
6
2
+
h
2
⇒
A
C
=
3
6
2
+
2
.
3
6
2
⇒
A
C
=
3
6
3
Δ
A
B
C
and
Δ
A
B
E
are similar triangles, so,
∠
A
C
B
=
∠
A
B
E
⇒
∠
B
A
D
=
∠
A
B
E
[
∠
A
C
B
=
∠
B
A
D
, Given ]
So, of
Δ
F
A
B
;
F
B
=
F
A
We know that,
Δ
A
B
C
and
Δ
A
B
E
are similar triangles, so,
A
B
A
C
=
A
E
A
B
⇒
3
6
3
6
3
=
A
E
3
6
⇒
A
E
=
3
3
6
⇒
A
E
2
=
3
3
6
2
Of
Δ
B
C
E
,
A
D
is the transversal, so by MENELAUS THEOREM,
D
C
B
D
.
A
E
A
C
.
F
B
E
F
=
1
⇒
1
1
.
3
3
6
3
6
3
.
F
A
E
F
=
1
[
D
is the midpoint of
B
C
, and
F
B
=
F
A
]
⇒
F
A
=
3
E
F
OF right triangle
F
E
A
, by Pythagorean Theorem,
F
A
2
=
E
F
2
+
A
E
2
⇒
(
3
E
F
)
2
=
E
F
2
+
3
3
6
2
⇒
8
E
F
2
=
3
3
6
2
⇒
E
F
2
=
5
4
[ANSWER]
Let B D = x → C D = x . We note that △ A B C ∼ △ D B A by Angle-Angle similarity. This means that B D A B = A B B C → x 3 6 = 3 6 2 x → x = 1 8 2 . By the Pythagorean Theorem on △ A B C , A B 2 + B C 2 = A C 2 → 3 6 2 + ( 2 ⋅ 1 8 2 ) 2 = A C 2 → A C = 3 6 3 .
We now note that △ A B C ∼ △ B E C , which means that A B A C = E B B C → 3 6 3 6 3 = E B 3 6 2 → E B = 3 6 3 2 . Using the Pythagorean Theorem on △ E B C , we have E C 2 = B C 2 − E B 2 → E C 2 = 3 6 2 ( 2 − 3 2 ) → E C = 2 4 3 , and then A E = 3 6 3 − 2 4 3 = 1 2 3 . Noting that E C = 2 ⋅ A E , we proceed to calculate E F by using Mass Points.
Assign point B a mass of 1 . Then the mass of C must also be 1 and the mass of A is 2 , which means that the mass of E is 3 . Thus, the ratio E F : F B = 1 : 3 → E F = 4 1 ⋅ E B = 4 1 ⋅ 3 6 3 2 = 9 3 2 . We square this value to get the desired value, E F 2 , which is ( 9 3 2 ) 2 = 8 1 ⋅ 3 2 = 5 4 .
given the facts above, we can say that triangles ABC, DAB, BAE, and FAE are congruent with θ : 36 90 - t h e t a : 36 90 : 36 90 : 36 ratios respectively..
through these, it is obvious that it is a 30-60-90 angled triangles..
in the figure above, we are finding the value of F E 2 of triangle BAE = FAE and as it is written, 90 : 36, which means BA = 36, AE = 18 and EB = 18 s q r t 3
thus, FA = 36 AE = 18 and EF = 18 s q r t 3
we are finding for the F E 2 so [18\( \ sqrt{3} ]^{2} ) = 54
From ∠ B A D = ∠ B C A we get that △ B C A ∼ △ B A D . Hence, B D A B = B A B C → A B 2 = B C ∗ B D ∠ C A B = ∠ A D B ∠ B A C = ∠ B D A Since D is a median, B C = 2 B D . So 1 2 9 6 = 2 B D 2 → B D = 1 8 2 and B C = 3 6 2 . Using ∠ A C B = ∠ E C B , we get that △ B C A ∼ △ B A D ∼ △ E C B . Hence, ∠ E B C = ∠ B D A → F D = F B . Now, ∠ B A C + ∠ B C A = 9 0 ∘ ∠ E B C + ∠ F B A = 9 0 ∘ ∠ B A C + ∠ B C A = ∠ E B C + ∠ F B A ∠ B A C + ∠ B C A = ∠ B D A + ∠ F B A ∠ B C A = ∠ F B A → ∠ B A D = ∠ F B A → A F = F B = F D Now using the Pythagorean Theorem, we get that A D 2 = D B 2 + A B 2 = 3 6 2 + 1 8 2 2 = 1 9 4 4 → A D = 1 9 4 4 → F B = 4 8 6 = 9 6 . In addition, we apply the pythagorean theorem to △ A B C to get A C 2 = A B 2 + B C 2 = 3 8 8 8 → A C = 3 6 3 Let x = A E , now using the geometric mean of E C and A E , we get: x ( 3 6 3 ) = 3 6 2 → x = A E = 1 2 3 Finally we apply the pythagorean theorem to △ A E B and △ A B D and get: A E 2 + E B 2 = A B 2 ( 1 2 3 ) 2 + ( F E + F B ) 2 = A B 2 F E 2 + 2 F E ∗ F B + F B 2 = 8 6 4 and A D 2 = A B 2 + D B 2 ( A F + F D ) 2 = 1 9 4 4 A F 2 + 2 A F ∗ F D + F D 2 = 1 9 4 4 Subtracting these two equations gives us. A F 2 − F E 2 + 2 F D ( A F − E F ) = 1 0 8 0 . Now, A F 2 − F E 2 = 4 3 2 and F D = A F = 9 6 . Substituting these two values in we get: − 1 8 6 F E = − 3 2 4 F E = 6 1 8 = 3 6 . So F E 2 = 5 4
You use a ruler and a protractor :D jk. First, you mark ∠ABE=∠BAD=∠ACB because ΔAEB~ΔBEC~ΔDBA~ΔABC. Using proportions, AB/BC=BD/AB. Since BD=CD, replace the proportion with 36/2(BD)=BD/36. Using cross multiplication, BD=18√2 and BC=36√2.Using pythag, AC=36√3. Using proportions again, AB/AC=AE/AB so 36/36√3=AE/36 and AE=12√3. Using pythag again, EB=12√6. Since ΔAFB is isosceles, AF=BF. Call EF x and BF 12√6-x. Replace BF with AF and use pythag. You end up with x=3√6 so EF^2=54
yay 1 up vote lol
Using AA similarity, triangle CAB is similar to triangle ADB, so AB/CB = DB/AB meaning that (AB)^2 = (CB)(DB). We know that AB = 36, and since D is the midpoint of CB, CB = 2DB. Thus, we have 36^2 = 2(DB)^2, so DB = 18sqrt(2).
We use Pythagorean to find that AC = 36sqrt(2). And since [ABC] = (AB)(BC)/2 = 36 * 36sqrt(2)/2 = (AC)(BE)/2 = (BE) * 36sqrt(3)/2, we know that BE = 12sqrt(6). Applying Pythagorean on triangle ABE, we find that AE = 12sqrt(3), and since AE + EC = 36sqrt(3), EC = 24sqrt(3).
The ratios of AE:EC = 1:2 and BD:DC = 1:1. Using mass points, we assign A a mass of 2, B a mass of 1, and C a mass of 1. Mass E = Mass A + Mass C = 2 + 1 = 3. Mass F = Mass B + Mass E = 1+3 = 4. From this, we see that the ratio FE:BE = 1:4.
Hence, FE = (BE)/4 = (12sqrt(6))/4 = 3sqrt(6), so (FE)^2 = 54.
Let A = { 0 , 0 } and B = { 3 6 , 9 } .
ADB and CAB are similar triangles as all angles are similar. Hence,
2 ∣ BD ∣ 3 6 = 3 6 ∣ BD ∣ ∣ BD ∣ = 1 8 2 D = { 3 6 , 1 8 2 }
Since ∠ A B C = 9 0 ∘ , C = { 3 6 , 3 6 2 } .
E is the foot of the perpendicular from B to AC. It must lie on AC.
E = λ { 3 6 , 3 6 2 } E ⋅ { 3 6 , 3 6 2 } = 0 E = { 1 2 , 1 2 2 }
F must lie on both AE and BE.
F = λ 2 { 3 6 , 1 8 2 } = { 3 6 , 0 } + μ { 1 2 − 3 6 , 1 2 2 } F = { 1 8 , 9 2 }
Hence,
∣ FE ∣ = ( 1 8 − 1 2 ) 2 + ( 9 2 − 1 2 2 ) 2 = 3 6 ∣ ∣ FE ∣ 2 = 5 4
Let ∠BAD=∠BCA = alpha
Since tan (alpha) = BD/ AB = AB /BC
then 1/2 BC^2 = AB^2
==> BC =AB*sqrt(2)
tan (alpha) =1/ sqrt(2) ==> alpha = 35.26 degrees
AC^2 = 36^2 + 36^2 (2) ==> AC = 36*sqrt(3)
AE . AC =AB^2 ==> AE =12 sqrt(3)
Use the triangle AEF to solve for FE
with angle FAE = beta
beta can be deduced from 2alpha +beta = 90 deg
Finally FE = 7.348...
and FE^2 = 54
We are given that angle ∠ B A D ≅ ∠ A C B . Therefore we should look for similar triangles. Right off the bat, we see that △ A B D ≅ △ C B A . This means that B D A B = A B C B . We know that A B = 3 6 , and B D = 2 C B . Solving, we get C D = B D = 1 8 2 and C B = 3 6 2 . Using the Pythagorean Theorem, we can find the hypotenuse, which is A C = 3 6 3 . We can find E B using area: 2 1 A C ∗ E B = 2 1 A B ∗ B C . Solving for E B , we get E B = 1 2 6 .
We can then solve for A E and E C . Applying the Pythagorean Theorem again with sides A E , E B , A B , we get A E = 1 2 3 ⟹ E C = 2 4 3 .
Draw the perpendicular from point D to side A C and call it I . We notice that △ C I D △ C E B . We can also see that D is the midpoint, to the ratio of the sides is 1 / 2 . Therefore, I D = 6 6 . Apply the Pythagorean Theorem again to △ C I D to get I C . I C = 1 2 3 . This implies that A E = E I = I C , which means the ratio of the sides of A E F to A E D is 1 / 2 . Therefore, F E = 3 6 ⟹ F E 2 = 5 4 .
Let B C = a . Then, B D = C D = 2 a . First, notice, from AA similarity, that △ B A D ∼ △ B C A . From this, we can deduce the following: B D B A = B A B C ⟹ 2 a 3 6 = 3 6 a ⟹ a = 3 6 2 . Now, we use a coordinate system. Note that this is different than that depicted in the problem diagram. The problem can be done in a similar manner using an equally effective coordinate system.
Let C be the point ( 0 , 0 ) . Let B be the point 3 6 2 , 0 . It follows that A is the point 3 6 2 , 3 6 ) , and D is the point ( 1 8 2 , 0 ) .
This gives us that the equation of line D A is y = 2 x − 3 6 and the equation of line E B is y = − 2 x + 7 2 .
Note that F is the intersection of these two lines. We find its coordinates. − 2 x + 7 2 2 2 x 2 x x = = = = 2 x − 3 6 1 0 8 5 4 2 7 2 . Thus, F is the point ( 2 7 2 , 1 8 ) . Finally, we need to find F E . To do this, note that F E = E B − F B and that E B is an altitude. So we can find it. We have equality of areas: 2 E B ⋅ A C = 2 A B ⋅ B C ⟹ 3 6 ⋅ 3 6 2 = E B ⋅ 3 6 3 . Solving for E B , we get 6 3 6 = 6 6 . Finally, we use the Distance Formula to find F B and we get 9 6 .
We obtain ∣ E B ∣ = 3 6 . A negative length may result due to the inversion of a coordinate system, but our answer is perfectly symmetric otherwise. We get F E = 3 6 , so F E 2 = 5 4 .
From similarity between triangles ABD and CBA results BC=36 2 , next AC=36 3 and BE=12 6 . The triangle FBD is isosceles, let G belong [BD] so that FG is height (altitude) and median. But, if G is the middle of [BD], F will be the middle [AD] (FG and AB are parallel lines, Reciprocal Theorem) and FG=18. In triangle FGB, FB=9 6 that implies EF=3 6 . Finally, EF 2 =54 :)
Let BD = CD = a and Angle BCA=Angle BAD = C
Given that AB = 36
Now since Triangle ABC and DBA are similar triangles
Therefore, AB/BD=BC/AB
Here AB = 36, BC = 2a and BD = a
Therefore, BC = 36√2
Tan C = 36/(36√2)= 1/√2
Sin C = 1/√3, Cos C = √2/√3
From triangle ABC and ABE, angle ABE = C, angle FAE = 90 -2C
Therefore AE = 36 Sin C
From triangle FAE, FE = (18 Cos 2C)/(Cos C) = 3√6
Hence, FE^2 = 54
As the hypothesis, ∠ B A D = ∠ B C A and ∠ A B C = 9 0 ∘ . Thus:
tan ∠ B A D = tan ∠ B C A
⇔ A B B D = B C A B
D is the midpoint of B C , this implies that B C = 2 B D . Thus:
B C = 2 B D = A B 2
We have ∠ B E A = ∠ A B C = 9 0 ∘ , thus ∠ E A B = ∠ A C B = ∠ B A D and Δ A F B is an isosceles triangle with A F = B F . In addition, ∠ A B D is a right angle, thus F is the midpoint of A D ( F is the center of the circle which pass A , B , D ), this implies:
B F = A F = 2 A D = 2 A B 2 + B D 2 = 4 6 A B
We also have:
sin ∠ B A C = A C B C = A B B E
⇔ B E = A C A B ⋅ B C = A B 2 + B C 2 A B ⋅ B C = 3 6 A B
Therefore, E F = B E − B F = 1 2 6 A B = 3 6 . And the final solution of the problem is E F 2 = 5 4 .
It is easy to see that A B C is similar to A E B . ∠ A E B = 9 0 ∘ and ∠ B C A = ∠ B C A . Since D is the midpoint of B C , we have: A B B D = 2 B D A B .
Plugging in 36 for A B , we can solve the ratio: B D = 1 8 2 .
Since A B C is similar to A E B , ∠ A B E = ∠ C ∴ ∠ A B E = ∠ B A D
∴ A B F is isosceles
Draw a perpendicular from F to A B . Call the intersection of the perpendiculars G . Since A B F is isosceles, F G also bisects A B , thus A G = B G = 1 8 . Also, A G F is similar to A B D ∴ B F = A F = 2 1 A D .
A D = A B 2 + B D 2 = 1 8 6 ∴ B F = 9 6
A E B can be easily shown to be similar to D B A . Thus, we have the ratio A D A B = A B E B , which we can solve to find E B = 1 2 6 .
E F = E B − B F = 1 2 6 − 9 6 = 3 6 ∴ E F 2 = 5 4
We have Δ A B D and Δ C B A are similar triangles, so A B 2 = B D . B C = 2 1 . B C 2
--> A B 2 = 2 1 B C 2 <=> B C = A B 2 --> A C = A B 3
We also have Δ A E B similar with Δ A B C => A B 2 = A E . A C
--> A E 2 = 3 1 A B 2
Then let α , β and θ be angle B A D , B A C and F A E respectively, from trigonometry we get t a n θ = 1 + tan β . tan α tan β − tan α = 2 2 − 2 2 = 4 2
For Δ A E F is a right triangle so F E 2 = A E 2 tan 2 θ = 3 1 A B 2 8 1 = 5 4
let, ∠ A C B = ∠ D A B = x . For △ A B C and △ A B D ,
t a n ( x ) = B C A B = A B B D
As , B D = 2 1 B C , t a n ( x ) = 2 1
for △ A B E , as ∠ B A E = 9 0 ∘ − x , ∠ A B E = x
Hence , ∠ E F A = 2 x , ∠ E A F = 9 0 ∘ − 2 x
Using sine rule in △ A E F ,
c o s ( 2 x ) E F = s i n ( 2 x ) A E
E F = c o t ( 2 x ) A E
A E = 3 1 A B
Also , t a n ( 2 x ) = 2 ( 1 / 2 ) / ( 1 − ( 1 / 2 ) ) = 2 2
Hence , A E 2 = 8 ∗ 3 3 6 ∗ 3 6 = 5 4
In triangles ADB and CAB,
Angle DAB = Angle ACB (Given)
Angle ABD = Angle CBA = 90 degree (Common angle)
So, triangles ADB and CAB are similar. (AA Similarity)
So, we have C B A B = A B D B which is equivalent to A B 2 = C B × D B
which is also equivalent to A B 2 = 2 1 C B 2
Through simple calculation, one will get CB = 36 2
By Pythagoras theorem, AC = 36 3 and AD = 18 6
Now observe that triangle CEB is a right angled triangle with angle CEB as 90 degree. This means that a circle with diameter CB can pass through the vertices of this triangle. AB will hence be the tangent to this circle at B.
So, by the Tangent Secant theorem, we have A B 2 = A E × A C .
This means that AE = 12 3
Now observe triangle AFB. From the earlier part, we have arrived at the conclusion that AB is the tangent to the circle with diameter CB. So by Tangent chord theorem, we have angle FBA = angle ECB = angle FAB. This means that triangle AFB is isosceles with AF = FB.
Now observe triangle FDB. We have angle FBD = 90 - angle FBA and angle ADB = 90 - angle FAB too. This means that triangle FDB is isosceles with FB = FD.
However, since we have shown that AF = FB, then AF = FD.
This will mean that AF = 9 6
Hence, by applying Pythagoras theorem again, one will get F E 2 = 54
Since ∠ B A D = ∠ B C A ⟹ ∠ B D A = ∠ B A C ( Using angle sum property in triangles ABD and ABC ) and ∠ A B D = ∠ C B A = 9 0 ∘ ⟹ △ A B D ∼ △ C B A .
⟹ B D A B = B A C B
⟹ B D = 1 8 2
Using Pythagorean theorem in △ A B C we find A C = 3 6 3 , and for a right angled triangle we obviously have A B × B C = A C × E B ⟹ E B = 3 6 3 2 .
Again using Pythagorean theorem in △ A E B we find A E = 3 3 6 .
Now ∠ B D A = ∠ B A C
⟹ tan ∠ B D A = tan ∠ B A C
⟹ B D A B = tan ( ∠ B A D + ∠ F A E )
⟹ 2 = 1 − 3 6 2 F E 3 3 6 F E 3 + 2 1
Simplifying out gives F E = 6 1 8 . So F E 2 = 5 4
First, we determine angles. Let ∠ D A B = α , then ∠ A D B = π / 2 − α , ∠ A D C = π / 2 + α , ∠ D A C = π / 2 − 2 α , and ∠ E B A = α . Hence the triangles A F B and B F D are both isosceles, with A F = B F = F D .
The rest can now be computed analytically. Let B = ( 0 , 0 ) , A = ( 3 6 , 0 ) , and C = ( 0 , x ) . Then D = ( 0 , x / 2 ) , and as F is the midpoint of A D , we have F = ( 1 8 , x / 4 ) . As B F ⊥ A C , their dot product must be zero, which gives x = 3 6 2 . Let E = A + p ⋅ A C = q ⋅ B F . This solves to p = 1 / 3 , q = 4 / 3 . Thus, E F = ( q − 1 ) ⋅ B F = ( 1 / 3 ) ⋅ ( 1 8 , 9 2 ) = ( 6 , 3 2 ) and therefore E F 2 = 6 2 + ( 3 2 ) 2 = 3 6 + 1 8 = 5 4 .
Let ∠ B A D = ∠ B C A = θ . Then, doing some angle-chasing, we see that ∠ A B F = θ as well, so A F = B F , and that ∠ B F D = ∠ B D F = 2 π − θ , so B F = D F . Thus, A F = B F = D F .
Now let B D = D C = x . Because △ A B D ∼ △ C B A , we have the equation B D A B = A B C B x 3 6 = 3 6 2 x Solving for x gives x = 1 8 2 . By Pythagoras on right triangle A B D , we have A D = 1 8 6 , so A F = B F = D F = 9 6 . Also, by Pythagoras on right triangle A B C , we have A C = 3 6 3 .
Notice that triangles A B C and B E C are similar, so we have E B C B = A B A C E B 3 6 2 = 3 6 3 6 3 Solving for E B gives E B = 1 2 6 . Thus, E F = E B − B F = 1 2 6 − 9 6 = 3 6 , and E F 2 = 5 4 .