Triangular Numbers 4 – Find A Formula
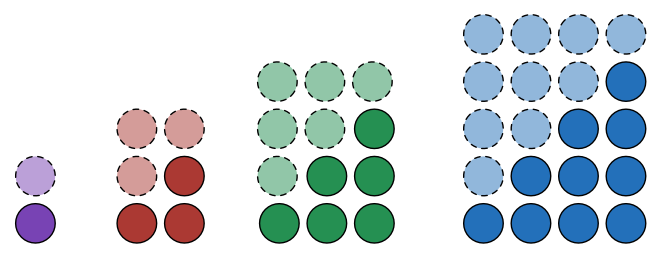 Which of the following gives us a general formula for the size of the
n
th
triangular number?
Which of the following gives us a general formula for the size of the
n
th
triangular number?
This problem is part of Arron's set What Makes A Number Triangular?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
7 solutions
Discussions for this problem are now closed
it's just like that...
Nailed it.
u all r right
We know that the rectangle formed from two of the th triangular numbers has dimensions . Thus, the size of just one of the triangular numbers is , or .
1,3,6,10..... now if we put the values of n in the equation n(n+1)/2 , the same sequence formed.
Observing the rows and columns of the rectangles , the series goes likes this 1-(1.2), 2-(2.3),3-(3.4),4(4.5),.........................n-{n(n+1)}.The half of the rectangle gives triangle(Those dark circles) so n(n+1)/2
The triangles in the pattern are formed by adding successive numbers.
So, the formula to find the number of dots in the n t h triangular number is:
= 2 n ( n + 1 ) (Formula to find sum of numbers from 1 to n )
So, the answer is: 2 n ( n + 1 )
The formula to derive the nth rectangular number is n(n+1). Now divide it by two and we get the general formula of the nth triangular number.
The formula to derive the nth rectangular number is n(n+1). Now divide it by two and we get the general formula of the nth triangular number.
No problem lads.
As simple as the area of rectangle divided by 2.
the series is like 1,3,6,10,15..........f(5)=5(5+1)/2=15
We know that the rectangle formed from two of the n th triangular numbers has dimensions n × n + 1 . Thus, the size of just one of the triangular numbers is 2 n × ( n + 1 ) , or 2 n ( n + 1 ) .