Triangular Square

Geometry Level 2

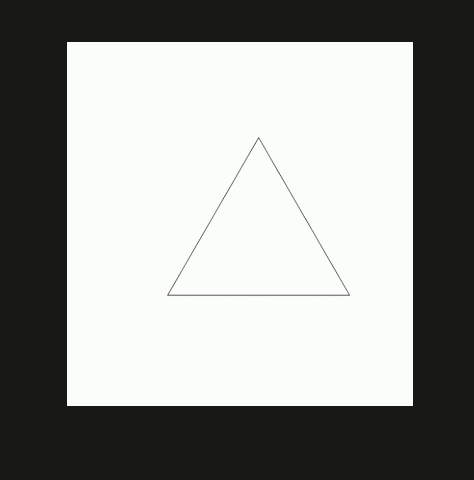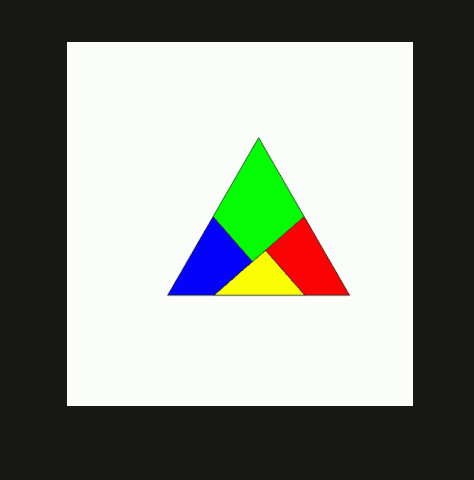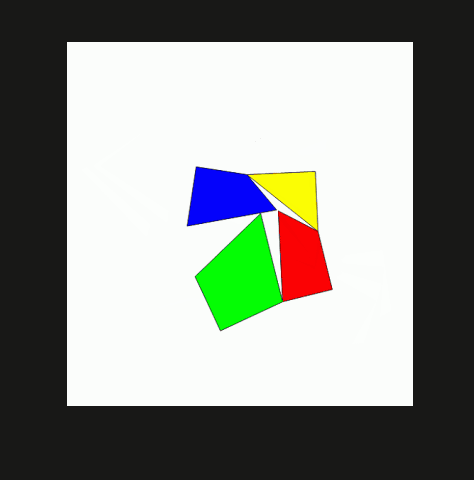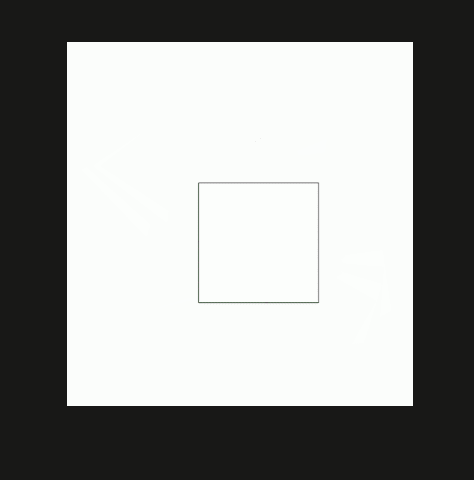
Johnell cuts an equilateral triangular paper whose sides measure 2 cm into pieces. He then rearranges the pieces to form a square without overlapping. How long is the side of the square formed?

\large \sqrt[3]{4}

2 3

\large \sqrt[3]{3}

\large \sqrt[4]{3}

3 solutions

Yasir Soltani
Feb 5, 2016

Let $A_t$ be the area of the Triangle cut then we have $A_t=\frac{1}{2}\times2\sqrt{3}$ $A_t=\sqrt{3}$ As there was no overlapping of sides, this means total area is conserved , if $A_s$ is the area of the square formed with side $x$ and $x\geqslant 0$ then $A_s=A_t$ $x^2=\sqrt{3}$ $x=\boxed{\sqrt[4]{3}}$

John Wyatt
Jan 20, 2016

Cut but no overlap means same amount of area

Area of Triangle = 0.5 a b*sin(C)

Area = 0.5 2 2*(60)

Area = 2*(SQRT(3)/2)

Area = 3^0.5

Area of Square = x^2

x^2 = 3^0.5

x = 3^0.25

Just for sake of curiosity, this link shows how to actually make the cuts to turn the equilateral triangle into a square.

Brian Charlesworth - 5 years, 4 months ago

Gean Llego
Jan 12, 2016

After cutting, the area of the figure is still the same. To find the area of an equilateral triangle, we use the following formula,

A=(a^2 √3)/4

Where a is the length of the side of an equilateral triangle. Finding the area we have,

A=(2^2 √3)/4

A=(4√3)/4

A= √3

Now, since the area of this triangle is equal to the area of the square, we can use the formula of the area of the square to find the length of the side.

A= s^2

Now, we equate the area of the triangle,

√(√3) = s^2

Since we are solving for s, we take the square root of both sides,

√(√3) =√(s^2 )

∜3=s

Or,

s=∜3

Triangular Square

3 solutions

0 pending reports