Tricky altitudes
Δ A B C is an isosceles triangle with A C = B C and A B = 2 . D is the midpoint of A B . The ratio of the radii of it's inscribed circle (radius is r 1 ) and circumscribed circle (radius is r 2 ) is 3 : 8 .
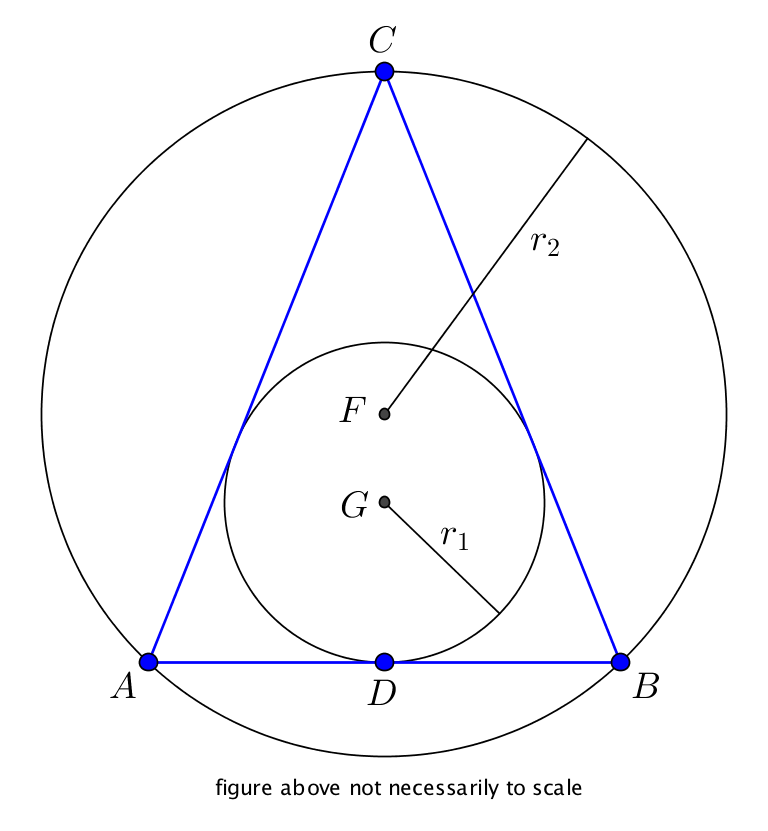
Two different triangles can be formed under these conditions. In one triangle the square of length C D is an integer a , in the other the square of length C D can be expressed as n m , where m and n are coprime positive integers.
What is the value of a + m + n ?
The answer is 31.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
8 solutions
Hello Parth,
I did not think of using Stewart's/Apollonius' theorem. Really nice, so thank you for sharing your solution.
Hope you liked the problem.
Thanks for your contribution, Patrick
An analytical approach
Choose a Cartesian coordinate system with D as it's origin, points A and B on the x -axis such that A ( − 1 , 0 ) and B ( 1 , 0 ) . Point C is on the y -axis such that C ( 0 , c ) . G is the center of the inscribed circle, F is the center of the circumscribed circle and E is the midpoint of BC .
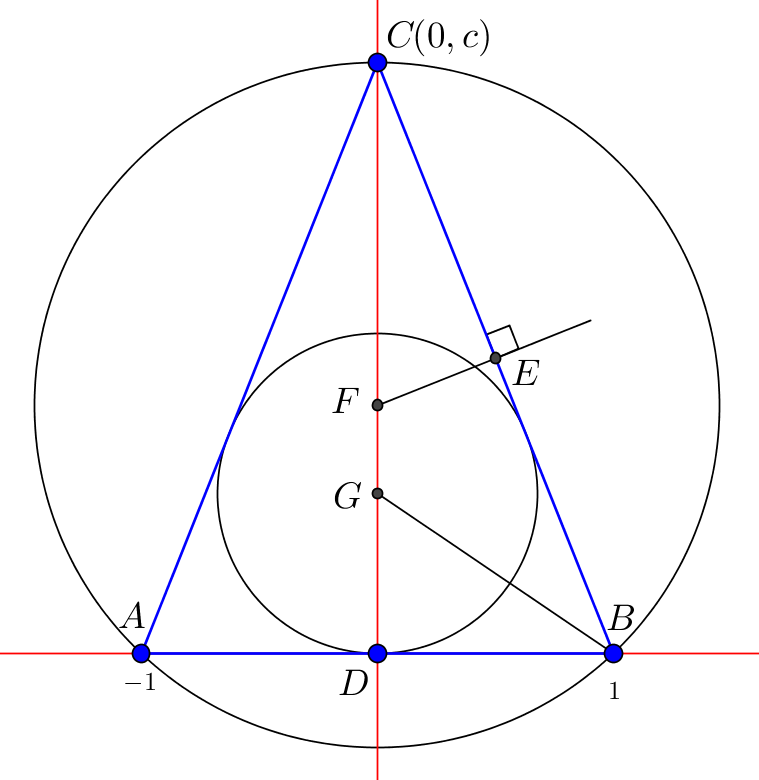
A vector equation of E F is E F : ( 2 1 c 2 1 ) + λ ⋅ ( 1 c )
The x -coordinate of F is 0, so: 2 1 + λ ⋅ c = 0 ⇒ λ = − 2 c 1
It follows that: F = ( 0 , 2 1 c − 2 c 1 ) = ( 0 , 2 c c 2 − 1 )
and: C F = c − 2 c c 2 − 1 = 2 c c 2 + 1
Let D G = h , so G ( 0 , h ) , then the distance from G to BC is: d ( G , B C ) = c 2 + 1 ∣ h − c ∣
This results in: h = c 2 + 1 ∣ h − c ∣ ⇒ D G = h = c − 1 + c 2 + 1 ( because h < c )
We know that the ratio of the radii r 1 : r 2 = 3 : 8 , so 8 ⋅ D G = 3 ⋅ C F or 8 h = 3 ⋅ C F , so:
⇔ ⇔ ⇔ ⇔ ⇔ ⇔ c − 8 + 8 c 2 + 1 = 3 ⋅ 2 c c 2 + 1 − 1 6 + 1 6 c 2 + 1 = 3 c 2 + 3 1 6 c 2 + 1 = 3 c 2 + 1 9 9 c 4 − 1 4 2 c 2 + 1 0 5 = 0 9 ( c 2 ) 2 − 1 4 2 c 2 + 1 0 5 = 0 ( 9 c 2 − 7 ) ( c 2 − 1 5 ) = 0 c 2 = 9 7 ∨ c 2 = 1 5
Because C D = c the values we're interested in are a = 1 5 and n m = 9 7 and therefore the desired sum is: a + m + n = 1 5 + 7 + 9 = 3 1
Moderator note:
It is easier to calculate the radii in terms of A C B = γ . For example, by the extended sine rule, we get 2 R = sin γ A B . The inradius is given by tan 2 1 8 0 − γ = A B / 2 r .
We can then set up the equation to solve for ∠ A C B .
The problem can be more easily solved using cosine rule! . It will reduce a lot of calculation work :)
Log in to reply
Hello Prakhar,
Great to hear that! Please share your solution in detail as it seems to be different from the posted ones so far, so we can all benefit from it.
Kind regards, Patrick
Yes I also used simple trigonometry.
Log in to reply
Hello Kushagra,
Great to hear that! Please share your solution in detail as it seems to be different from the posted ones so far, so we can all benefit from it. Hopefully it will even be different to the one Prakhar had in mind.
Kind regards, Patrick
A solution without much calculation:
Let R , r be the circumradius, inradius of △ A B C respectively. We know that F G 2 = R ( R − 2 r ) . Letting r = 3 x and R = 8 x , we see F G 2 = 8 x ( 8 x − 6 x ) = 1 6 x 2 ⟹ F G = 4 x . Therefore, F D = F G ± r = 4 x ± 3 x = 7 x or x depending on which side D is on.
We know that A D = 2 1 A B = 1 .
If D is closer to G than F , then by Pythagorean Theorem on △ F A D , 1 = A D 2 = F A 2 − F D 2 = ( 8 x ) 2 − ( 7 x ) 2 = 1 5 x 2 ⟹ x 2 = 1 5 1 In this case C D 2 = ( R + F D ) 2 = 2 2 5 x 2 = 1 5 .
If D is closer to F than G , then by Pythagorean Theorem on △ F A D , 1 = A D 2 = F A 2 − F D 2 = ( 8 x ) 2 − x 2 = 6 3 x 2 ⟹ x 2 = 6 3 1 In this case C D 2 = ( R − F D ) 2 = 4 9 x 2 = 9 7 .
So a = 1 5 , m = 7 and n = 9 which gives our final answer of 1 5 + 7 + 9 = 3 1 .
Hello Daniel,
Thank you for your solution. I really like your approach using Euler's theorem in geometry for the distance between the circumcentre F and the incentre G .
Hope you liked the problem.
Thanks for your contribution, Patrick
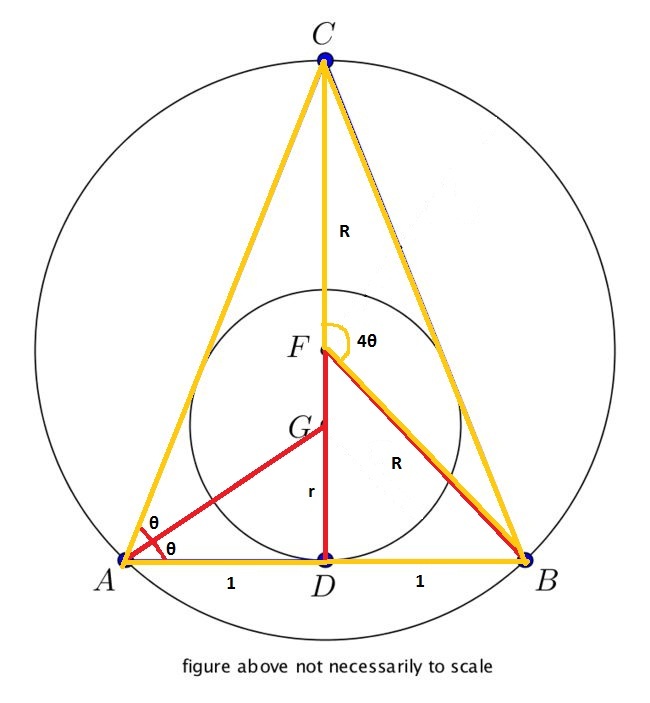
From the diagram, we can see that t a n θ = r and t a n 2 θ = C D . We can also find out that s i n 4 θ = R 1 . Since we know that R = 3 8 r , we can then substitute r = t a n θ into s i n 4 θ = 8 r 3 . Thus, we get s i n 4 θ = 8 t a n θ 3 . Expand s i n 4 θ into the form 4 s i n θ c o s θ ( 1 − 2 s i n 2 θ ) and t a n θ to c o s θ s i n θ . Put them back into the equation and c o s θ will cancel out nicely leaving a quadratic equation in terms of s i n 2 θ which is 4 s i n 2 θ ( 1 − 2 s i n 2 θ ) = 8 3 . Solve the equation and obtain the two values of θ . Then, put them back into the equation C D = t a n 2 θ and square it and you will get 9 7 and 1 5 .
The same
Denote the area by K .
A C = B C = a , A B = 2 ⟹ s = a + 1 . By Heron's Formula, we have K = s ( s − a ) ( s − b ) ( s − c ) = a 2 − 1 Thus, we have r s 4 R a b c = a 2 − 1 ⟹ r = a + 1 a 2 − 1 = a 2 − 1 ⟹ R = 2 a 2 − 1 a 2
Since r R = 3 8 , we have r R = a + 1 a 2 − 1 2 a 2 − 1 a 2 = 3 8 ⟹ 3 a 2 − 1 6 a + 1 6 = 0 ⟹ a = 3 4 , 4
Observe that the desired value is a 2 − 1 . The answer follows from substituting a in.
Hello Alan,
I really like all the different solutions. Thanks for sharing your clever approach! Hope you liked the problem.
Thanks for your contribution, Patrick
Sorry, but I'm still learning LaTeX.

I solved the same way as Daniel Liu.
My solution is identical to the Daniel Liu
An easy geometrical solution
Let A C = B C = x
Using r = s Δ and R = 4 Δ a b c , where s = 2 a + b + c and Δ 2 = s ( s − a ) ( s − b ) ( s − c ) = 8 s ( b + c − a ) ( a + c − b ) ( a + b − c )
Now since
R r = 8 3
⇔ s a b c 4 Δ 2 = 8 3
⇔ a b c ( b + c − a ) ( a + c − b ) ( a + b − c ) = 4 3
⇔ 2 x 2 ( 2 x − 2 ) ( 2 ) ( 2 ) = 4 3
⇔ 1 6 ( x − 1 ) = 3 x 2
⇔ 3 x 2 − 1 6 x + 1 6 = 0
⇔ ( 3 x − 4 ) ( x − 4 ) = 0
⇔ x = 4 3 ∨ x = 4
From Apollonius Theorem,
A C 2 + B C 2 = 2 ( C D 2 + A D 2 )
⇔ 2 x 2 = 2 ( C D 2 + 1 )
⇔ C D 2 = x 2 − 1
⇔ C D 2 = 1 5 ∨ 9 7
∴ a = 1 5 a n d n m = 9 7
⇒ a + m + n = 3 1