Tricky Locus
A chord of length 6 slides inside a triangle of side lengths 1 3 , 1 4 and 1 5 .
The midpoint of the chord traces a closed curve whose area is A − B π , where A and B are integers.
Find B + 3
The answer is 12.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
This is one of the results I found really beautiful!!!! I was able to solve the question only because I knew this already.....!!
That's really nice - thank you for posting the problem! By the way, the image in the problem looks different from the locus I found - did you use different parameters to draw it?
Log in to reply
I copied it from here
Does it look like the one I posted?
Great gif! :)
This question really confused me. From reading the solution, I imagine that the key additional piece of information is that the series of chords in question are all of fixed length?
Log in to reply
The problem states that they are all 4 units long
Log in to reply
They way it was written I interpreted unit to be an unknown.
I recommend this problem as well.
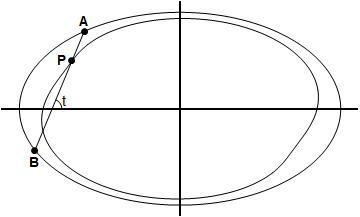
Suppose that A B is a rod of length p + q , and that P is a point on the rod such that A P = p and P B = q . We let A B slide around the triangle in such a manner that, as the points of contact A and B make a full anticlockwise tour around the triangle, the angle t that the rod makes with the x -axis increases from 0 to 2 π . We now want to know about the locus of P .
If the coordinates of P are ( x , y ) , where x , y are functions of t , then the coordinates of A and B are A ( x + p cos t , y + p sin t ) B ( x − q cos t , y − q sin t ) Thus the area of the region swept out by the point P is X P = 2 1 ∫ 0 2 π ( x y ˙ − x ˙ y ) d t while the area of the region swept out by the point A is X A = 2 1 ∫ 0 2 π ( x + p cos t ) ( y ˙ + p cos t ) − ( x ˙ − p sin t ) ( y + p sin t ) ) d t = X P + π p 2 + p Q and the area of the region swept out by the point B is X B = 2 1 ∫ 0 2 π ( x − q cos t ) ( y ˙ − q cos t ) − ( x ˙ + q sin t ) ( y − q sin t ) ) d t = X P + π q 2 − q Q where Q = 2 1 ∫ 0 2 π ( ( x + y ˙ ) cos t + ( y − x ˙ ) sin t ) d t and hence q X A + p X B = ( p + q ) X P + π p q ( p + q ) This calculation can be extended to handle the case when the outside curve is only piecewise differentiable, as here In this case X A = X B = 8 4 and p = q = 3 , obtaining the area X P = 8 4 − 9 π , making the answer 9 + 3 = 1 2 .
Here is a more direct proof of this particular problem. As Steve's diagram shows, for most of the time the rod simply slides along one of the edges, until it has to negotiate a corner of the triangle. Thus X P is the area of the triangle, minus the three regions missed at the vertices. Suppose that the rod has to move past a vertex of angle α . Suppose that the origin of our coordinate system is at the triangle's vertex, while one side of the triangle lies along the x -axis, while the second side of the triangle lies in the first quadrant portion of y = x tan α . The the coordinates of the midpoint of the rod, when the rod makes an angle t with the x -axis, are x = 3 cos t + 6 cot α sin t , y = 3 sin t 0 ≤ t ≤ π − α In this case x y ˙ − x ˙ y = 9 , and so the area "missed" at this vertex is 2 9 ( π − α ) . Thus the area missed as the rod goes around the triangle P Q R is 2 9 ( ( π − P ) + ( π − Q ) + ( π − R ) ) = 2 9 ( 3 π − P − Q − R ) = 9 π
I've changed the question. Please make suitable changes to your solution.
Log in to reply
Why on earth could you just not post a new problem? The old problem was interesting, at least if you took the time to understand the theorem.
Should i post the triangle problem as a separate problem? I just didn't want to post two problems involving the same cocept, in a row, so i revised this problem
Log in to reply
It's a bit late, but that's what I think you should have done. If nothing else, the question now asks for B + 3 , without requiring knowledge of A .
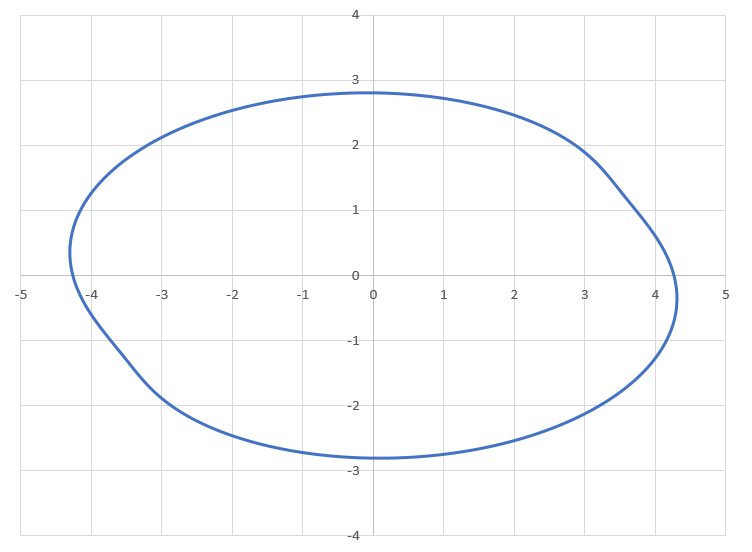
For fun, here is the shape of the locus I got. It looks a bit different from the image in the problem, but the enclosed area turns out to be 1 2 π regardless.
The locus for the revised triangular problem is below:
https://ds055uzetaobb.cloudfront.net/uploads/xAcURemDA4-locus-tri.png
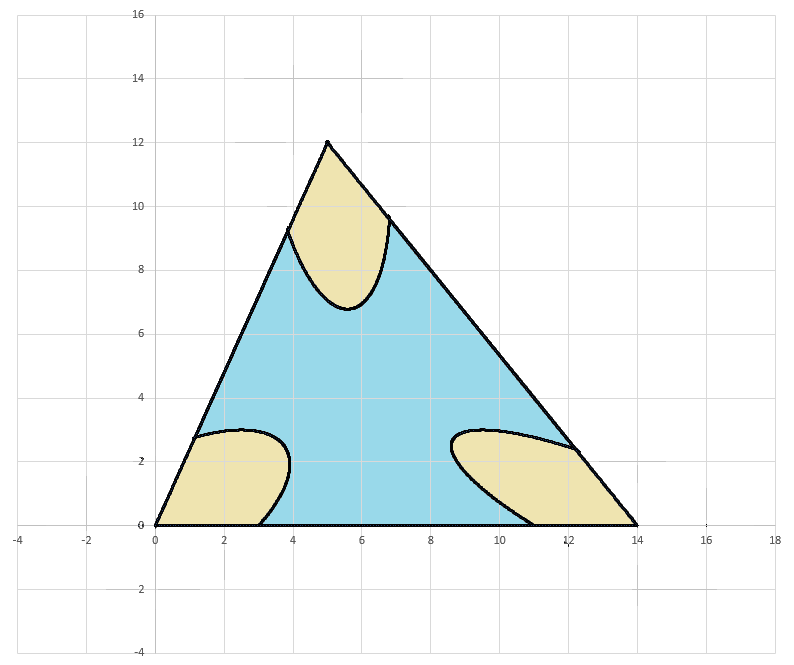
I've changed the question, could you help me with a picture of the new locus?
Log in to reply
I have added the revised graphic to my solution, in addition to the image link.
Sure, I'll try it this afternoon
Holditch's theorem states that if a chord of fixed length is allowed to rotate inside a convex closed curve, then the locus of a point on the chord a distance p from one end and a distance q from the other is a closed curve whose enclosed area is less than that of the original curve by p q π
In this case p = q = 3 .
Area enclosed by the locus = Area of the triangle − p q π = 8 4 − 9 π