Trigonometry! #75
If 7 csc θ − 3 cot θ = 7 then find the value of ∣ 7 cot θ − 3 csc θ ∣
This problem is part of the set Trigonometry .
The answer is 3.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
please reply this method is correct or wrong
7cscx-3cotx=7 7/sinx-3cosx/sinx=7 (7-3cosx)/sinx=7 7-3cosx=7sinx 7(1-sinx)=3cosx 1-sinx=3/7cosx---------------------------eq 1 let 7cotx-3cscx=k thefore by same above method we get 7-3cosx=ksinx we know 1-sin^2x=cos^2 1+sinx)(1-sinx)=cos^2 1+sinx)3/7cosx=cos^2 now simplifing it we get 7-3cosx=3sinx thus k=3
Log in to reply
Could you please use latex? It's hard to understand what you're trying to say.
7 csc θ − 3 cot θ = 7
Squaring both sides,
4 9 csc 2 θ − 4 2 csc θ cot θ + 9 cot 2 θ = 4 9
4 9 ( 1 + cot 2 θ ) − 4 2 csc θ cot θ + 9 ( csc 2 θ − 1 ) = 4 9
4 9 + 4 9 cot 2 θ − 4 2 csc θ cot θ + 9 csc 2 θ − 9 = 4 9
4 9 cot 2 θ − 4 2 csc θ cot θ + 9 csc 2 θ = 9
( 7 cot θ ) 2 − 2 ( 7 cot θ ) ( ( 3 csc θ ) + ( 3 csc θ ) 2 = 3 2
( 7 cot θ − 3 csc θ ) 2 = 3 2
7 cot θ − 3 csc θ = ± 3
∣ 7 cot θ − 3 csc θ ∣ = 3
Thanks. I updated the question to ask for the absolute value, which is 3.
Log in to reply
OK. Thanks for your reply and your attention.
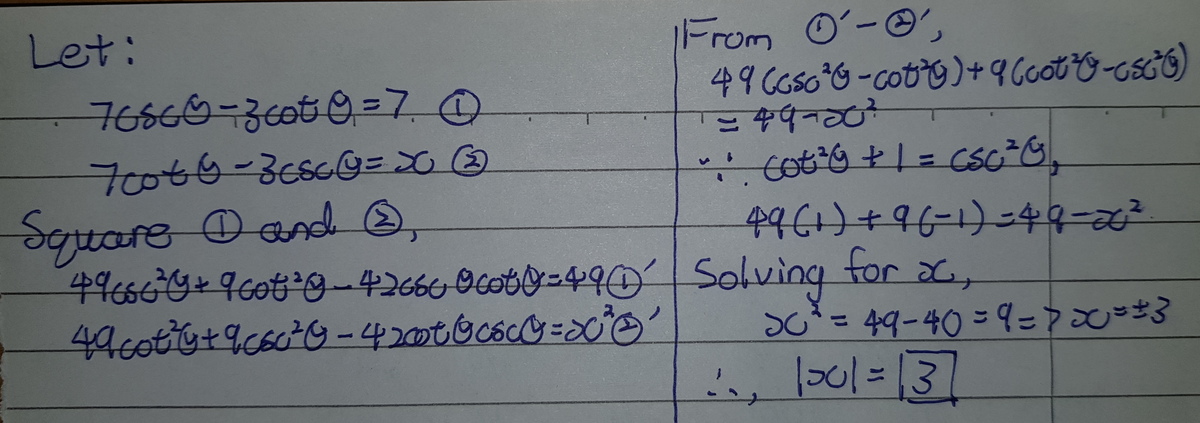

I dont know if somebody has a simplier solution.
7cscx - 3 cotx= 7 We square both side
49csc^2 x + 9cot^2 x - 42cscx.cotx = 49 49(cot^2x+1) + 9 cot^2 x - 42cscx.cotx=49 58cot^2x - 42 cscx.cotx= 0 58 cot^2x=42 cscx.cotx 58 cotx= 42 csc x 29 cotx= 21 csc x
Substitute to the equation 29/3 (cotx) - 3cotx= 7 (20/3) cot x= 7 Cotx= 21/20 Cscx= 29/20
So for 7cotx-3csc x= 7.21/20 - 3.29/20 = 60/20=3