The Pyramids

Number Theory Level 3

If $a$ and $b$ are positive integers such that $\frac{1} {a} + \frac{1} {b} = \frac{1} {5}$

Then total number of pairs of $(a,b)$ is?

2 solutions

Brian Charlesworth
Feb 12, 2015

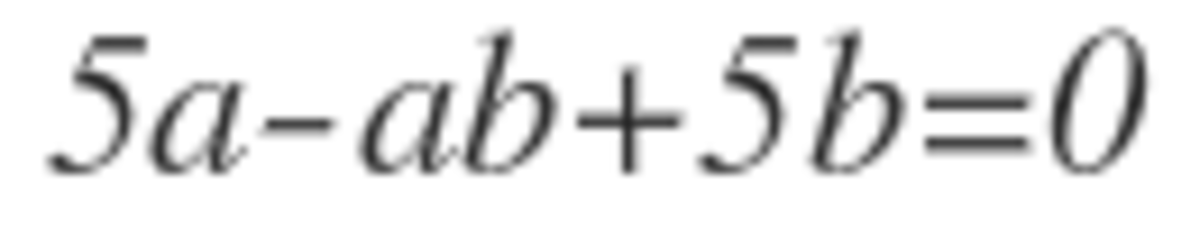
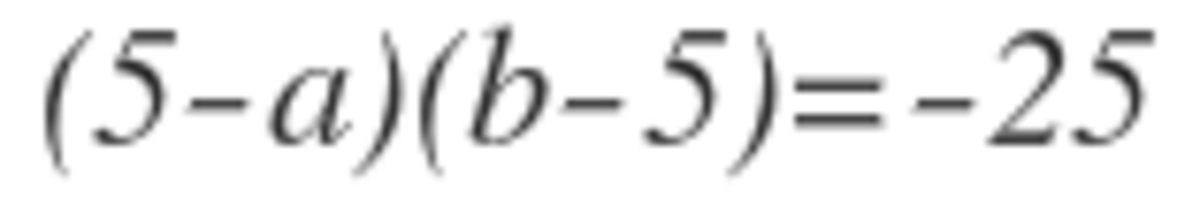
Rewrite the equation as

$\dfrac{a + b}{ab} = \dfrac{1}{5} \Longrightarrow 5a + 5b = ab \Longrightarrow ab - 5a - 5b = 0 \Longrightarrow (a - 5)(b - 5) = 25.$

Now since $25$ can only be factored as the ordered product pairs $1*25 = 5*5 = 25*1$ , we have only $\boxed{3}$ possible pairs $(a,b)$ , namely

$(6,30), (10,10), (30,6)$ .

Deb Sen
Mar 21, 2015

First simplify:

Substract ab:

Then use a very useful tool called Simon's favorite factoring trick

Factor out a:

Subtract 25 from each side for convenient factoring

Finally you get :

From this you get pairs for (a,b) that are positive integers are (30,6),(6,30) and (10,10), so therefore you have 3 all together.