A geometry problem by Aziz Alasha

From the figure above, find the area of the coloured regions x + y to two decimal places. Use π ≈ 7 2 2 .
The answer is 18.29.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Since the area of both halves of two-sided arc F C is the area of the combination of two inner arcs A F and F B , the sum of the shaded region is equivalent to determining the area of the outer sliced arc in the quarter circle with radius 8 .
Thus, the answer is Area Shaded = Area Quarter circ A B C − Area Δ A B C = 4 π ⋅ 8 2 − 2 1 ⋅ 8 2 = 1 6 π − 3 2 ≈ 1 8 . 2 9
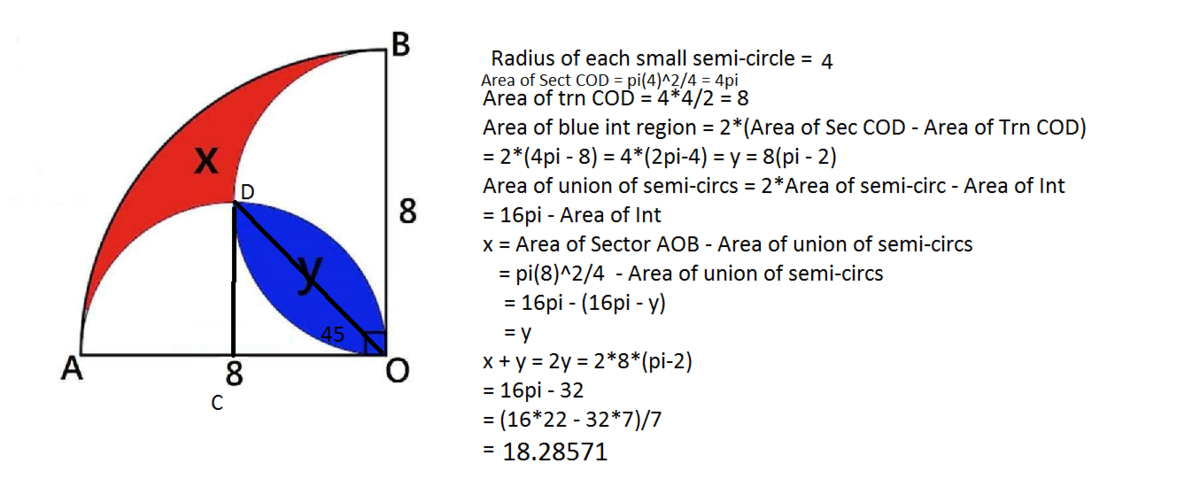
We note that:
2 x = Area of sector A O C − Area of sector A D E − Area of △ O D E = 8 1 π ( 8 2 ) − 4 1 π ( 4 2 ) − 2 1 ( 4 2 ) = 4 π − 8
2 y = Area of sector O D E − Area of △ O D E = 4 1 π ( 4 2 ) − − 2 1 ( 4 2 ) = 4 π − 8
x + y = 4 ( 4 π − 8 ) = 1 6 π − 3 2 = 1 6 × 7 2 2 − 3 2 ≈ 1 8 . 2 9