Trying to fit in 2016
Algebra
Level
3
The number is:
None of these choices
Real
Has modulus 1
Purely imaginary
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
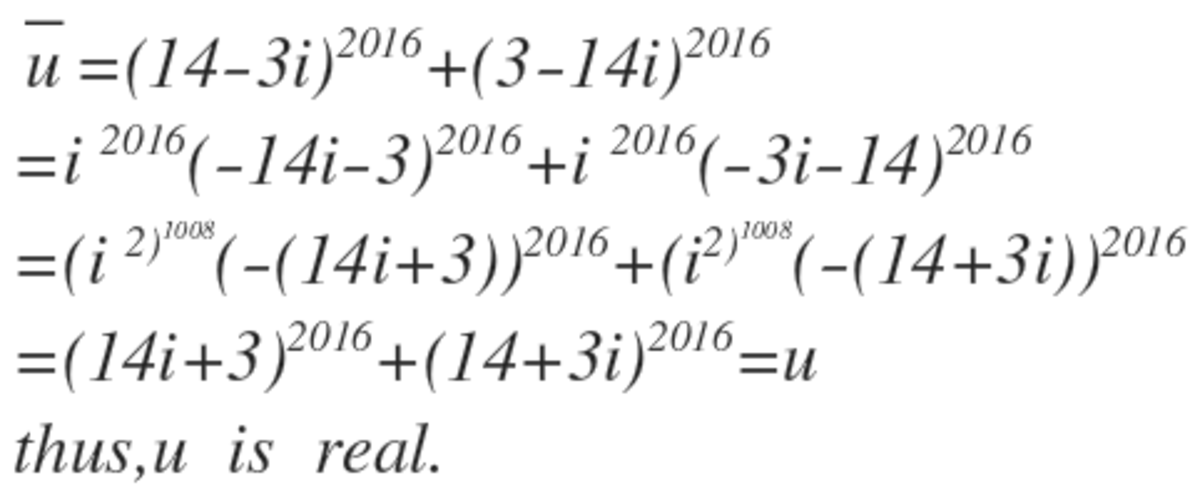
u = ( 1 4 + 3 i ) 2 0 1 6 + ( 3 + 1 4 i ) 2 0 1 6 = ( 1 4 + 3 i ) 2 0 1 6 + i 2 0 1 6 i 2 0 1 6 × ( 3 + 1 4 i ) 2 0 1 6 = ( 1 4 + 3 i ) 2 0 1 6 + 1 ( 3 i − 1 4 ) 2 0 1 6 = ( 1 4 + 3 i ) 2 0 1 6 + ( − 1 ) 2 0 1 6 ( 1 4 − 3 i ) 2 0 1 6 = ( 1 4 + 3 i ) 2 0 1 6 + ( 1 4 − 3 i ) 2 0 1 6 = k = 0 ∑ 2 0 1 6 ( 2 0 1 6 k ) 1 4 k ( 3 i ) 2 0 1 6 − k + k = 0 ∑ 2 0 1 6 ( − 1 ) k ( 2 0 1 6 k ) 1 4 k ( 3 i ) 2 0 1 6 − k
We note that all the k = odd terms, which are imaginary, cancel out. Therefore, u is real .