Tunnel Vision
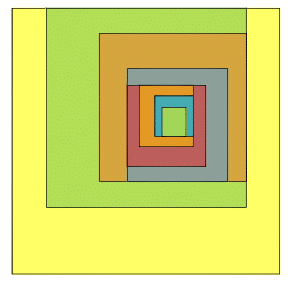 Inside a unit square, there is an infinite series of squares, each with sides
4
3
of the sides of the square one size larger. Their positions are at the top, on the right, at the bottom, on the left, at the top again, and so on, centered on the side they are on. As the size of the squares goes to zero, their location converges to a point
(
x
,
y
)
, where the origin of the coordinate system is the lower left corner of the original square.
Inside a unit square, there is an infinite series of squares, each with sides
4
3
of the sides of the square one size larger. Their positions are at the top, on the right, at the bottom, on the left, at the top again, and so on, centered on the side they are on. As the size of the squares goes to zero, their location converges to a point
(
x
,
y
)
, where the origin of the coordinate system is the lower left corner of the original square.
If the ratio y x can be expressed as b a , where a and b are coprime positive integers, submit your answer as a + b .
The answer is 57.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
I get the maps, the fact that they fix the image, the fact that z 0 is fixed, and the solution. It all works. I still have a problem though. It fixes the entire image, not just z 0 , so how come the solution gives us just that one point?
Log in to reply
I may be using the wrong term there saying the image is "fixed".
Rather, I just mean to use the symmetry of self-similarity. This is analogous to how a square has 90 degree rotational symmetry, but only the centroid of the square is fixed by this rotation.
More explicitly, note that 3 4 i ( z − 8 1 − i ) = 3 4 i ( z − z 0 ) + z 0
This shows that the map I gave can be rewritten as a 90 degree rotation about z 0 followed by a stretch about the point z 0 , so in this context, it obviously is the unique fixed point.
Let's track the position of the center as it changes from one square to the next smaller one and so on. Both coordinates start at 2 1 . y is the first to increase, by the difference between 2 1 and 4 3 of 2 1 , that is by 8 1 . Next only x changes. By the time it's y 's turn, the starting square is only ( 4 3 ) 2 = 1 6 9 of the original. The change is a decrease and it is 8 1 of this figure. The sequence for y is:
y = 2 1 + 8 1 × ∑ n = 0 ∞ ( − 1 6 9 ) n = 2 1 + 8 1 × 2 5 1 6 = 5 0 2 9
The sequence of locations for x starts with the same 2 1 but the additions and subtractions are all 4 3 of those for y .
x = 2 1 + 8 1 × 4 3 × ∑ n = 0 ∞ ( − 1 6 9 ) n = 2 1 + 8 1 × 4 3 × 2 5 1 6 = 2 5 1 4 .
So the ratio y x goes to 2 9 2 8 .
Let z 0 = x + i y denote the point in question on the Argand plane. Then we consider the following composition of maps:
In particular, this means that the composition of these maps, if we restrict our attention to the unit square and ignore colors, fixes the image. Therefore, the point z 0 we want to find is fixed by the composition of these maps, giving the equation: z 0 = 3 4 i ( z 0 − 8 1 − i )
Solving for z 0 yields z 0 = 2 5 1 4 + 5 0 2 9 i and y x = 2 9 / 5 0 1 4 / 2 5 = 2 9 2 8