Twin Incircles
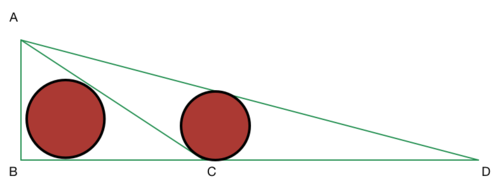 Let
be a triangle with sides
,
and
. Extend the line
up to a point
so that
is closer to
than to
.
Let
be a triangle with sides
,
and
. Extend the line
up to a point
so that
is closer to
than to
.
This new point is a point such that the radius of the incircle of the triangle is equal to the radius of the incircle of the triangle . If the ratio can be expressed as , and being coprime, then find the value of
The answer is 13.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
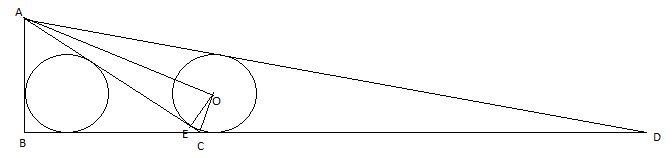
First of all, let's find the measure of the radius of the triangle △ A B C .
The easiest way to do so, I believe, is by finding the area of the triangle. Since it's a ( 3 , 4 , 5 ) triangle, we know it's a right triangle and thus its area equals 2 3 ∗ 4 = 6 . This area can also be calculated by finding the semiperimeter of the triangle, and multiplying it by the radius of the incircle. Let's call this radius r; the semiperimeter of the triangle equals 2 3 + 4 + 5 = 6 . Thus, 6 ∗ r = 6 , and so r = 1 .
Now we will adopt a similar procedure with triangle △ A C D . I will call C D = a and A D = b . The area of this triangle equals 2 3 a ; the reason why is because the segment B D is perpendicular to the segment A B , the latter of which measures 3 and is also a height of the triangle △ A C D . Also, the semiperimeter of this triangle equals 2 a + b + 5 , thus 2 r ∗ ( a + b + 5 ) = 2 3 a ; since we know r = 1, we can write the equation as b = 2 a − 5 .
Now, to find the values of a and b , we can apply Pythagoras' Theorem on the triangle △ A B D to yield the following:
3 2 + ( 4 + a ) 2 = b 2
3 2 + ( 4 + a ) 2 = ( 2 a − 5 ) 2
9 + ( 1 6 + 8 a + a 2 ) = ( 4 a 2 − 2 0 a + 2 5 )
3 a 2 − 2 8 a = 0
a = 0 or a = 3 2 8
a cannot have a value of zero because it would make b = − 5 and we'd have a negative length, which is absurd; thus, we find that a = 3 2 8 , and then b = 2 ∗ 3 2 8 − 5 = 3 5 6 − 1 5 = 3 4 1 .
Thus, the ratio C D A D = 2 8 4 1 , and therefore the difference sought is 4 1 − 2 8 = 1 3