Two Circles in a Triangle
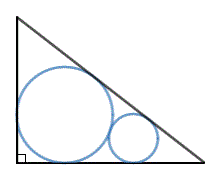 If the incircle to a right triangle has a radius of
6
7
6
2
and a circle tangent to the incircle and the hypotenuse and one leg of the triangle has a radius of
3
4
5
0
, find the perimeter of the right triangle.
If the incircle to a right triangle has a radius of
6
7
6
2
and a circle tangent to the incircle and the hypotenuse and one leg of the triangle has a radius of
3
4
5
0
, find the perimeter of the right triangle.
The answer is 80605.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
Put an origin at the right-angle. Let the two vertices of the triangle on the x -axis be ( 0 , 0 ) and ( a , 0 ) , and the vertex on the y -axis be ( 0 , b ) , so the sides of the triangle are a , b and c = a 2 + b 2 .
The incircle has centre ( 6 7 6 2 , 6 7 6 2 ) ; the smaller circle has centre ( u , 3 4 5 0 ) . The distance between their centres is the sum of their radii; so ( u − 6 7 6 2 ) 2 + ( 6 7 6 2 − 3 4 5 0 ) 2 = ( 6 7 6 2 + 3 4 5 0 ) 2
Solving, u = 1 6 4 2 2 .
The centres and the point ( a , 0 ) form a straight line. Equating gradients (or rather, their reciprocals, to get a nicer equation), 3 4 5 0 a − 1 6 4 2 2 = 6 7 6 2 a − 6 7 6 2
Solving, a = 2 5 2 9 6 9
The inradius of a right-angled triangle is given by 2 1 ( a + b − c ) ; we also have a 2 + b 2 = c 2 . Putting these together and solving, we find b = 2 0 5 8 0 and c = 2 6 7 0 8 1 , so the perimeter of the triangle is 8 0 6 0 5 .
Nice solution!
Let
R
=
6
7
6
2
,
r
=
3
4
5
0
,
D
,
E
,
F
,
G
,
H
points of tangency of the sides of the triangle with the circles,
K
,
L
the centers of the circles,
C
E
=
C
F
=
x
,
B
G
=
B
H
=
y
(tangent lines to the circles),
L
M
⊥
K
D
.
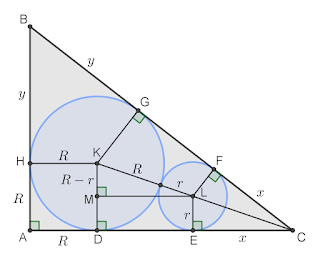
By Pythagoras theorem on △ K L M , L M = ( R + r ) 2 − ( R − r ) 2 = 2 R r = 9 6 6 0 , hence, D E = F G = L M = 9 6 6 0 .
The points K and L lie on the angle bisector of ∠ A C B and by similarity of △ C L E and △ C K D , r x = R x + 9 6 6 0 which solves to x = 1 0 0 6 2 . 5 .
Again by Pythagoras theorem, this time on △ A B C , ( y + 9 6 6 0 + x ) 2 = ( y + R ) 2 + ( R + 9 6 6 0 + x ) 2 which solves to y = 1 3 8 1 8 .
Finally, for the perimeter P of △ A B C we have P = 2 ( x + y + R + 9 6 6 0 ) = 8 0 6 0 5 .
Great solution!
The base of the triangle has length b = 6 7 6 2 + ( 6 7 6 2 + 3 4 5 0 ) 2 − ( 6 7 6 2 − 3 4 5 0 ) 2 ( 1 + 3 3 1 2 3 4 5 0 ) = 2 6 4 8 4 . 5 . Let the triangle be △ A B C right angled at C and base C A . Then sin ( 2 A ) = 1 0 2 1 2 3 3 1 2 . This gives tan A = 1 0 8 1 8 4 0 . So a = ∣ B C ∣ = b tan A = 2 0 5 8 0 and c = ∣ A B ∣ = a 2 + b 2 = 3 3 5 0 4 . 5 . Therefore the perimeter of the triangle is 2 6 4 8 4 . 5 + 2 0 5 8 0 + 3 3 5 0 4 . 5 = 8 0 6 0 5
Let R = 6 7 6 2 , r = 3 4 5 0 , and the smallest angle of the right triangle be θ . Then the base of the triangle is b = tan 2 θ R + R , the height of the triangle is h = b tan θ , and the area of the triangle is A = 2 b h . Since R is the inradius of the triangle, A = 2 R p , where p is the perimeter of the triangle. Then we have:
2 R p ⟹ p = 2 1 ( tan 2 θ R + R ) 2 tan θ = R ( t 1 + 1 ) 2 × 1 − t 2 2 t = t ( 1 − t ) 2 R ( 1 + t ) Let t = tan 2 θ
To find t = tan 2 θ , we let the distance between the left vertex to the center of the small circle be x . Then by similar triangles we have:
r x R x ⟹ x ⟹ t = R x + r + R = r x + r 2 + r R = R − r r ( R + r ) = 1 0 6 3 7 . 5 = tan 2 θ = x 2 − r 2 r = 3 5 1 2
Therefore p = t ( 1 − t ) 2 R ( 1 + t ) = 8 0 6 0 5