Two much of a good thing?
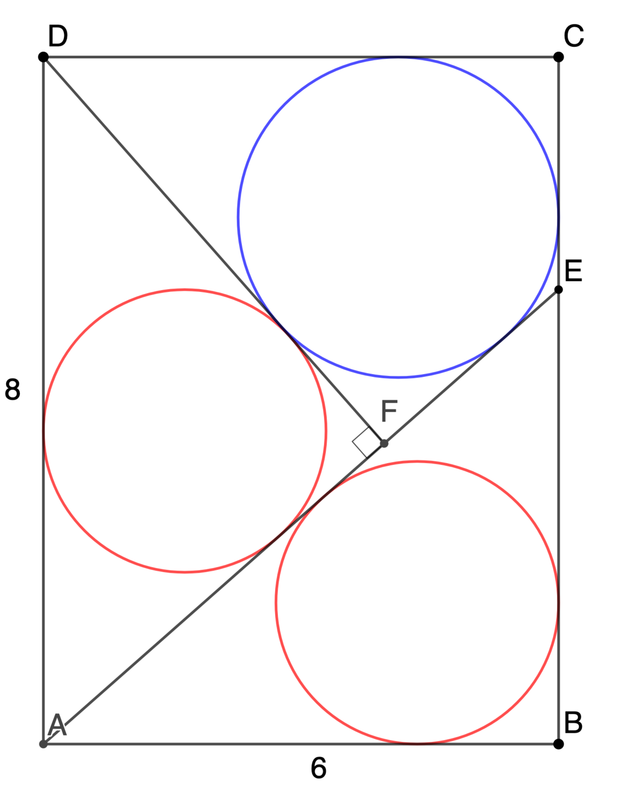
is a rectangle. and . is perpendicular to . If the red circles are congruent, what is the ratio of the radius of the blue circle to the radius of a red circle? Express the ratio as where and are integers and is square-free. Submit .
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
△ A D F and △ A B E have congruent incircle and three vertex angles. The two triangles are congruent and therefore D F = A B = 6 .
Extend D C and A E to meet at G . We note that △ D F G and △ A B E are similar. Then the ratio of the radius of the blue circle to that of the red circle r r e d r b l u e = E B D F = 8 2 − 6 2 6 = 2 7 6 = 7 3 . Therefore a + b = 3 + 7 = 1 0 .