Try your hand at the 7's

Consider the function $g_\alpha(x) \; = \; \fra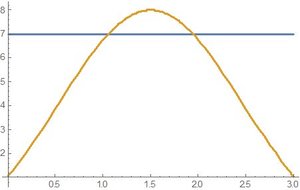c{(x+1)^3}{x^\alpha} \qquad x > 0$ for any $0 < \alpha < 3$ . Then $g_\alpha'(x) \; = \; \frac{(x+1)^2}{x^{\alpha+1}}\big[(3-\alpha)x - \alpha\big]$ from which it is clear that the minimum value of $g_\alpha(x)$ is $h(\alpha) \; = \; g_\alpha\left(\tfrac{\alpha}{3-\alpha}\right) \; = \; \frac{27}{(3-\alpha)^{3-\alpha} \alpha^\alpha}\;.$ Plotting the function of $h(\alpha)$ against $\alpha$ , we see that $h(\alpha) > 7$ when $\alpha$ lies in a specific range. Solving the equation $h(\alpha) = 7$ numerically, we deduce that $h(\alpha) > 7$ for $1.05581 < \alpha < 1.94419$ , approximately.

Now consider the function $f_\alpha(x) \; = \; (x+1)^3 - 7x^\alpha \; = \; x^\alpha\big[g_\alpha(x) - 7\big] \qquad x > 0$ It is clear that $f_\alpha(x)$ will be negative for large enough $x$ whenever $\alpha \ge 3$ ; in the range $0 < \alpha < 3$ we see that $f_\alpha(x) > 0$ for all $x > 0$ provided that $g_\alpha(x) > 7$ for all $x > 0$ , and this happens when $1.05581 < \alpha < 1.94419$ .

Back to the problem. We note that (using the AM/GM inequality) $(a+1)(b+1)(c+1) \; = \; abc + (ab + ac + bc) + (a + b + c) + 1 \; \ge \; abc + 3(abc)^{\frac23} + 3(abc)^{\frac13} + 1 \; = \; \big(1 + (abc)^{\frac13}\big)^3$ Note that this inequality can be made an equality by choosing $a=b=c$ , and so the next stage is the key one. Provided that $1.05581 < \alpha < 1.94419$ we deduce that $(a+1)(b+1)(c+1) \;\ge\; \big(1 + (abc)^{\frac13}\big)^3 \; > \; 7\big[(abc)^{\frac13}\big]^\alpha \qquad a,b,c > 0$ and hence that $\big[(a+1)(b+1)(c+1)\big]^7 \; > \; 7^7 (abc)^{\frac73\alpha} \qquad a,b,c > 0$ and so we deduce that the inequality $\big[(a+1)(b+1)(c+1)\big]^7 \; > \; 7^7 (abc)^\sigma \qquad a,b,c > 0$ holds provided that $2.46355 < \sigma = \tfrac73\alpha < 4.53645$ . Thus the least integer value of $\sigma$ that works is $\boxed{3}$ .