Uncertain Mechanics - 1

Classical Mechanics Level 3

Consider a rigid rod, one end of which is hinged at the origin and is placed along the positive X-axis. The rod is capable of rotating about the Z-axis and has a length $L=1$ and a mass per unit length $\lambda(x)$

$\lambda(x) = \lambda_o \mathrm{e}^{-x}$

It so happens that the parameter $\lambda_o$ has a value which is not exactly known. It is only known that the parameter is normally distributed such that its expected value is $\mu=100$ and its standard deviation is $\sigma=10$ . Compute the probability that the moment of inertia of the rod about the Z-axis is greater than $17$ .

Note:

$\mathrm{e} \approx 2.718$ is the Euler's number

The answer is 0.2792.

1 solution

Dark Angel
Jun 24, 2020

To be honest i dont know much about probability and statistics so i have used normal distribution function probability calculator 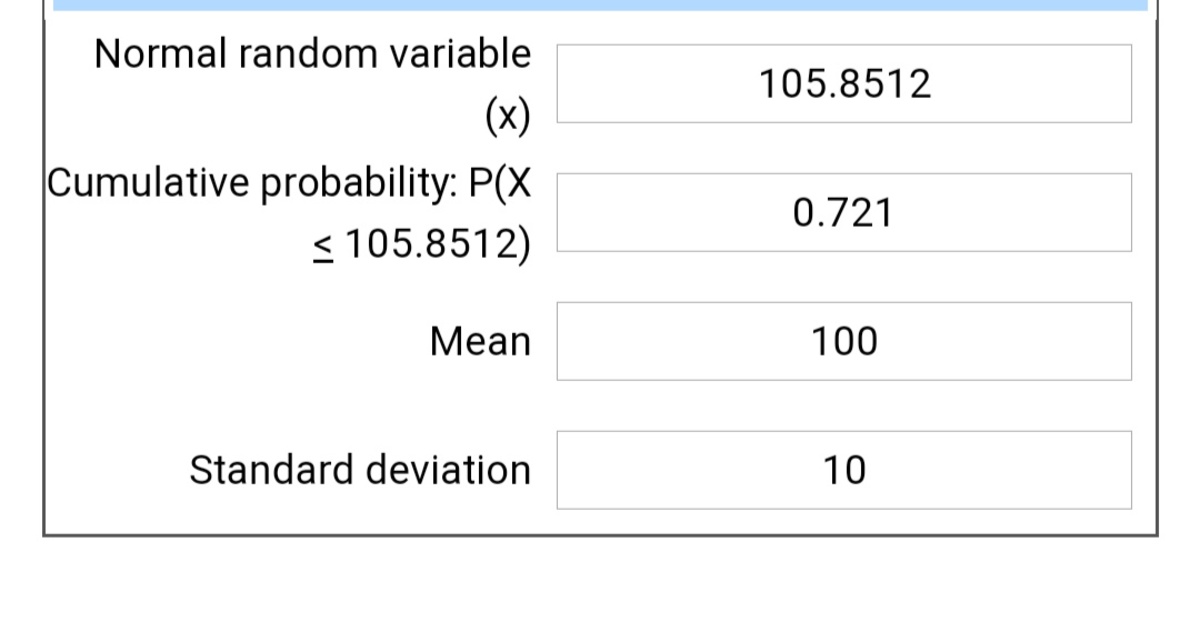as probability less than 105.8512 is 0.721 so greater than 105.8512 is 0.279

Thanks for the solution. Calculating the probability boils down to solving the following exponential integral:

$P(I > 17) = \frac{1}{\sqrt{2 \pi} \sigma_1} \int_{17}^{\infty} \mathrm{e}^{-\frac{(I - \mu_1)^2}{2 \sigma_1^2}} \ dI$

$\sigma_1 = \left(2 - \frac{5}{e}\right) \sigma$ $\mu_1 = \left(2 - \frac{5}{e}\right) \mu$

Karan Chatrath - 11 months, 3 weeks ago

I suppose it is √2π than 2π

dark angel - 11 months, 3 weeks ago

Yes it is; nice catch. Thanks for pointing it out.

Karan Chatrath - 11 months, 3 weeks ago

Uncertain Mechanics - 1

The answer is 0.2792.

1 solution

0 pending reports