Use different tools
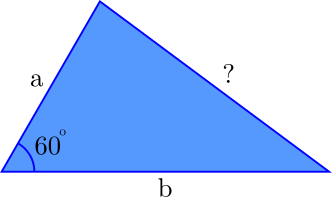
Given positive numbers , , such that . Let and the minimum value of be . Find .
Hint:
The answer is 100.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Employing the hint, the question mark should be replaced by a 2 + b 2 − 2 a b cos 6 0 ∘ = a 2 + b 2 − 2 a b ( 2 1 ) = a 2 + b 2 − a b on the basis of cosine rule. If a were replaced by c , we have b 2 + c 2 − b c for the other question mark. Now imagine c were joined to the 60 degree vertex. Then Q would be minimised if the two lengths with the question marks form a straight line when joined together. This minimum value would then be a 2 + c 2 − 2 a b cos 1 2 0 ∘ = a 2 + c 2 − 2 a c ( − 2 1 ) = a 2 + c 2 + a c .
So q = a 2 + c 2 + a c which means q 2 + a c = a 2 + c 2 + a c + a c = ( a + c ) 2 = 1 0 2 = 1 0 0 .