Using all trigonometric functions

Geometry Level 5

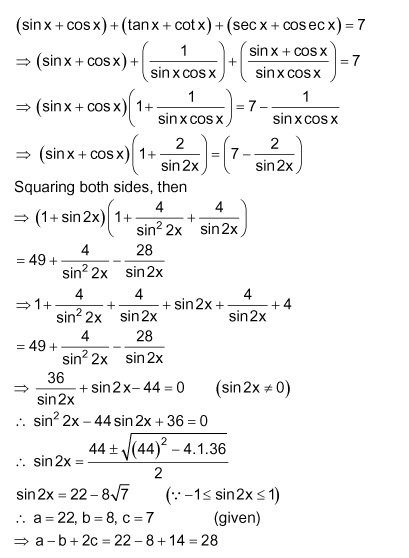
$\large \sin(x) + \cos(x) + \tan(x) + \csc(x) + \sec(x) + \cot(x) = 7$

Suppose a real number $x$ satisfy the equation above.

And for positive constants $a,b,c$ , we have $\sin(2x) = a - b \sqrt c$ with $c$ square-free. Find the value of $a-b+2c$ .

The answer is 28.

2 solutions

Tanishq Varshney
May 30, 2015

Moderator note:

Yes, we just need to group them such that we can apply the substitution $\sin(2A) = 2\sin(A) \cos(A)$ .

Bonus question: With the same given equation, can you write a least degree polynomial such that it has a root of $\tan\left(\frac x2 \right)$ ?

To make your work neater, you can factor it nicely as:

$7\sin(2x)-2=(\sin x+\cos x)(2+\sin(2x))$

Now, if we consider $A=\sin(2x)$ and square both sides of that equation we have, we get, after a bit of simplification,

$A^3-44A^2+36A=0\implies A=0,22\pm 8\sqrt7$

We can easily rule out the two extraneous solutions $A=0$ and $A=22+8\sqrt 7$ using the reason you provided. We get the answer as $A=22-8\sqrt 7$ .

Prasun Biswas - 6 years ago

I used the substitution $\sin(x) + \cos(x) = A$

So, $\sin(x)\cdot\cos(x) = \frac{A^2 - 1}{2}$

-> Find A

-> Evaluate $A^2 - 1$

Renjith Joshua - 6 years ago

Didn't think of solvable to sin 2 x which I suppose to realize as asked. Nevertheless, a general way is still workable. I think you made your own a solvable question. Nice to know this equation.

Lu Chee Ket - 5 years, 7 months ago

Lu Chee Ket
Nov 2, 2015

x = 1.07764686747605+

Sin 2 x = 0.83398951148327+ = 22 - 8 Sqrt (7)

22 - 8 + 2 (7) = 28

Using all trigonometric functions

The answer is 28.

2 solutions

Moderator note:

0 pending reports