Vector Field / Line Integral (Part 2)

Calculus Level 5

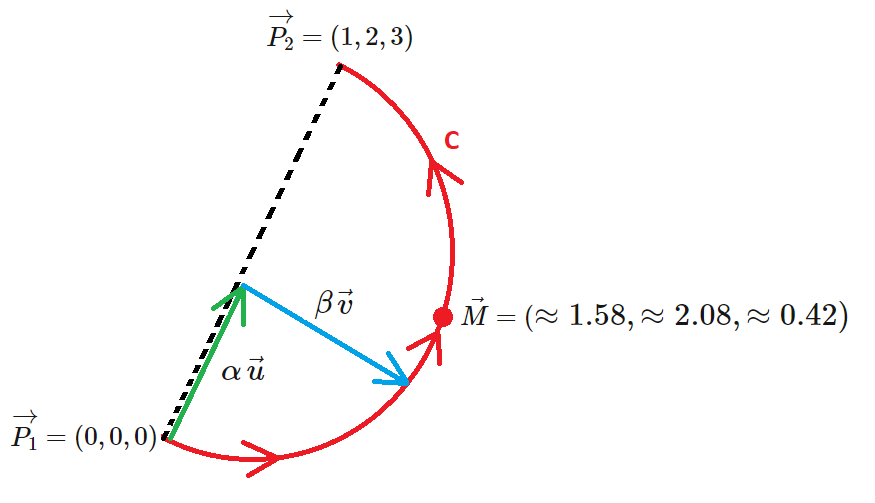
The vector field F ⃗ \vec{F} has components ( F x , F y , F z ) = ( x y , y z , z x ) (F_x,F_y,F_z) = (x y , y z , z x) . Consider the integration path C C , which is a semicircle starting at P 1 ⃗ = ( 0 , 0 , 0 ) \vec{P_1} = (0,0,0) and ending at P 2 ⃗ = ( 1 , 2 , 3 ) \vec{P_2} = (1,2,3) (see "details" section). The diameter of the semicircle is D D , and its radius is R R .

P ⃗ = P 1 ⃗ + α u ⃗ + β v ⃗ ( R − α ) 2 + β 2 = R 2 0 ≤ α ≤ D \large{\vec{P} = \vec{P_1} + \alpha \, \vec{u} + \beta \, \vec{v} \\ (R - \alpha)^2 + \beta^2 = R^2 \\ 0 \leq \alpha \leq D}

What is the value of the line integral of the vector field over the path?

∫ C F ⃗ ⋅ d ℓ ⃗ \large{\int_C \vec{F} \cdot \vec{d \ell}}

Details and Assumptions:
1) Vector u ⃗ \vec{u} is a unit-vector in the direction of ( 1 , 2 , 3 ) (1,2,3)
2) Vector v ⃗ \vec{v} is a unit-vector in the direction of ( 1 , 1 , − 1 ) (1,1,-1)
3) The middle point of the curve is M ⃗ = ( ≈ 1.58 , ≈ 2.08 , ≈ 0.42 ) \vec{M} = (\approx 1.58 ,\approx 2.08,\approx 0.42) . Use the positive root for β \beta


The answer is 4.202.

Steven Chase by Steven Chase , 37, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

The center of the semicircle is at 1 2 ( { 0 , 0 , 0 } + { 1 , 2 , 3 } ) \frac{1}{2} (\{0,0,0\}+\{1,2,3\}) or { 1 2 , 1 , 3 2 } \left\{\frac{1}{2},1,\frac{3}{2}\right\} .

The normalized u ⃗ \vec{u} vector from the center to the start is { − 1 14 , − 2 7 , − 3 14 } \left\{-\frac{1}{\sqrt{14}},-\sqrt{\frac{2}{7}},-\frac{3}{\sqrt{14}}\right\} .

The normalized v ⃗ \vec{v} vector is { 1 3 , 1 3 , − 1 3 } \left\{\frac{1}{\sqrt{3}},\frac{1}{\sqrt{3}},-\frac{1}{\sqrt{3}}\right\} .

p ⃗ ( θ ) \vec{p}(\theta ) is { 7 6 sin ⁡ ( θ ) − cos ⁡ ( θ ) 2 + 1 2 , 7 6 sin ⁡ ( θ ) − cos ⁡ ( θ ) + 1 , − 7 6 sin ⁡ ( θ ) − 1 2 3 cos ⁡ ( θ ) + 3 2 } \left\{\sqrt{\frac{7}{6}} \sin (\theta )-\frac{\cos (\theta )}{2}+\frac{1}{2},\sqrt{\frac{7}{6}} \sin (\theta )-\cos (\theta )+1,-\sqrt{\frac{7}{6}} \sin (\theta )-\frac{1}{2} 3 \cos (\theta )+\frac{3}{2}\right\} .

∂ p ( θ ) ∂ θ \frac{\partial p(\theta )}{\partial \theta } is { sin ⁡ ( θ ) 2 + 7 6 cos ⁡ ( θ ) , sin ⁡ ( θ ) + 7 6 cos ⁡ ( θ ) , 3 sin ⁡ ( θ ) 2 − 7 6 cos ⁡ ( θ ) } \left\{\frac{\sin (\theta )}{2}+\sqrt{\frac{7}{6}} \cos (\theta ),\sin (\theta )+\sqrt{\frac{7}{6}} \cos (\theta ),\frac{3 \sin (\theta )}{2}-\sqrt{\frac{7}{6}} \cos (\theta )\right\} .

f ( x , y , z ) f(x,y,z) is { x y , y z , x z } \{x y,y z,x z\} .

The expression to be integrated, f ⃗ ( p ⃗ ( θ ) ⋅ ∂ p ( θ ) ∂ θ \vec{f}(\vec{p}(\theta )\cdot\frac{\partial p(\theta )}{\partial \theta } expands and simplifies to 1 72 sin ⁡ 2 ( θ 2 ) ( − 34 42 cos ⁡ ( 2 θ ) + 58 42 cos ⁡ ( θ ) + 6 sin ⁡ ( θ ) ( 13 − 97 cos ⁡ ( θ ) ) + 32 42 ) \frac{1}{72} \sin ^2\left(\frac{\theta }{2}\right) \left(-34 \sqrt{42} \cos (2 \theta )+58 \sqrt{42} \cos (\theta )+6 \sin (\theta ) (13-97 \cos (\theta ))+32 \sqrt{42}\right) .

The indefinite integral is 1 864 ( 18 42 θ − 189 42 sin ⁡ ( 2 θ ) + 258 42 sin ⁡ ( θ ) + 34 42 sin ⁡ ( 3 θ ) − 1341 cos ⁡ ( θ ) + 990 cos ⁡ ( 2 θ ) − 291 cos ⁡ ( 3 θ ) ) \frac{1}{864} \left(18 \sqrt{42} \theta -189 \sqrt{42} \sin (2 \theta )+258 \sqrt{42} \sin (\theta )+34 \sqrt{42} \sin (3 \theta )-1341 \cos (\theta )+990 \cos (2 \theta )-291 \cos (3 \theta )\right) .

The result is 1 8 π 7 6 + 34 9 \frac{1}{8} \pi \sqrt{\frac{7}{6}}+\frac{34}{9} or about 4.20194126461.

0 Helpful 0 Interesting 1 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...