Vertical Circular Motion!
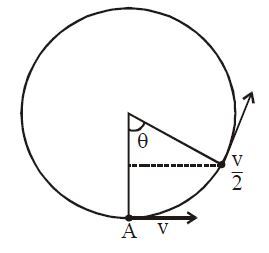 A bob of mass
is suspended by mass-less string of length
. The horizontal velocity at point
is such that the tension at point
is five times the weight of bob. The angle '
' at which the speed of bob is half of speed at point
is:
A bob of mass
is suspended by mass-less string of length
. The horizontal velocity at point
is such that the tension at point
is five times the weight of bob. The angle '
' at which the speed of bob is half of speed at point
is:
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let T be the tension in the string.
Given T = 5 M g
T − M g = M L v 2
v 2 = 4 g L ............................... ( 1 )
Applying conservation of energy
0 . 5 M v 2 = M g L ( 1 − cos θ ) + 0 . 5 M ( 2 v ) 2
1 − cos θ = 2 3
cos θ = − 0 . 5
θ = 1 2 0 o