Volume of Cube/Cone - Part 3
A cube of unit side length is placed on the x y plane with its base centered at the origin. In addition, a right circular cone is superimposed on the cube, such that its circular base lies flat on the x y plane as well, with a diameter of 2 , and centered at the origin as well, so that the circular edge of the cone base passes through the four vertices of the cube base. The height of the cone is 2 . Find the volume of the region in space that is inside the cube and inside the cone (the common region).
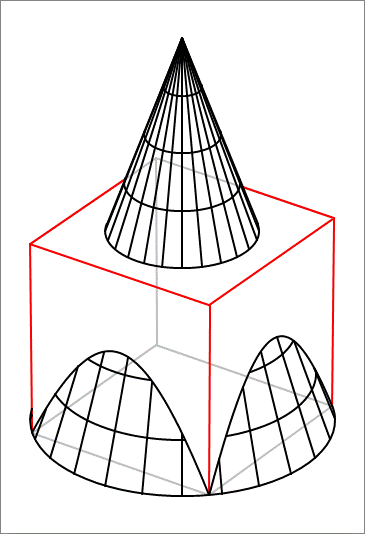
The answer is 0.787.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
In the first problem of this series , it was determined that the volume of the cone lying outside the cube is given by
V 1 = 2 4 9 π − 3 4 + 3 2 ln ( 2 + 1 )
It follows that the volume of the cone lying inside the cube is
V = 3 1 π ( 2 1 ) 2 ( 2 ) − V 1 = − 2 4 π + 3 4 − 3 2 ln ( 2 + 1 ) = 0 . 7 8 7
@Hosam Hajjir sir i have again uploaded the problem , with correct answer.
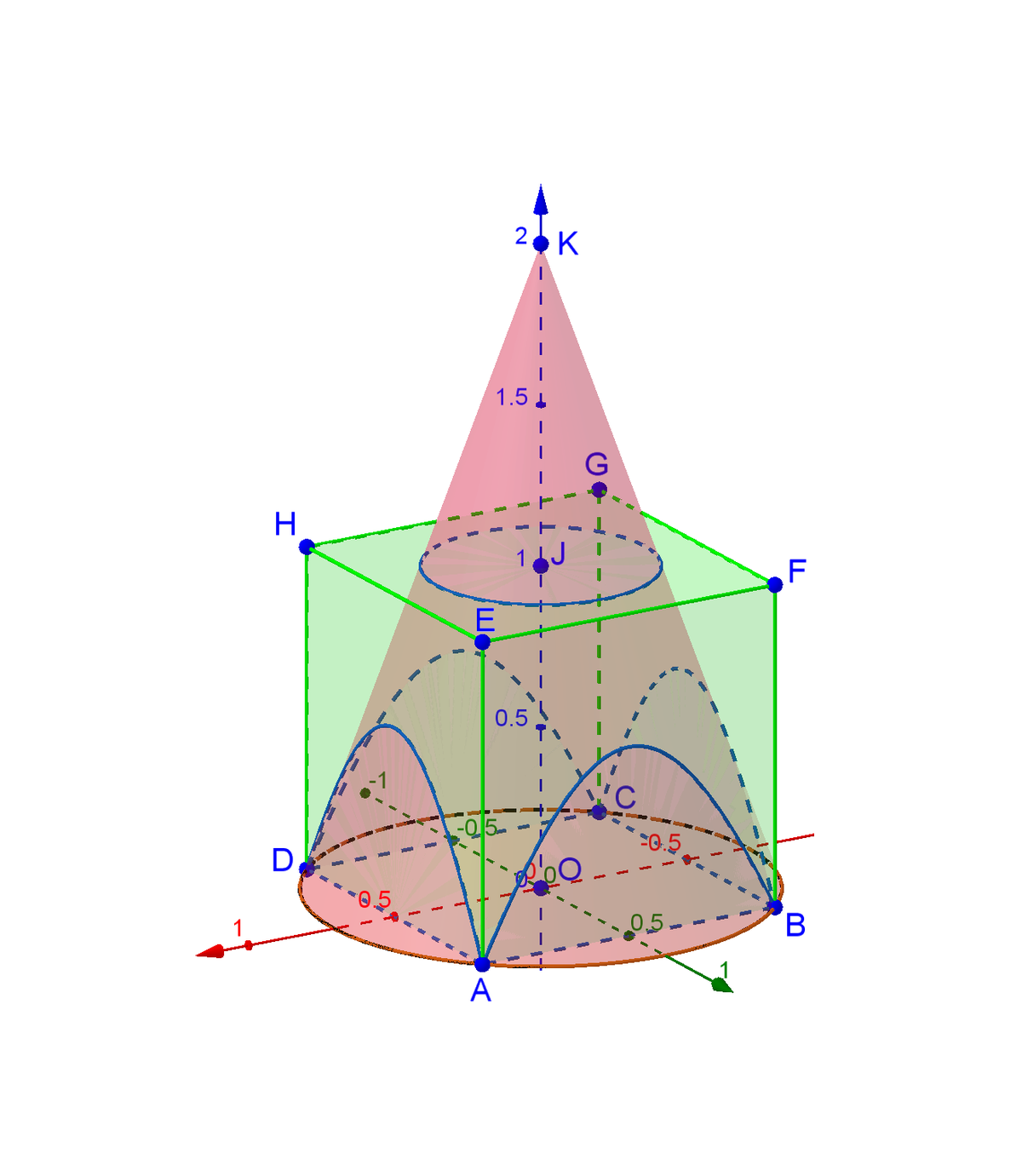 figure 1
The volume that is inside the cube and inside the cone (
figure 1
) can be calculated by the relation
V
=
V
t
r
u
n
c
a
t
e
d
c
o
n
e
−
4
⋅
V
c
o
n
e
s
e
g
m
e
n
t
By “cone segment” we mean the part of the cone that is outside the cube, bounded by the cord
A
D
, the hyperbolic arc and the arc of the cone’s circular base that corresponds to the cord
A
D
. We proceed in calculating the volume of this segment.
figure 1
The volume that is inside the cube and inside the cone (
figure 1
) can be calculated by the relation
V
=
V
t
r
u
n
c
a
t
e
d
c
o
n
e
−
4
⋅
V
c
o
n
e
s
e
g
m
e
n
t
By “cone segment” we mean the part of the cone that is outside the cube, bounded by the cord
A
D
, the hyperbolic arc and the arc of the cone’s circular base that corresponds to the cord
A
D
. We proceed in calculating the volume of this segment.
In the animated
figure 2
,
M
is the midpoint of
A
D
⌢
(assume
M
∈
x
′
x
) and
Z
S
W
is the hyperbolic intersection of a plane through a point
V
of
O
M
perpendicular to
O
M
. Denote
S
V
=
h
and
M
V
=
t
. The triangles
△
S
M
V
,
△
K
M
O
are similar right triangles, hence,
M
V
S
V
=
O
M
O
K
⇒
t
h
=
2
2
2
⇒
h
=
2
2
t
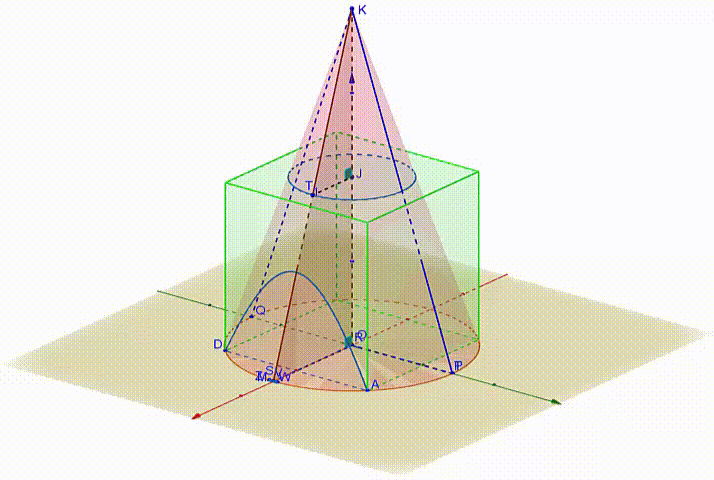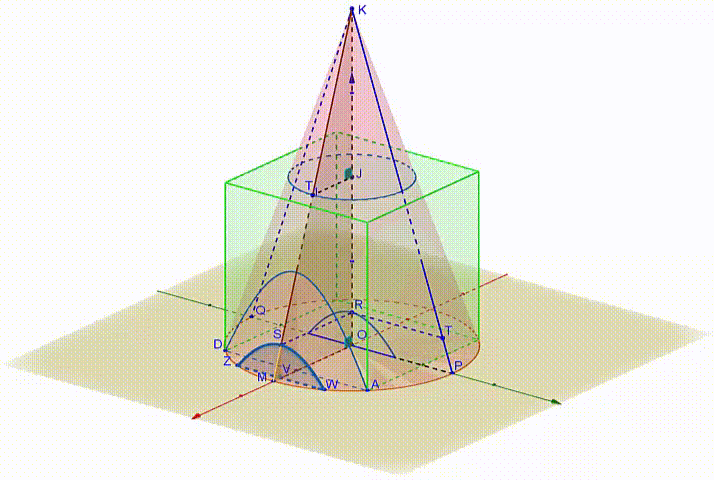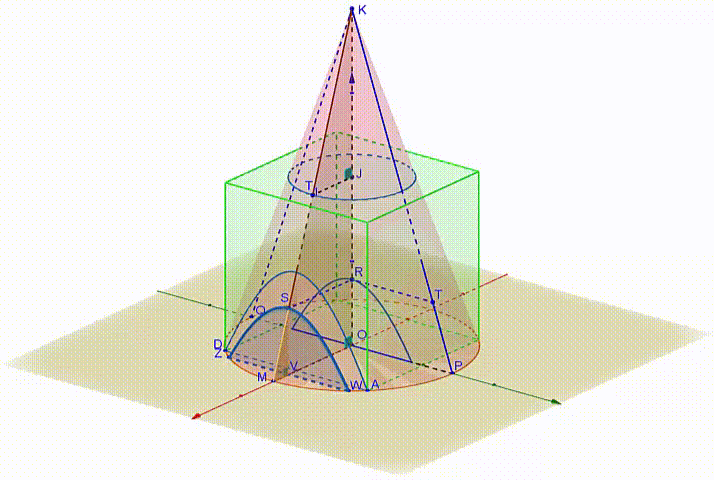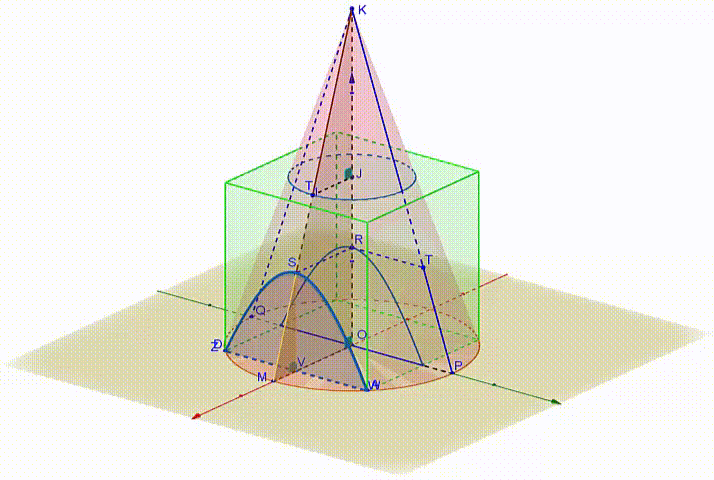 figure 2
figure 2
If we project the hyperbolic segment on the y z plane the vertex of the hyperbola is projected on point R and its asymptotes are the lines K P , K Q ( figures 2 and 3 ).

figure 3
The area enclosed by a hyperbola with equation a 2 x 2 − b 2 y 2 = 1 and the line l : x = k is given by the formula A = b ⎣ ⎡ k ( a k ) 2 − 1 − a ⋅ arcosh ( a k ) ⎦ ⎤ (for a proof see here )
On the y z plane, consider a 2D coordinate system with the origine at K and the line K O as the x-axis (positive direction the direction of K O ). In this setting, line l has equation x = 2 .
Moreover,
a
=
K
R
=
K
O
−
h
=
2
−
2
2
t
,
b
=
R
T
=
R
S
=
O
V
=
O
M
−
M
V
=
2
2
−
t
Substituting we get A = ( 2 2 − t ) ⎣ ⎡ 2 ( 2 − 2 2 t 2 ) 2 − 1 − ( 2 − 2 2 t ) ⋅ arcosh ( 2 − 2 2 t 2 ) ⎦ ⎤ Now, the volume of the cone segment is V c o n e s e g m e n t = 0 ∫ 2 2 − 1 A ⋅ d t = 0 ∫ 2 2 − 1 ( 2 2 − t ) ⎣ ⎡ 2 ( 2 − 2 2 t 2 ) 2 − 1 − ( 2 − 2 2 t ) ⋅ arcosh ( 2 − 2 2 t 2 ) ⎦ ⎤ ⋅ d t ≈ 0 . 0 3 2 3 3 6 9
Next, we go for the volume of the truncated cone.
The big cone with base on plane
x
y
and height
2
is similar to the small one with base
(
J
,
J
T
)
and height
1
.
Since the ratio of the heights is
K
O
K
J
=
2
1
, the ratio of their volumes is
8
1
.
Hence,
V
t
r
u
n
c
a
t
e
d
c
o
n
e
=
V
b
i
g
c
o
n
e
−
V
s
m
a
l
l
c
o
n
e
=
8
7
×
V
b
i
g
c
o
n
e
=
8
7
×
3
1
×
π
×
(
2
2
)
2
×
2
=
2
4
7
π
Finally,
V
=
V
t
r
u
n
c
a
t
e
d
c
o
n
e
−
4
⋅
V
c
o
n
e
s
e
g
m
e
n
t
=
2
4
7
π
+
4
×
0
.
0
3
2
3
3
6
9
≈
0
.
7
8
7
Wow, that is a really beautiful and cool solution. Thank you for the effort you put into it!
Log in to reply
Thanks! I'm glad you liked it. I'm just playing around having fun with Geogebra.
Log in to reply
Huh, I hadn't heard of that. What a neat tool, and a creative application of it!
The volume of the cone inside the cube ( V ) is equal to the volume of a truncated cone with a top circle of 2 2 in diameter and the bottom circle base of 2 in diameter ( V t ) subtracts four of the solid shaded green in the figure ( V s ) . That is V = V t − 4 V s .
To find V s we consider A ( z ) is the cross-sectional area of the solid at z . We note that the radius of the circular arc is given by 2 − z r = 2 2 2 ⟹ r = 8 2 − z . And A ( z ) = 2 θ r 2 − 2 r 2 sin θ , where θ = 2 cos − 1 2 r 1 . Then we have:
V s = ∫ 0 2 − 2 2 r 2 ( θ − sin θ ) d z = 2 ∫ 2 1 2 1 r 2 ( θ − sin θ ) d r = 2 1 ∫ 2 1 2 1 ( 4 r 2 cos − 1 2 r 1 − 4 r 2 − 1 ) d r ≈ 0 . 0 3 2 3 3 7 Since 8 r = 2 − z ⟹ 8 d r = − d z
Therefore V = V t − 4 V s = 3 π ( 2 × ( 2 1 ) 2 − ( 2 2 1 ) 2 ) − 4 × 0 . 0 3 2 3 3 7 ≈ 0 . 7 8 7 .