Volume of My Valentine 2
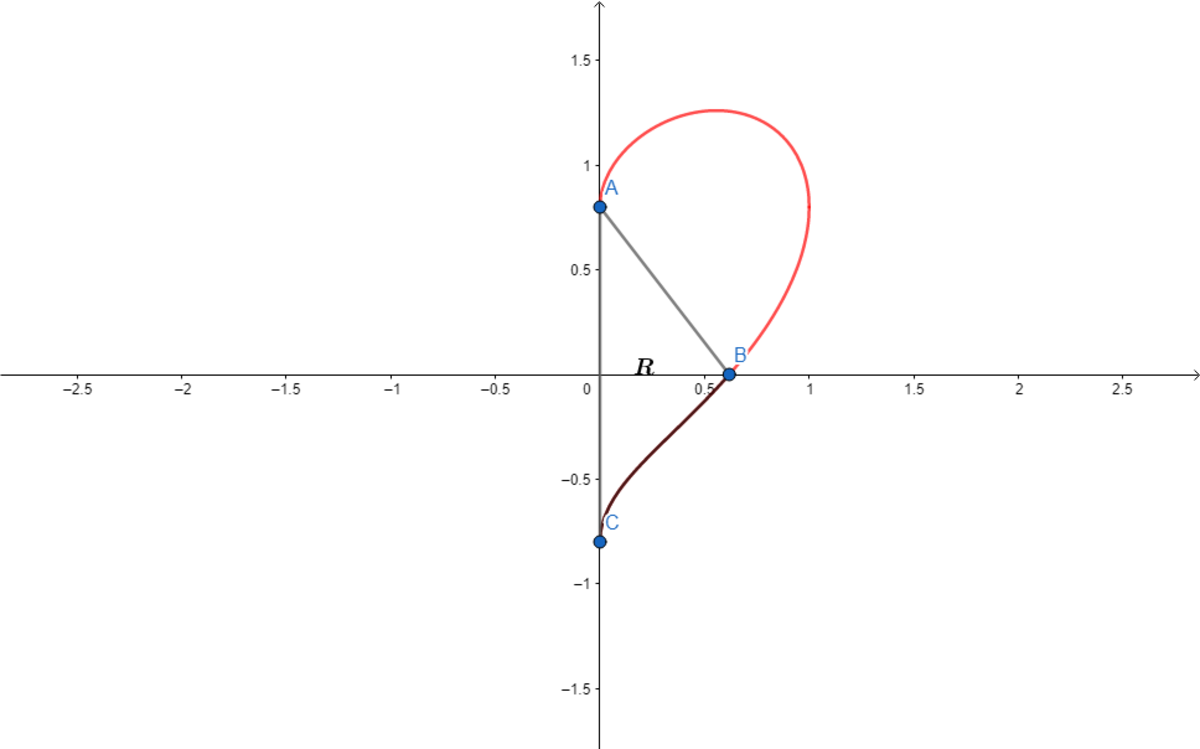
In the half heart curve x 2 + ( 4 5 y − x ) 2 = 1 above, A B goes from the positive y intercept to the positive x intercept and points A , B , C encloses the region R .
If the region R of the half heart curve x 2 + ( 4 5 y − x ) 2 = 1 is revolved about the y axis the resulting volume is V R = b a 3 π ( c 1 − c 1 ( ϕ − 1 ) a c + a ∗ c 1 ( ϕ − 1 ) a − b a ( ϕ − 1 ) a b ) .
Find a + b + c , where a , b and c are coprime positive integers and ϕ is the golden ratio.
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
For the x intercept of y 1 = 5 4 ( x − 1 − x 2 ) we obtain:
x 2 + x − 1 = 0 ⟹ x = 2 − 1 ± 5 , since x = − 2 1 + 5 = − ϕ results in a complex valued square root ⟹ x = 2 5 − 1 is the x intercept of y 1 = 5 4 ( x − 1 − x 2 ) .
The equation of the line passing thru A : ( 0 , 5 4 ) and B : ( 2 5 − 1 , 0 ) is:
y = 5 4 ( 5 − 1 − 2 x + 1 ) ⟹
V R = 2 π ( 5 4 ) ∫ 0 2 5 − 1 x ( ( 1 − 5 − 1 2 x + 1 − x 2 − x ) ) d x = 5 8 π ∫ 0 2 5 − 1 ( x − 5 − 1 2 x 2 + x 1 − x 2 − x x ) d x
For ∫ x 1 − x 2 d x
Let x = sin ( θ ) ⟹ d x = cos ( θ ) ⟹ ∫ x 1 − x 2 d x = − ∫ ( cos ( θ ) ) 2 ( − sin ( θ ) ) d θ = − 3 1 ( cos ( θ ) ) 3 .
∴ ∫ 0 2 5 − 1 x 1 − x 2 d x = 3 1 ( 1 − ( 2 5 − 1 ) 2 3 ) ⟹
V R = 5 8 π ( 3 1 − 3 1 ( 2 5 − 1 ) 2 3 + ( 2 x 2 − 3 ( 5 − 1 ) 2 x 3 − 5 2 x 2 3 ) ∣ 0 2 5 − 1
Let β = 2 5 − 1 = 2 5 + 1 − 1 = ϕ − 1 ⟹
V R = 5 8 π ( 3 1 − 3 1 ( ϕ − 1 ) 2 3 + 6 1 ( ϕ − 1 ) 2 − 5 2 ( ϕ − 1 ) 2 5 ) = 5 2 3 π ( 3 1 − 3 1 ( ϕ − 1 ) 2 3 + 2 ∗ 3 1 ( ϕ − 1 ) 2 − 5 2 ( ϕ − 1 ) 2 5 ) = b a 3 π ( c 1 − c 1 ( ϕ − 1 ) a c + a ∗ c 1 ( ϕ − 1 ) a − b a ( ϕ − 1 ) a b )
⟹ a + b + c = 1 0 .