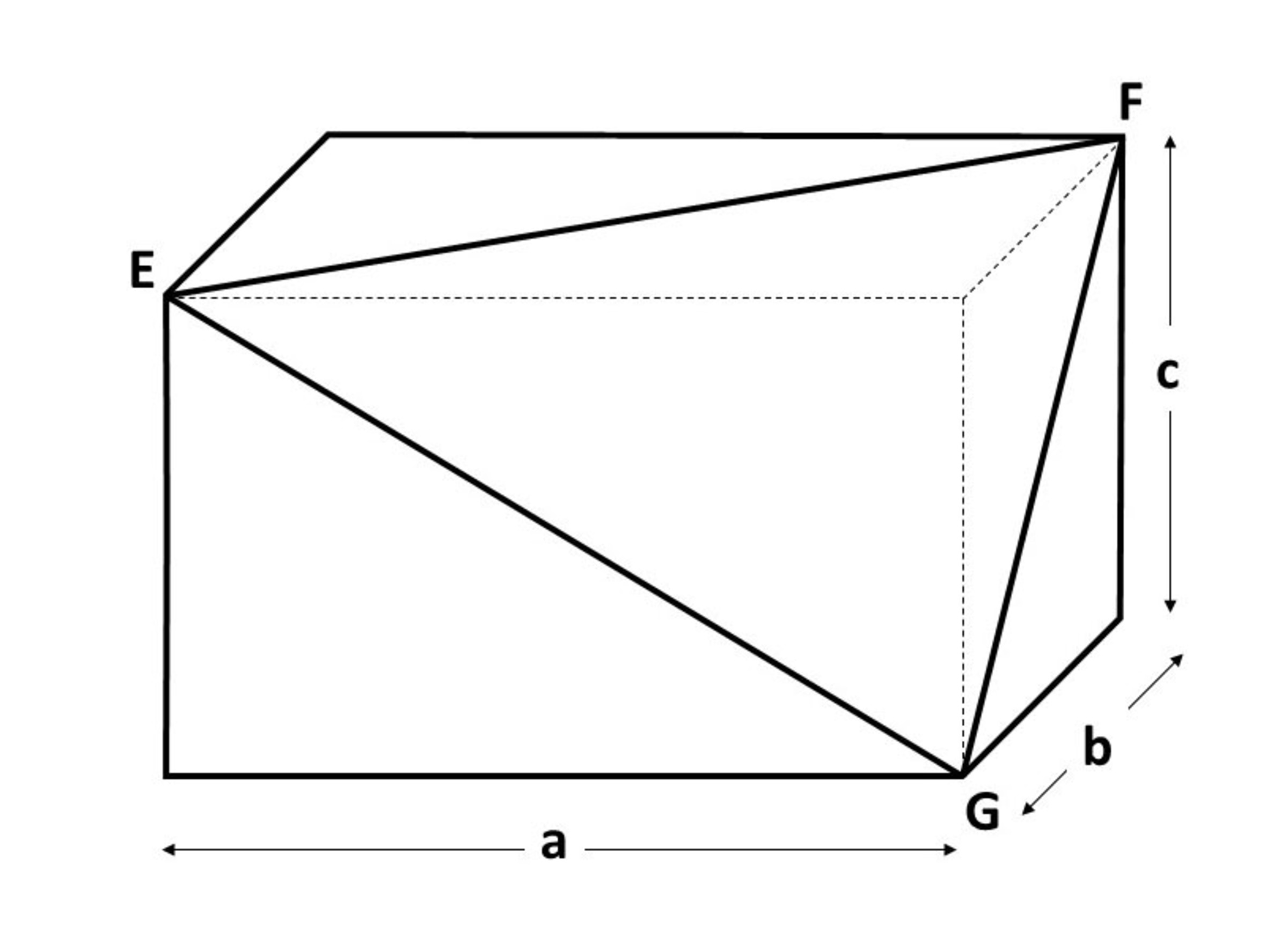
Volume of what remains of the cuboid

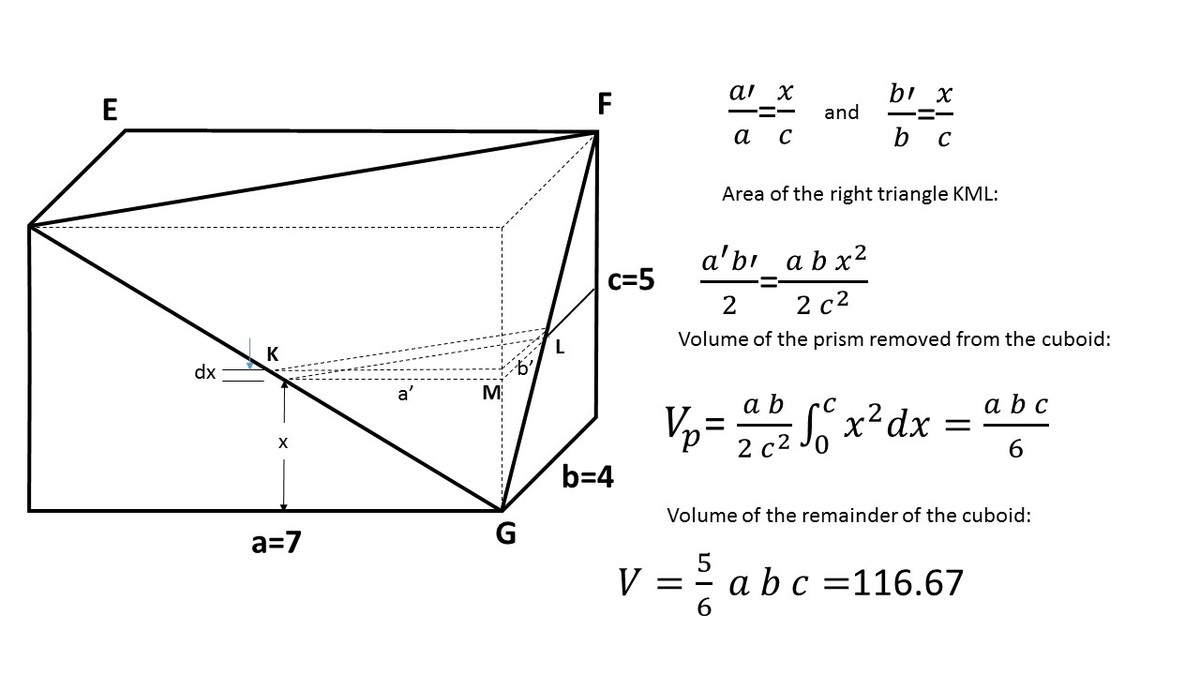
Let the vertex of the pyramid be $H$ . The volume of the pyramid $EFGH$ is given by:

$\begin{aligned} V_{\text{pyramid}} & = \frac 13 \times \text{ base area } \times \text{ height} \\ & = \frac 13 \times [EFH] \times \overline{HG} \\ & = \frac 13 \times \frac {ab}2 \times c \\ & = \frac {abc}6 \end{aligned}$

Then the volume of what remains is given by:

$\begin{aligned} V_{\text{remains}} & = V_{\text{cuboid}} - V_{\text{pyramid}} \\ & = abc - \frac {abc}6 \\ & = \frac 56 abc \\ & \approx \boxed{116.67} \end{aligned}$