Water and Electricity
A 120V source is connected across the plates of a parallel-plate capacitor. The capacitor is being submerged vertically at constant speed into a container filled with water. What current in Amps flows through the voltage source during this process if it takes τ = 2 0 s to totally submerge the capacitor? The capacitance (in air) of the capacitor is C 0 = 1 2 μ F and water's relative permittivity is ϵ = 8 1 .
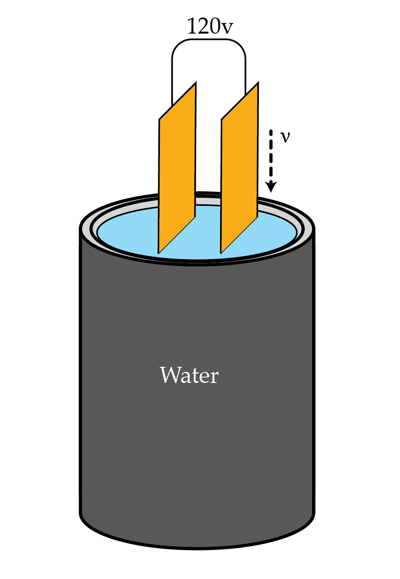
Hint: If the capacitor is partially submerged at an instant of time try figuring out how to treat it as two separate capacitors at that instant.
The answer is 0.00576.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Discussions for this problem are now closed
The wording was kind misleading in a way. The question should have specified that the average current was asked. I infered it from the fact that it was asking for the current for a finite time interval.
The charge stored initially on the capacitor is C 0 V , and charge stored on it finally is ϵ C 0 V . So net charge, flown through the battery is Q = ( ϵ − 1 ) C 0 V . Thus average current is τ Q = τ ( ϵ − 1 ) C 0 V
In this case, because it's being submerged at a constant speed, the average current is equal to the instantaneous current (dA/dt is constant). But otherwise, yeah you're right.
I = t Δ Q ⇒ I = t C f V − C i V ⇒ I = t 8 1 C i V − C i V ⇒ I = t 8 0 C i V ⇒ I = 2 0 8 0 × 1 2 × 1 0 − 6 × 1 2 0 ⇒ I = 0 . 0 0 6
Comment if you have problem understanding any step.
I think, practical imperfections are too may in this procedure ,uncertainty will be very high due to
-
action of sharp point with water ,
2.charges are accelerated due various dipoles
-
bond breaking due accelerated plates effect ....
4.frenzies flied effect (direction of electric field will not be constant between the plates )
and it will go on and on.
we can take 2 or 3 assumptions it is acceptable but this problems make me take many assumption ,can u make me clear with the assumption please . thank u
Well said! This is the case with many problems in Physics which is kind of very frustrating sometimes.
We know that I = d t d Q = d t d ( C V ) = V d t d C + C d t d V Since V = 1 2 0 V is constant, the current can simply be expressed as I = 1 2 0 × d t d C Thus, we need to find an expression for the total capacitance C as a function of time.
It is important to realise that since one part of the capacitor is immersed in water while the other part is exposed to air, the two parts can be treated as two different capacitors. Furthermore, by examining how the voltage source is connected to these two capacitors closely, we would realise that the two capacitors are connected in parallel series.
Next, let the capacitance of the capacitor exposed to air and the capacitor exposed to water be C 1 and C 2 respectively. Then, C = C 1 + C 2 Now, let the distance between the capacitor plates, width, total height, and height of part of the capacitor immersed in water be D , x , L , and y respectively. C 1 and C 2 can then be expressed as C 1 = ϵ 0 D x ( L − y )
C 2 = ϵ ϵ 0 D x y
However, since C 0 can be expressed as C 0 = ϵ 0 D x L
We can rewrite the expressions for C 1 and C 2 as C 1 = ( 1 − L y ) C 0
C 2 = ( L y ϵ ) C 0
Meanwhile, we also realise that L y can be expressed in terms of time, as follows: L y = v τ v t = 2 0 t
Therefore, we can rewrite the expressions for C 1 and C 2 again in terms of time. Adding the two expressions would give the following expression of C in terms of t : C = [ 1 + 2 0 t ( ϵ − 1 ) ] C 0
In conclusion, the value of the current can be obtained as follows: I = 1 2 0 × d t d C = 1 2 0 × ( 2 0 ϵ − 1 ) C 0 = 5 . 7 6 × 1 0 − 3 A