Circuit, circuit, and circuit
Three resistors , , and are arranged into three circuits of different total resistance as shown below:
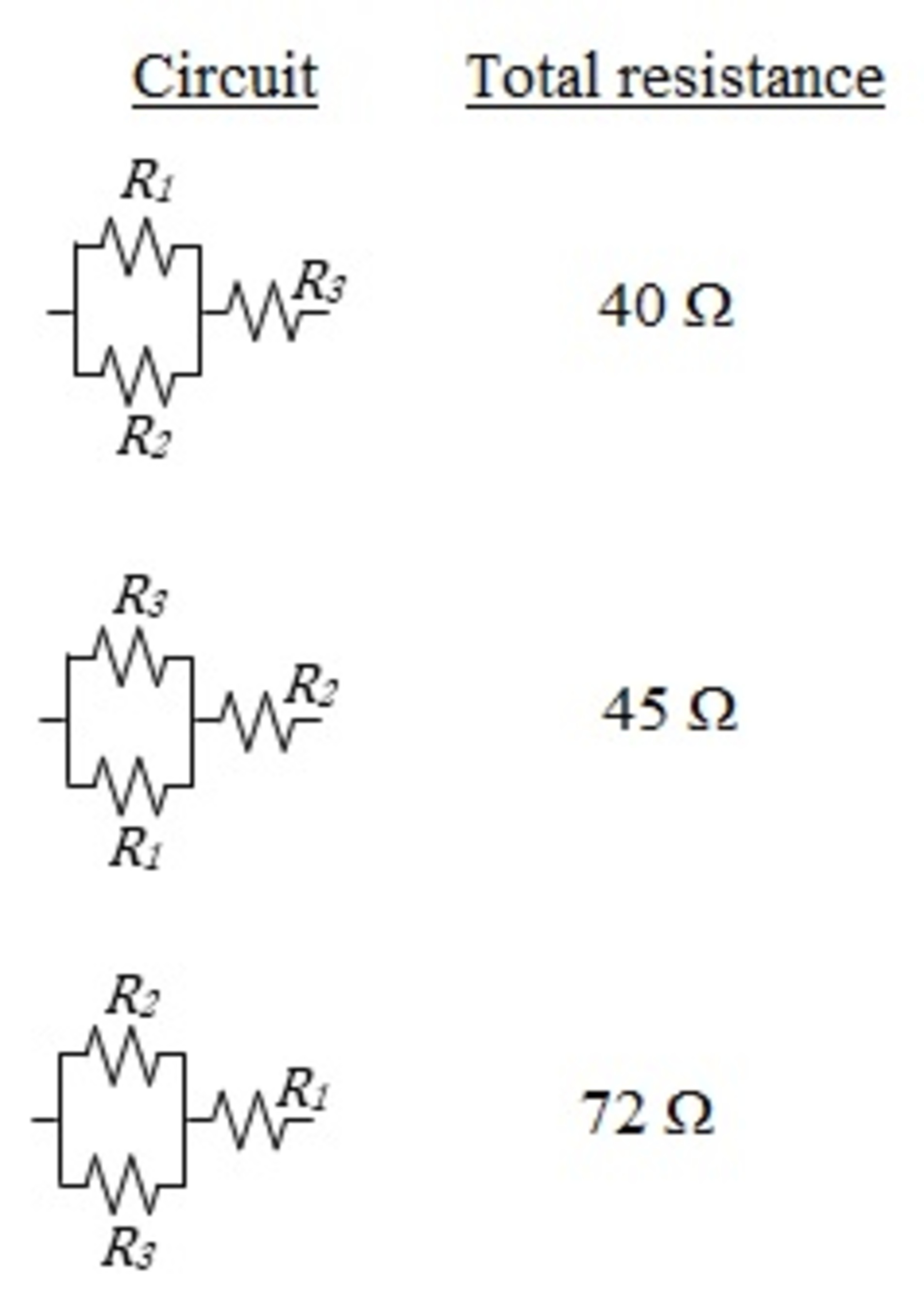
Find the difference between the highest resistance and the lowest resistance among the three resistors.
The answer is 40.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
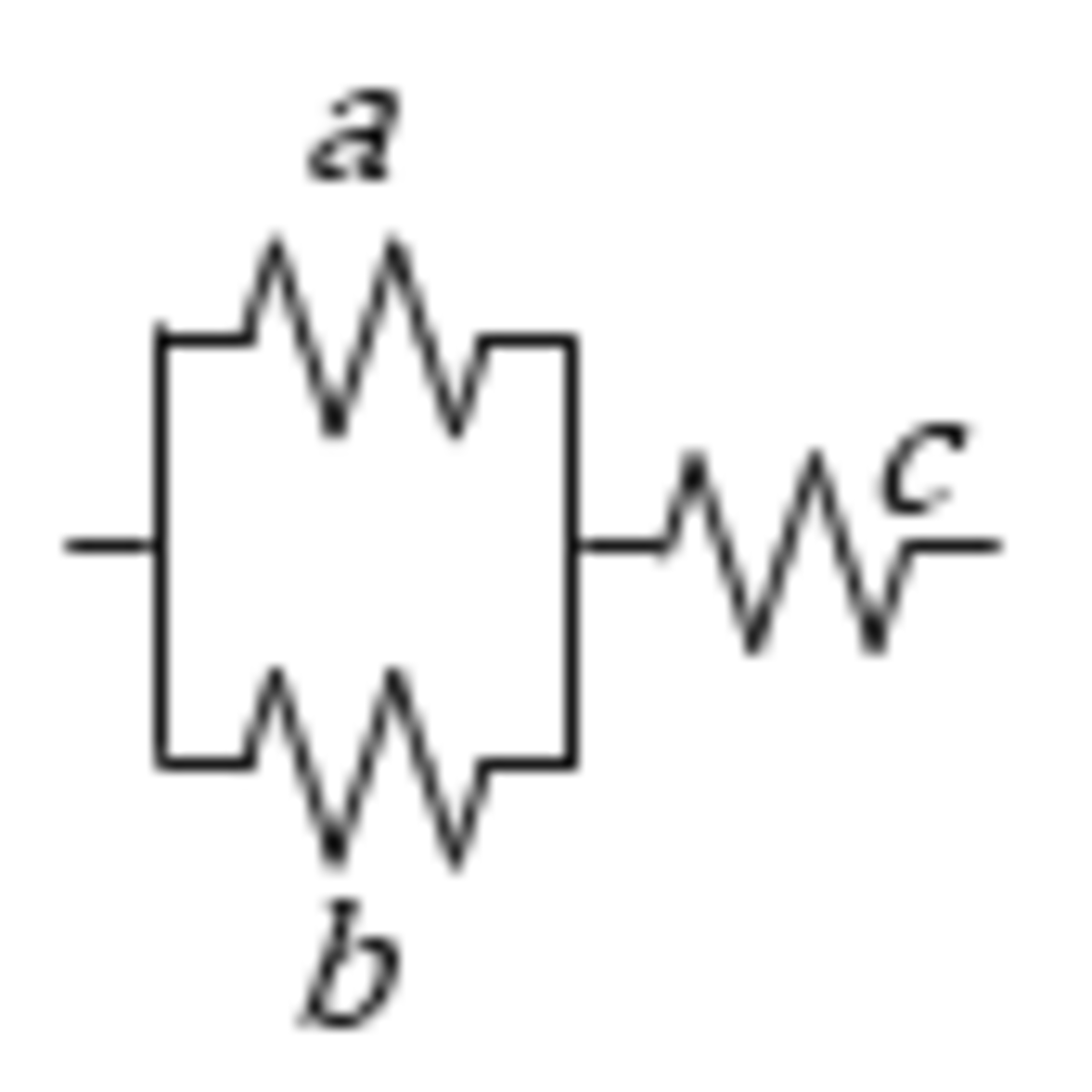
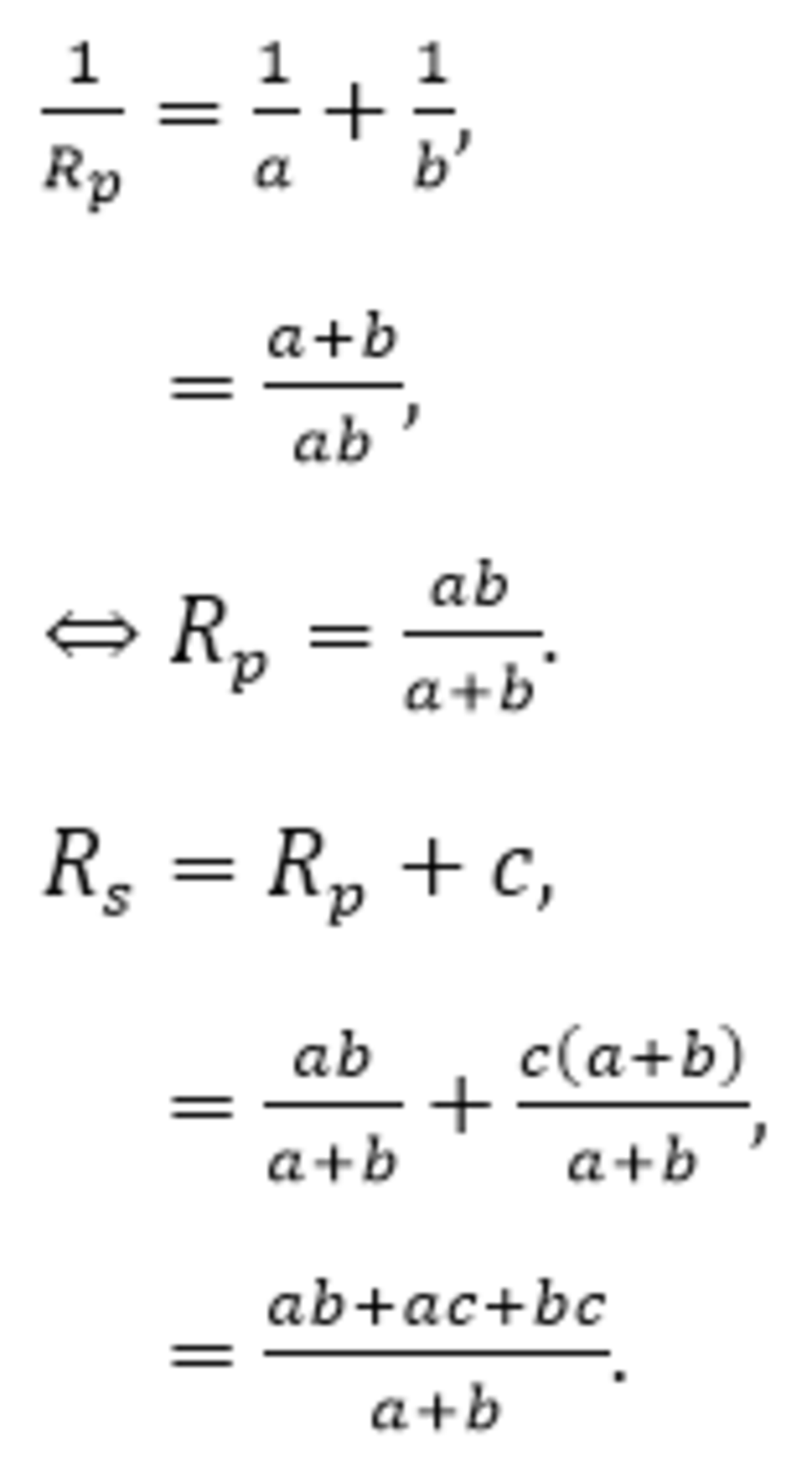
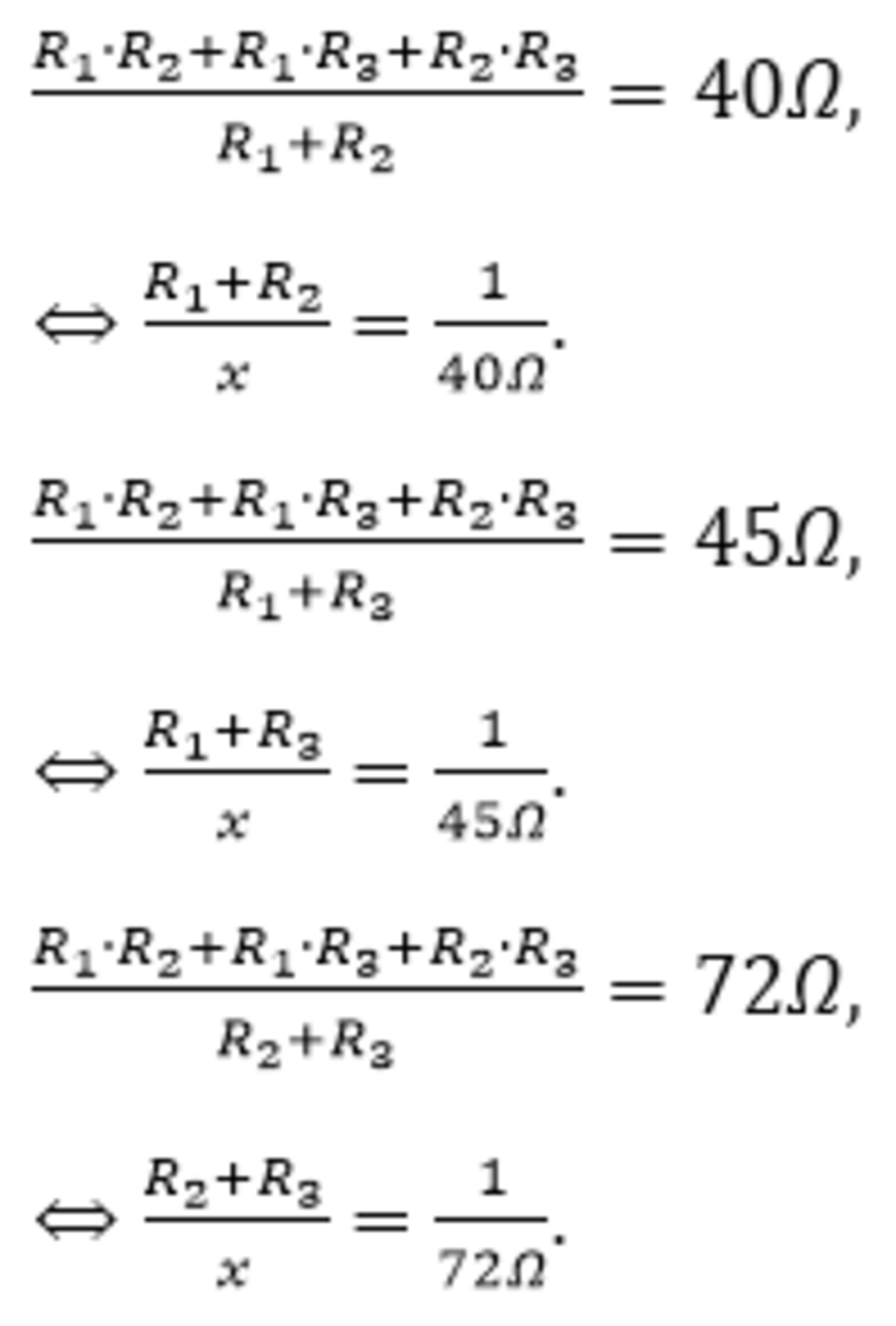
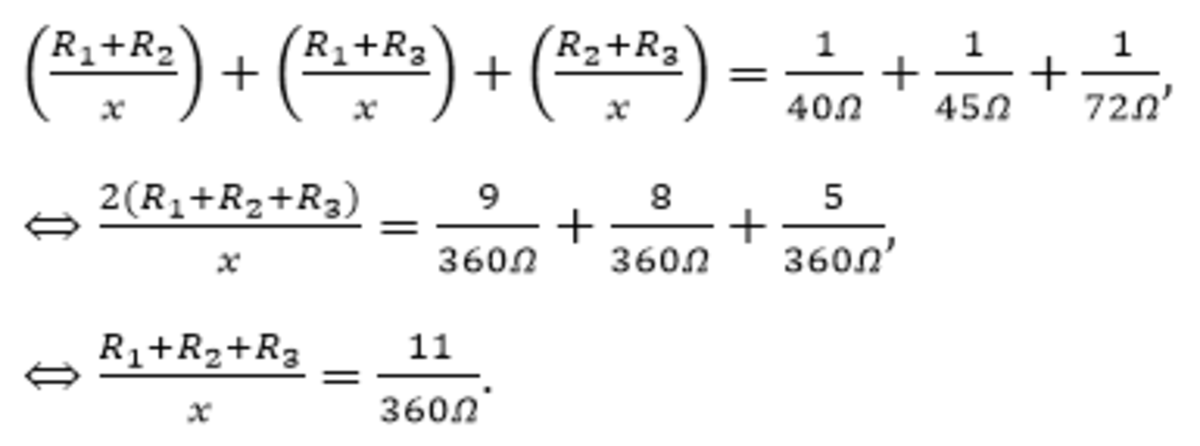
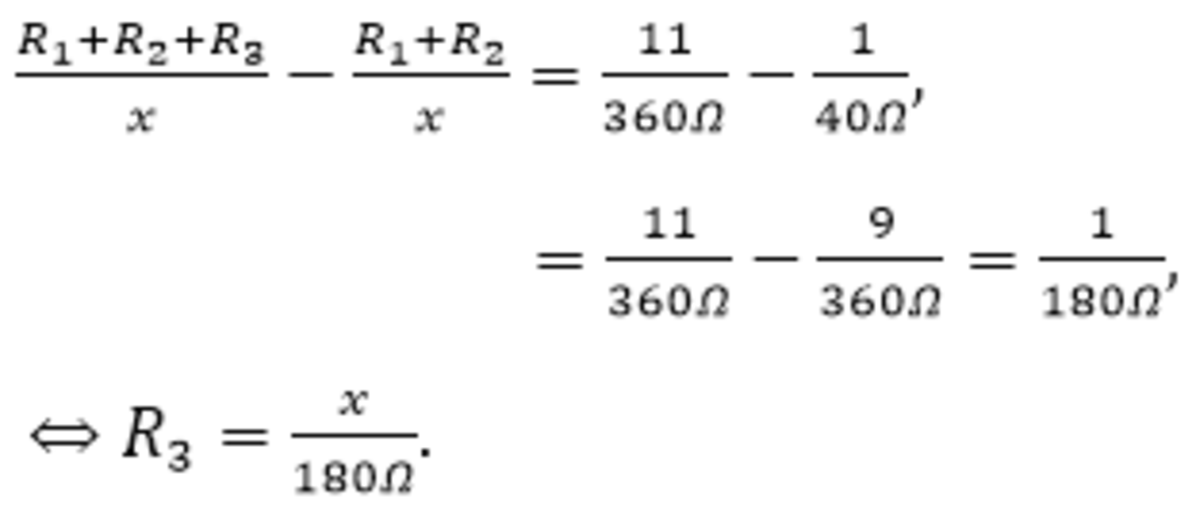


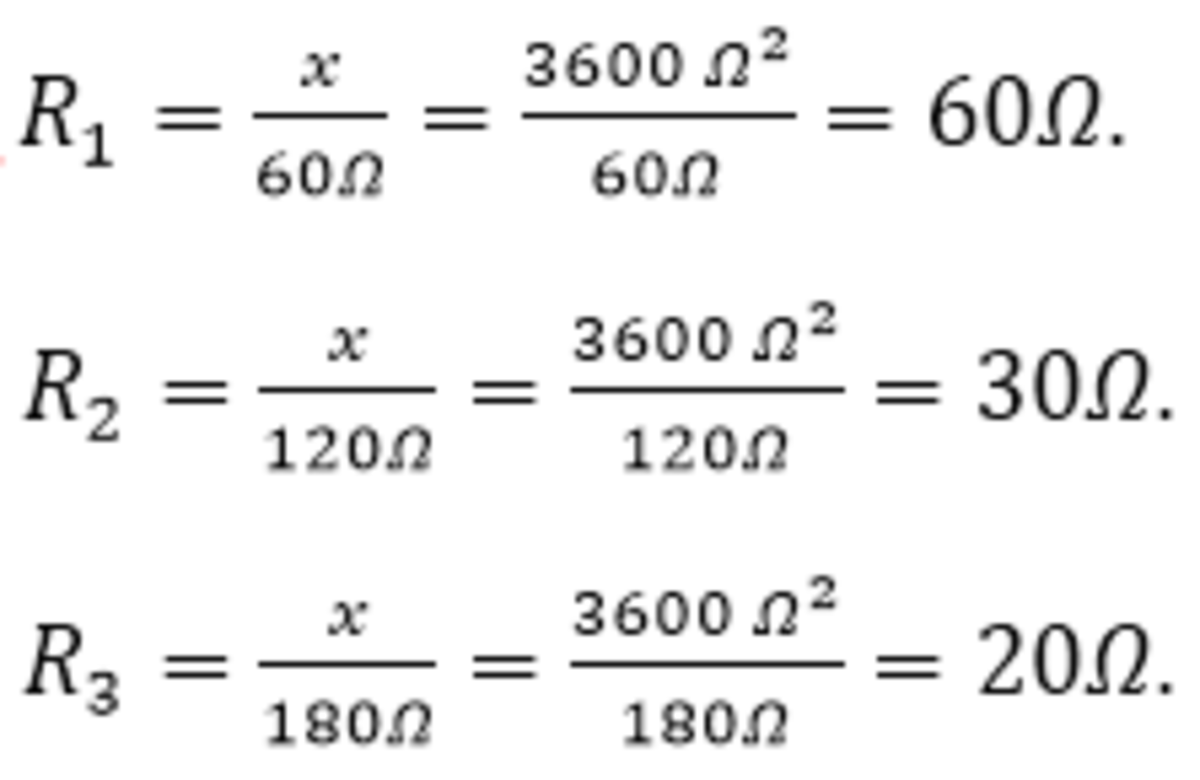
From the three circuits, we have:
⎩ ⎪ ⎪ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎪ ⎪ ⎧ R 1 ∣ ∣ R 2 + R 3 = R 1 + R 2 R 1 R 2 + R 3 = 4 0 R 3 ∣ ∣ R 1 + R 2 = R 3 + R 1 R 3 R 1 + R 2 = 4 5 R 2 ∣ ∣ R 3 + R 1 = R 2 + R 3 R 2 R 3 + R 1 = 7 2 ⟹ R 1 R 2 + R 2 R 3 + R 3 R 1 = 4 0 ( R 1 + R 2 ) ⟹ R 1 R 2 + R 2 R 3 + R 3 R 1 = 4 5 ( R 3 + R 1 ) ⟹ R 1 R 2 + R 2 R 3 + R 3 R 1 = 7 2 ( R 2 + R 3 ) . . . ( 1 ) . . . ( 2 ) . . . ( 3 )
⟹ 4 0 ( R 1 + R 2 ) = 4 5 ( R 3 + R 1 ) = 7 2 ( R 2 + R 3 )
{ ( 1 ) = ( 2 ) : ( 2 ) = ( 3 ) : R 1 = 8 R 2 − 9 R 3 5 R 1 = 8 R 2 + 3 R 3 . . . ( 4 ) . . . ( 5 )
( 4 ) + 3 ( 5 ) : 1 6 R 1 = 3 2 R 2 ⟹ R 1 = 2 R 2
( 4 ) : 2 R 2 = 8 R 2 − 9 R 3 ⟹ R 3 = 3 2 R 2
( 1 ) : R 1 + R 2 R 1 R 2 + R 3 3 R 2 2 R 2 2 + R 3 3 2 R 2 + R 3 R 3 + R 3 ⟹ R 3 R 2 R 1 = 4 0 = 4 0 = 4 0 = 4 0 = 2 0 Ω = 2 3 R 3 = 3 0 Ω = 2 R 2 = 6 0 Ω Note that R 1 = 2 R 2 Note that R 3 = 3 2 R 2
Therefore, the difference between the highest and lowest resistance among the three resistors: R 1 − R 3 = 6 0 − 2 0 = 4 0 .