Wedged in equilateral triangles.
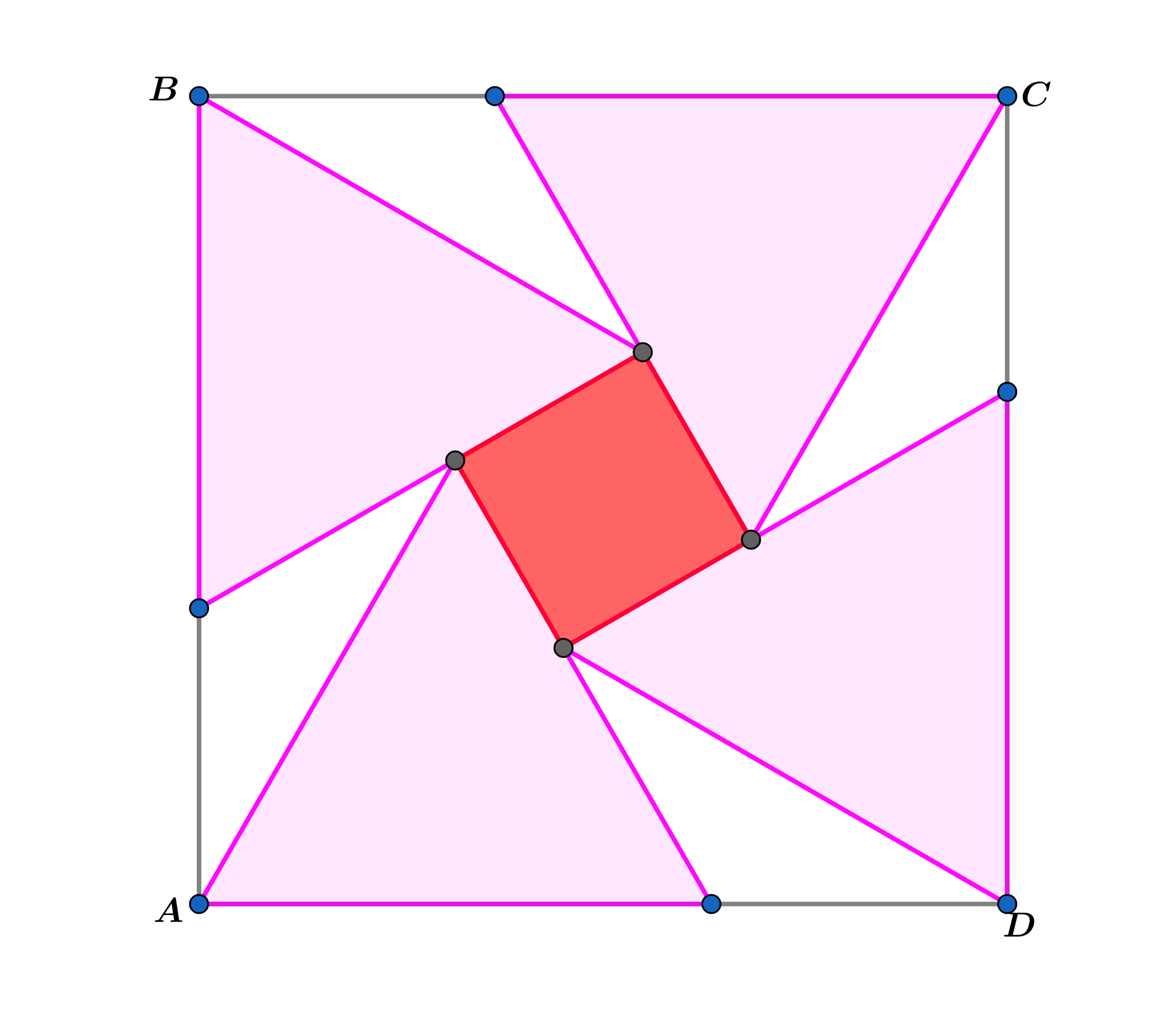
In square , four congruent equilateral triangles are wedged in to form the inner red square.
Let be the area of the red square.
If , where and are coprime positive integers, find .
The answer is 14.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let m be a side of square A B C D .
Let A e be the area of equilateral △ D F E ⟹ 4 A e = 4 ( 2 1 ) ( a ) ( 2 3 a ) = 3 a 2
Let A I be the area of △ G F D
The height h △ G F D = ( m − a ) sin ( 6 0 ∘ ) = 2 3 ( m − a ) ⟹
4 A I = 4 ( 2 1 ) ( m − a ) 2 ( 2 3 ) = 3 ( m − a ) 2
Using the law of cosines on △ G F D with included ∠ F G D ⟹
a 2 = 2 ( m − a ) 2 ( 2 3 ) = 3 ( m − a ) 2 = 3 a 2 − 6 m a + 3 m 2 ⟹ 2 a 2 − 6 m a + 3 m 2 = 0
⟹ a = 2 3 ± 3 m
a = 2 3 + 3 m ⟹ m − a = − ( 1 + 3 ) m < 0
a = 2 3 − 3 m ⟹ m − a = 2 3 − 1 m
∴ we drop a = 2 3 + 3 m and choose a = 2 3 − 3 m
⟹ 4 A e = 4 3 ( 3 − 3 ) 2 m 2 = 2 3 3 ( 2 − 3 ) m 2
and
4 A I = 3 ( m − a ) 2 = 4 3 ( 3 − 1 ) 2 m 2 = 2 3 ( 2 − 3 ) m 2
⟹ A s = m 2 − ( 4 A e + 4 A I ) = ( 1 − ( 2 3 ( 2 − 3 ) ) ) m 2 = ( 1 − ( 4 3 − 6 ) ) m 2
= ( 7 − 4 3 ) m 2
⟹ A △ A B C D A s = ( 7 − 4 3 ) = α − β λ ⟹ α + β + λ = 1 4 .