What a triangle is that?

Geometry Level 4

Given a triangle A B C ABC with ∡ A C B = 100 \measuredangle ACB=100 and ∡ B A C = 30 \measuredangle BAC=30 , point M M lies on the side A C AC ,such that C M = C B CM=CB . If the ratio B M : A C = p : q BM:AC=p:q , where p p and q q are co-prime positive integers, compute p + q p+q .


The answer is 2.

View wiki
Kristian Vasilev by Kristian Vasilev , 24, Bulgaria

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

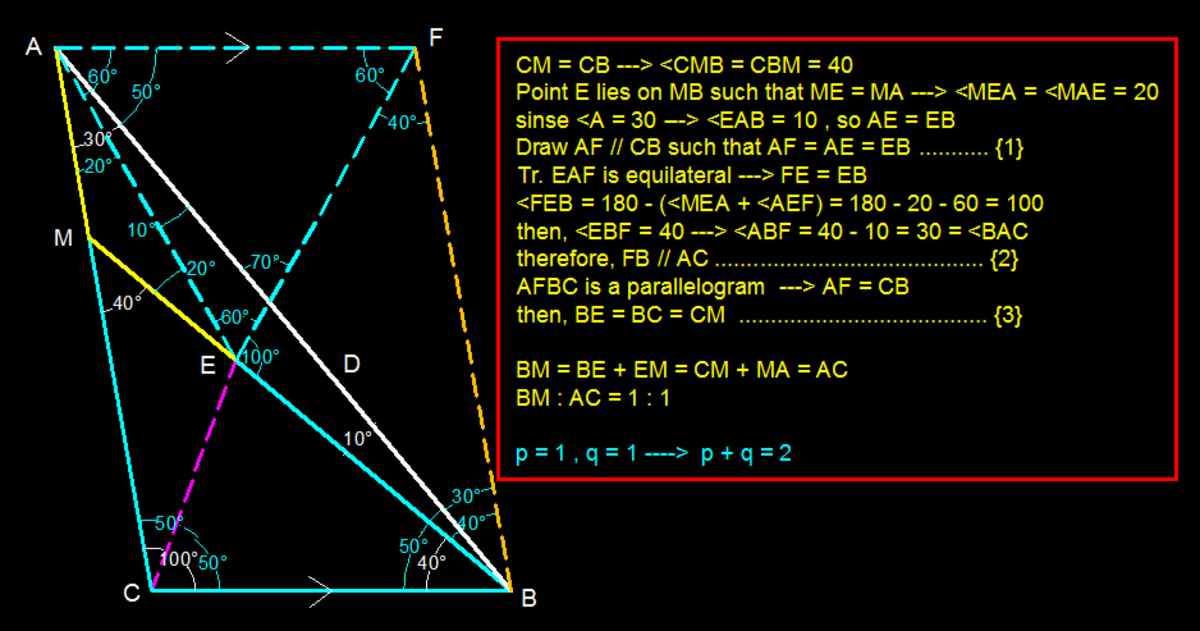
Let C M = C B = a CM=CB=a , then by Sine Rule, we have:

q sin ⁡ 5 0 ∘ = a sin ⁡ 3 0 ∘ ⇒ q = 2 a sin ⁡ 5 0 ∘ \quad \dfrac {q}{\sin{50^\circ}} = \dfrac {a}{\sin{30^\circ}}\quad \Rightarrow q = 2a\sin{50^\circ}

By Cosine Rule:

p 2 = a 2 + a 2 − 2 a 2 cos ⁡ 10 0 ∘ = 2 a 2 ( 1 − cos ⁡ 10 0 ∘ ) \quad p^2 = a^2+a^2-2a^2\cos{100^\circ}= 2a^2(1-\cos{100^\circ})

= 2 a 2 ( 1 − ( 1 − 2 sin ⁡ 2 5 0 ∘ ) = 4 a 2 sin ⁡ 2 5 0 ∘ = q 2 \quad \quad = 2a^2( 1 - (1-2\sin^2 {50^\circ}) = 4a^2\sin^2 {50^\circ} = q^2

⇒ q = p ⇒ q : p = 1 : 1 ⇒ q + p = 2 \quad \Rightarrow q=p \quad \Rightarrow q:p = 1:1 \quad \Rightarrow q+p = \boxed {2}

4 Helpful 0 Interesting 0 Brilliant 0 Confused

nice solution, but please try without trigonometry

Kristian Vasilev - 6 years, 6 months ago

Log in to reply

Ur free to do that :)

Chirayu Bhardwaj - 5 years, 3 months ago
Ahmad Saad
Nov 3, 2015

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...