What a weird position!

Logic Level 3

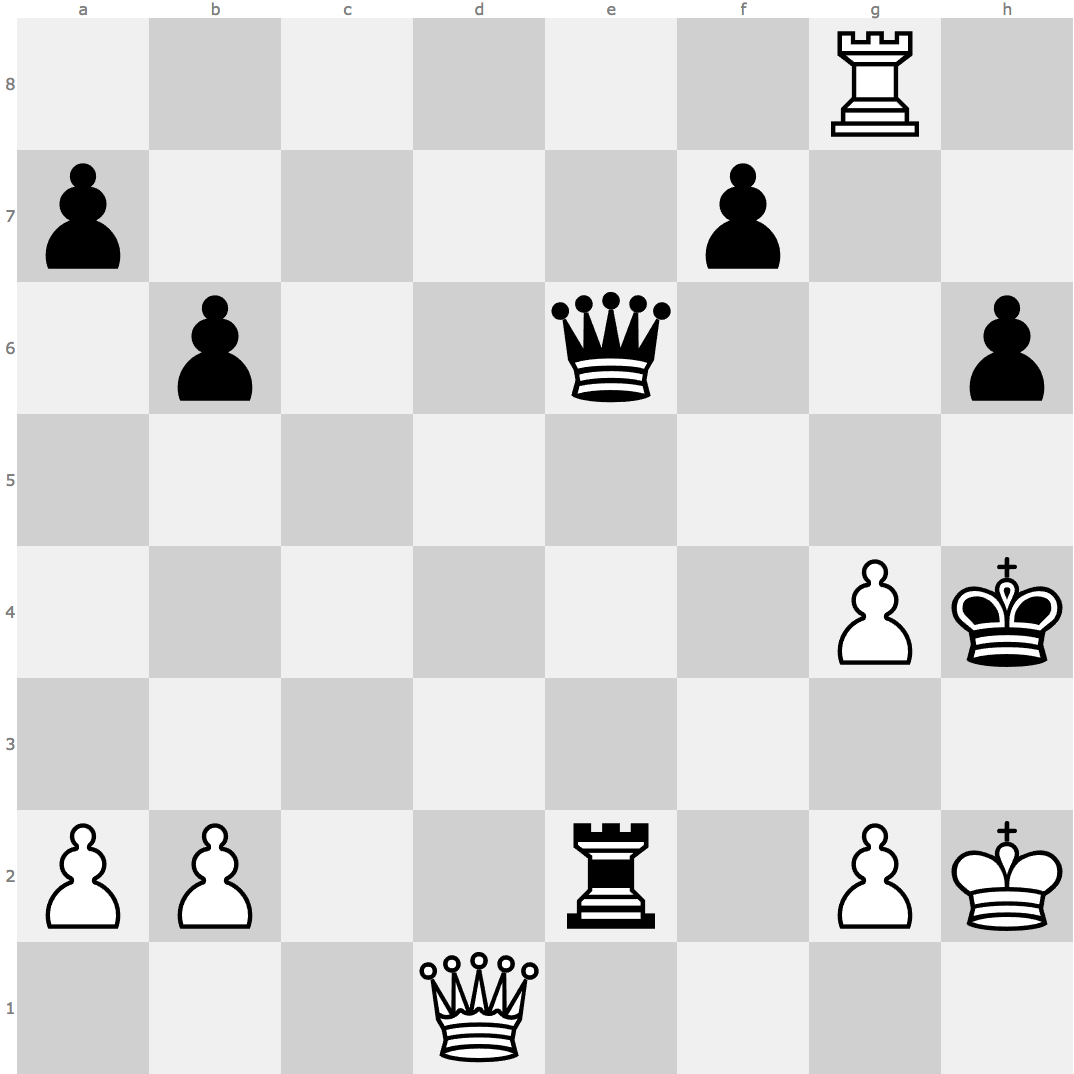
It is currently white to move. In how many moves will white successfully checkmate black, assuming both play optimally?

Mate in 1 Mate in 2 Mate in 3 Mate in 4

View wiki
Sharky Kesa by Sharky Kesa , 19, United Kingdom

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Sharky Kesa
Feb 18, 2017

  1. Qe1+! Rxe1

  2. g3#

This position was in Stahlberg vs Becker 1944.

Alternatively, Black can move their rook to f2 to get

  1. ... Rf2

  2. Qxf2#

4 Helpful 0 Interesting 0 Brilliant 0 Confused

Its ironic -To sacrifice your queen to mate with a pawn :)

Anirudh Sreekumar - 4 years, 3 months ago
Michael Huang
Feb 19, 2017

Since the checkmate is not unique, there is the position different from Sharky's moves:

White Black
1. Qe1+ Rf2
2. Qxf2
1 Helpful 0 Interesting 0 Brilliant 0 Confused

Why would the rook go to f2?

Though, it's hard to say what optimal play is, given that checkmate happens in the next turn regardless of what the rook does?

Calvin Lin Staff - 4 years, 3 months ago

Log in to reply

I asked that question myself too, but I figured out that this is possible.

What the rook does is to prevent the king from being checked. Even though this wouldn't be the best optimal move, this extends the number of moves made.

If suppose that the rook is not moved to f2, then this returns to Sharky's solution, where the rook captures the queen. The final checkmate is g3, showing that the pawn (on the king's zone) checkmates the black king.

The main issue for the black is that:

Since the Black king runs out of options for protection or safety as shown in the starting point, the best move is Qe1+, which reduces the White's problem to two possible cases - either the white queen is captured, or the rook is moved to f2. Thus, either case leads to Mate in 2.

I hope this helps! I appreciate your thoughts about my solution. :)

Michael Huang - 4 years, 3 months ago

Log in to reply

Yea. For such puzzles, the solution should be along the lines of

White moves.
Black could counter in all of these ways: A, B, C....
Scenario A: White does this. (Black could counter with AA, AB, AC, ......)
Scenario B: White does this. (Black could counter with BA, BB, BC, ...)


We should use a "clean single sequence" if and only if it's clear that every possible Black counter is uniquely determined.

Calvin Lin Staff - 4 years, 3 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...